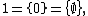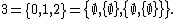“Set-theoretic definition of natural numbers”的意思、由来-开放百科全书

词条

Set-theoretic definition of natural numbers

释义

Definition as von Neumann ordinals
Definition by Frege and Russell
See also
References
External links

Several ways have been proposed to construct the natural numbers using set theory. These include the representation via von Neumann ordinals, commonly employed in axiomatic set theory, and a system based on equinumerosity that was proposed by Frege and by Russell.

Definition as von Neumann ordinals

In Zermelo–Fraenkel (ZF) set theory, the natural numbers are defined recursively by letting {{nowrap|1=0 = {} }} be the empty set and {{nowrap|1=n + 1 = n ∪ {n} }} for each n. In this way n = {0, 1, ..., n − 1} for each natural number n. The first few numbers defined this way are:

The set N of natural numbers is defined in this system as the smallest set containing 0 and closed under the successor function S defined by S(n) = n ∪ {n}. The structure ⟨N,0,S⟩ is a model of the Peano axioms. The existence of the set N follows from the axiom of infinity in ZF set theory.

The set N and its elements, when constructed this way, are an initial part of the von Neumann ordinals.

Definition by Frege and Russell

Gottlob Frege and Bertrand Russell each proposed defining a natural number n as the collection of all sets with n elements. More formally, a natural number is an equivalence class of finite sets under the equivalence relation of equinumerosity. This definition may appear circular, but it is not, because equinumerosity can be defined in alternate ways, such as by Hume's principle.{{Clarification needed|reason=Alternative not given -- equinumerosity is defined in terms of Hume's Principle. Non-circularity not obvious.|date=December 2017}}

This definition works in naive set theory, type theory, and in set theories that grew out of type theory, such as New Foundations and related systems. But it does not work in the axiomatic set theory ZFC and related systems, because in such systems the equivalence classes under equinumerosity are proper classes rather than sets.

William S. Hatcher (1982) derives Peano's axioms from several foundational systems, including ZFC and category theory, and from the system of Frege's Grundgesetze der Arithmetik using modern notation and natural deduction. The Russell paradox proved this system inconsistent, but George Boolos (1998) and David J. Anderson and Edward Zalta (2004) show how to repair it.

References

Anderson, D. J., and Edward Zalta, 2004, "Frege, Boolos, and Logical Objects," Journal of Philosophical Logic 33: 1–26.
George Boolos, 1998. Logic, Logic, and Logic.
Hatcher, William S., 1982. The Logical Foundations of Mathematics. Pergamon. In this text, S refers to the Peano axioms.
Holmes, Randall, 1998. Elementary Set Theory with a Universal Set. Academia-Bruylant. The publisher has graciously consented to permit diffusion of this introduction to NFU via the web. Copyright is reserved.
Patrick Suppes, 1972 (1960). Axiomatic Set Theory. Dover.

External links

Stanford Encyclopedia of Philosophy:
- Quine's New Foundations — by Thomas Forster.
- Alternative axiomatic set theories — by Randall Holmes.
McGuire, Gary, "[https://web.archive.org/web//http://www.maths.may.ie/staff/gmg/nn.ps What are the Natural Numbers?]"
Randall Holmes: New Foundations Home Page.

1 : Basic concepts in infinite set theory

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。

Definition as von Neumann ordinals

Definition by Frege and Russell

See also

References

External links