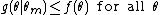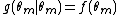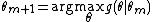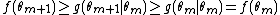- History
- Algorithm
- Constructing the surrogate function
- References
The MM algorithm is an iterative optimization method which exploits the convexity of a function in order to find their maxima or minima. The MM stands for “Majorize-Minimization” or “Minorize-Maximization”, depending on whether the desired optimization is a maximization or a minimization. MM itself is not an algorithm, but a description of how to construct an optimization algorithm.
The expectation–maximization algorithm can be treated as a special case of the MM algorithm.[1][2]
However, in the EM algorithm conditional expectations are usually involved, while in the MM algorithm convexity and inequalities are the main focus, and it is easier to understand and apply in most cases.
History
The historical basis for the MM algorithm can be dated back to at least 1970, when Ortega and Rheinboldt were performing studies related to line search methods.[3] The same concept continued to reappear in different areas in different forms. In 2000, Hunter and Lange put forth "MM" as a general framework.[4] Recent studies{{who?|date=September 2018}} have applied the method in a wide range of subject areas, such as mathematics, statistics, machine learning and engineering.{{cn|date=September 2018}}
Algorithm
The MM algorithm works by finding a surrogate function that minorizes or majorizes the objective function. Optimizing the surrogate function will drive the objective function upward or downward until a local optimum is reached.
Taking the minorize-maximization version, let  be the objective concave function to be maximized. At the {{mvar|m}} step of the algorithm,
be the objective concave function to be maximized. At the {{mvar|m}} step of the algorithm, 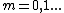 , the constructed function
, the constructed function 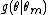 will be called the minorized version of the objective function (the surrogate function) at
will be called the minorized version of the objective function (the surrogate function) at  if
if
Then, maximize 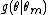 instead of
instead of  , and let
, and let
The above iterative method will guarantee that 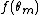 will converge to a local optimum or a saddle point as {{mvar|m}} goes to infinity.[5] By the above construction
will converge to a local optimum or a saddle point as {{mvar|m}} goes to infinity.[5] By the above construction
The marching of  and the surrogate functions relative to the objective function is shown in the figure.
and the surrogate functions relative to the objective function is shown in the figure.
Majorize-Minimization is the same procedure but with a convex objective to be minimised.
Constructing the surrogate function
One can use any inequality to construct the desired majorized/minorized version of the objective function. Typical choices include
- Jensen's inequality
- Convexity inequality
- Cauchy–Schwarz inequality
- Inequality of arithmetic and geometric means
- Quadratic majorization/mininorization via second order Taylor expansion of twice-differentiable functions with bounded curvature.
References
2. ^Kenneth Lange: "MM Optimization Algorithms", SIAM, {{ISBN|978-1-611974-39-3}} (2016).
3. ^{{cite book |last1=Ortega |first1=J.M. |last2=Rheinboldt|first2=W.C. |title=Iterative Solutions of Nonlinear Equations in Several Variables |location=New York |publisher=Academic |year=1970 |pages=253–255 |url=https://books.google.com/books?id=GA1P9UNnrmMC&pg=PA253|isbn=9780898719468 }}
4. ^{{cite journal |last1=Hunter|first1=D.R. |last2=Lange|first2=K. |title=Quantile Regression via an MM Algorithm |journal=Journal of Computational and Graphical Statistics |year=2000 |volume=9 |issue=1 |pages=60–77 |doi=10.2307/1390613|jstor=1390613 |citeseerx=10.1.1.206.1351 }}
5. ^{{cite journal |last=Wu |first=C. F. Jeff |year=1983 |title=On the Convergence Properties of the EM Algorithm |journal=Annals of Statistics |volume=11 |issue=1 |pages=95–103 |doi= 10.1214/aos/1176346060|jstor=2240463 }}
- Iłownica, Pomeranian Voivodeship
- Iłownica, Silesian Voivodeship
- Iłowo
- Iłowo (disambiguation)
- Iłowo-Osada
- Iłowo-Osada Commune
- Iłowo, Radziejów County
- Iłowo, Sępólno County
- Iłowo, West Pomeranian Voivodeship
- Iłowo-Wieś
- Iły
- Iły, Kuyavian-Pomeranian Voivodeship
- Iły, Masovian Voivodeship
- Iłów
- Iłów Commune
- Iłówiec
- Iłówiec (disambiguation)
- Iłówiec, Greater Poland Voivodeship
- Iłówiec, Masovian Voivodeship
- Iłówiec Wielki
- Iłówko
- Iłża Commune
- Iński
- Iński Landscape Park
- Iński Park Krajobrazowy