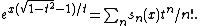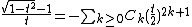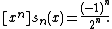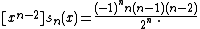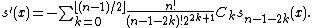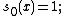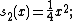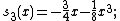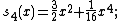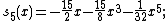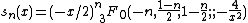- References
In mathematics the Mott polynomials sn(x) are polynomials introduced by {{harvs|txt|authorlink=Nevill Francis Mott|first=N. F. |last=Mott|year=1932|loc=p. 442}} who applied them to a problem in the theory of electrons.
They are given by the exponential generating function
Because the factor in the exponential has the power series
in terms of Catalan numbers 
, the coefficient in front of 
of the polynomial can be written as
,
according to the general formula for generalized Appell polynomials,
where the sum is over all compositions 
of 
into 
positive odd integers. The empty product appearing for 
equals 1. Special values, where all contributing Catalan numbers equal 1, are
By differentiation the recurrence for the first derivative becomes
The first few of them are {{OEIS|A137378}}
The polynomials sn(x) form the associated Sheffer sequence for –2t/(1–t2) {{harv|Roman|1984|loc=p.130}}.
{{harvs|txt | last1=Erdélyi | first1=Arthur | last2=Magnus | first2=Wilhelm | author2-link=Wilhelm Magnus | last3=Oberhettinger | first3=Fritz | last4=Tricomi | first4=Francesco G. | title=Higher transcendental functions. Vol. III | publisher=McGraw-Hill Book Company, Inc., New York-Toronto-London | mr=0066496 | year=1955|loc=p. 251|url=https://authors.library.caltech.edu/43491/}} give an explicit expression for them in terms of the generalized hypergeometric function 3F0:
References
- {{Citation | last1=Erdélyi | first1=Arthur | last2=Magnus | first2=Wilhelm | author2-link=Wilhelm Magnus | last3=Oberhettinger | first3=Fritz | last4=Tricomi | first4=Francesco G. | title=Higher transcendental functions. Vol. III | publisher=McGraw-Hill Book Company, Inc., New York-Toronto-London | mr=0066496 | year=1955}}
- {{Citation | last1=Mott | first1=N. F. | title=The Polarisation of Electrons by Double Scattering | jstor=95868 | year=1932 | journal=Proceedings of the Royal Society of London. Series A, Containing Papers of a Mathematical and Physical Character | issn=0950-1207 | volume=135 | issue=827 | pages=429–458 | doi=10.1098/rspa.1932.0044}}
- {{Citation | last1=Roman | first1=Steven | title=The umbral calculus | url=https://books.google.com/books?id=JpHjkhFLfpgC | publisher=Academic Press Inc. [Harcourt Brace Jovanovich Publishers] | location=London | series=Pure and Applied Mathematics | isbn=978-0-12-594380-2 | mr=741185 |id=Reprinted by Dover, 2005 | year=1984 | volume=111}}
- Edward Forster (botanist)
- Edward Forster (clergyman)
- Edward Forster the Younger
- Edward Forster the younger
- Edward Fort
- Edward Francis (bishop)
- Edward Francis Cavanagh, Jr.
- Edward Francis Cleary
- Edward Francis Fahy
- Edward Francis (footballer, born 1999)
- Edward Francis Paschke
- Edward Francis Rook
- Edward Francis Tedesco
- Edward Francis Trevenen Brown
- Edward Francis Tuke
- Edward Francis Wilson
- Edward Frank Gillett
- Edward Franklin
- Edward Franklin Bowers
- Edward Franklin Castetter
- Edward Frank Wise
- Edward Frederick Anderson
- Edward Frederick (British Army officer)
- Edward Frederick Grey Ponsonby
- Edward Frederick (Indian Army officer)