“Ogden–Roxburgh model”的意思、由来-开放百科全书

词条

Ogden–Roxburgh model

释义

References

The Ogden–Roxburgh model^[1] is an approach which extends hyperelastic material models to allow for the Mullins effect. It is used in several commercial finite element codes, and is named for R.W. Ogden and D. G. Roxburgh.

The basis of pseudo-elastic material models is a hyperelastic second Piola–Kirchhoff stress , which is derived from a suitable strain energy density function :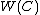

The key idea of pseudo-elastic material models is that the stress during the first loading process is equal to the basic stress . Upon unloading and reloading is multiplied by a positive softening function . The function thereby depends on the strain energy 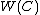of the current load and its maximum 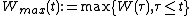in the history of the material:

It was shown that this idea can also be used to extend arbitrary inelastic material models for softening effects.^[2]

References

1. ^{{cite journal|last=Ogden|first=R. W|author2=Roxburgh, D. G.|title=A pseudo–elastic model for the Mullins effect in filled rubber.|journal=Proceedings of the Royal Society of London A|year=1999|issue=1988|volume=455|pages=2861–2877|doi=10.1098/rspa.1999.0431|bibcode=1999RSPSA.455.2861W}}
2. ^{{cite journal|last=Naumann|first=C.|author2=Ihlemann, J.|title=On the thermodynamics of pseudo-elastic material models which reproduce the Mullins effect|journal=International Journal of Solids and Structures|year=2015|volume=69–70|pages=360–369|doi=10.1016/j.ijsolstr.2015.05.014}}

L. Mullins, Rubber Chemistry and Technology, 42, 339 (1969).

4 : Continuum mechanics|Elasticity (physics)|Rubber properties|Solid mechanics

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。