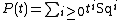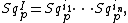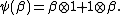- Cohomology operations
- Axiomatic characterization
- Ádem relations Bullett–Macdonald identities
- Computations Infinite Real Projective Space Infinite Complex Projective Space
- Construction
- The structure of the Steenrod algebra
- Hopf algebra structure and the Milnor basis
- Relation to formal groups
- Algebraic construction
- Applications
- Connection to the Adams spectral sequence and the homotopy groups of spheres
- See also
- References Pedagogical References
In algebraic topology, a Steenrod algebra was defined by {{harvs|txt|last=Cartan|first=Henri|authorlink=Henri Cartan|year=1955}} to be the algebra of stable cohomology operations for mod  cohomology.
cohomology.
For a given prime number  , the Steenrod algebra
, the Steenrod algebra  is the graded Hopf algebra over the field
is the graded Hopf algebra over the field 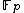 of order
of order  , consisting of all stable cohomology operations for mod
, consisting of all stable cohomology operations for mod  cohomology. It is generated by the Steenrod squares introduced by {{harvs|txt|last=Steenrod|first=Norman|authorlink=Norman Steenrod|year=1947}} for
cohomology. It is generated by the Steenrod squares introduced by {{harvs|txt|last=Steenrod|first=Norman|authorlink=Norman Steenrod|year=1947}} for  , and by the Steenrod reduced
, and by the Steenrod reduced  th powers introduced in {{harvtxt|Steenrod|1953}} and the Bockstein homomorphism for
th powers introduced in {{harvtxt|Steenrod|1953}} and the Bockstein homomorphism for  .
.
The term "Steenrod algebra" is also sometimes used for the algebra of cohomology operations of a generalized cohomology theory.
Cohomology operations
A cohomology operation is a natural transformation between cohomology functors. For example, if we take cohomology with coefficients in a ring, the cup product squaring operation yields a family of cohomology operations:
Cohomology operations need not be homomorphisms of graded rings; see the Cartan formula below.
These operations do not commute with suspension—that is, they are unstable. (This is because if  is a suspension of a space
is a suspension of a space  , the cup product on the cohomology of
, the cup product on the cohomology of  is trivial.) Steenrod constructed stable operations
is trivial.) Steenrod constructed stable operations
for all 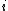 greater than zero. The notation
greater than zero. The notation  and their name, the Steenrod squares, comes from the fact that
and their name, the Steenrod squares, comes from the fact that  restricted to classes of degree
restricted to classes of degree  is the cup square. There are analogous operations for odd primary coefficients, usually denoted
is the cup square. There are analogous operations for odd primary coefficients, usually denoted  and called the reduced
and called the reduced  -th power operations:
-th power operations:
The  generate a connected graded algebra over
generate a connected graded algebra over 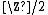 , where the multiplication is given by composition of operations. This is the mod 2 Steenrod algebra. In the case
, where the multiplication is given by composition of operations. This is the mod 2 Steenrod algebra. In the case  , the mod
, the mod  Steenrod algebra is generated by the
Steenrod algebra is generated by the  and the Bockstein operation
and the Bockstein operation 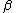 associated to the short exact sequence
associated to the short exact sequence
In the case  , the Bockstein element is
, the Bockstein element is  and the reduced
and the reduced  -th power
-th power  is
is 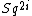 .
.
Axiomatic characterization
{{harvs|txt|last1=Steenrod|first1=Norman|authorlink1=Norman Steenrod|last2=Epstein|first2=David B. A. |authorlink2=David B. A. Epstein|year=1962}} showed that the Steenrod squares are characterized by the following 5 axioms:
are characterized by the following 5 axioms:- Naturality:
 is an additive homomorphism and is functorial with respect to any
is an additive homomorphism and is functorial with respect to any  so
so  .
.  is the identity homomorphism.
is the identity homomorphism. for
for 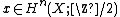 .
.- If
 then
then 
- Cartan Formula:

In addition the Steenrod squares have the following properties:
 is the Bockstein homomorphism
is the Bockstein homomorphism 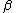 of the exact sequence
of the exact sequence 
 commutes with the connecting morphism of the long exact sequence in cohomology. In particular, it commutes with respect to suspension
commutes with the connecting morphism of the long exact sequence in cohomology. In particular, it commutes with respect to suspension 
- They satisfy the Ádem relations, described below
Similarly the following axioms characterize the reduced  -th powers for
-th powers for  .
.
- Naturality:
 is an additive homomorphism and natural.
is an additive homomorphism and natural. 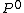 is the identity homomorphism.
is the identity homomorphism. is the cup
is the cup  -th power on classes of degree
-th power on classes of degree  .
.- If
 then
then 
- Cartan Formula:

As before, the reduced p-th powers also satisfy Ádem relations and commute with the suspension and boundary operators.
Ádem relations
The Ádem relations for  were conjectured by {{harvs|txt|last=Wu|first=Wen-tsün|authorlink=Wu Wenjun|year=1952}} and established by {{harvs|txt|authorlink=José Ádem|first=José|last=Ádem|year=1952}}. They are given by
were conjectured by {{harvs|txt|last=Wu|first=Wen-tsün|authorlink=Wu Wenjun|year=1952}} and established by {{harvs|txt|authorlink=José Ádem|first=José|last=Ádem|year=1952}}. They are given by
for all  such that
such that 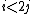 . (The binomial coefficients are to be interpreted mod 2.) The Ádem relations allow one to write an arbitrary composition of Steenrod squares as a sum of Serre–Cartan basis elements.
. (The binomial coefficients are to be interpreted mod 2.) The Ádem relations allow one to write an arbitrary composition of Steenrod squares as a sum of Serre–Cartan basis elements.
For odd  the Ádem relations are
the Ádem relations are
for a<pb and
for a≤pb
Bullett–Macdonald identities
{{harvs|txt|last1=Bullett|first1=Shaun R.|last2=Macdonald|first2=Ian G.|authorlink2=Ian G. Macdonald|year=1982}} reformulated the Ádem relations as the following identities.For  put
put
then the Ádem relations are equivalent to
For  put
put
then the Ádem relations are equivalent to the statement that
is symmetric in  and
and  . Here
. Here 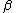 is the Bockstein operation and
is the Bockstein operation and  .
.
Computations
Infinite Real Projective Space
The Steenrod operations for real projective space can be readily computed using the formal properties of the Steenrod squares. Recall that
where  For the operations on
For the operations on  we know that
we know that
Using the operation
we note that the Cartan relation implies that
is a ring morphism. Hence
Since there is only one degree  component of the previous sum, we have that
component of the previous sum, we have that
Construction
Suppose that  is any degree
is any degree  subgroup of the symmetric group on
subgroup of the symmetric group on  points,
points,  a cohomology class in
a cohomology class in  ,
,  an abelian group acted on by
an abelian group acted on by  , and
, and  a cohomology class in
a cohomology class in  . {{harvtxt|Steenrod|1953}} showed how to construct a reduced power
. {{harvtxt|Steenrod|1953}} showed how to construct a reduced power  in
in  , as follows.
, as follows.
- Taking the external product of
 with itself
with itself  times gives an equivariant cocycle on
times gives an equivariant cocycle on  with coefficients in
with coefficients in  .
. - Choose
 to be a contractible space on which
to be a contractible space on which  acts freely and an equivariant map from
acts freely and an equivariant map from  to
to  Pulling back
Pulling back  by this map gives an equivariant cocyle on
by this map gives an equivariant cocyle on  and therefore a cocycle of
and therefore a cocycle of  with coefficients in
with coefficients in  .
. - Taking the slant product with
 in
in 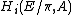 gives a cocycle of
gives a cocycle of  with coefficients in
with coefficients in  .
.
The Steenrod squares and reduced powers are special cases of this construction where  is a cyclic group of prime order
is a cyclic group of prime order  acting as a cyclic permutation of
acting as a cyclic permutation of  elements, and the groups
elements, and the groups  and
and  are cyclic of order
are cyclic of order  , so that
, so that  is also cyclic of order
is also cyclic of order  .
.
The structure of the Steenrod algebra
{{harvs|txt|last=Serre|first=Jean-Pierre|authorlink=Jean-Pierre Serre|year=1953}} (for ) and {{harvs|txt|authorlink=Henri Cartan|last=Cartan|first=Henri|year=1954|year2=1955}} (for
) and {{harvs|txt|authorlink=Henri Cartan|last=Cartan|first=Henri|year=1954|year2=1955}} (for  ) described the structure of the Steenrod algebra of stable mod
) described the structure of the Steenrod algebra of stable mod  cohomology operations, showing that it is generated by the Bockstein homomorphism together with the Steenrod reduced powers, and the Ádem relations generate the ideal of relations between these generators. In particular they found an explicit basis for the Steenrod algebra. This basis relies on a certain notion of admissibility for integer sequences. We say a sequence
cohomology operations, showing that it is generated by the Bockstein homomorphism together with the Steenrod reduced powers, and the Ádem relations generate the ideal of relations between these generators. In particular they found an explicit basis for the Steenrod algebra. This basis relies on a certain notion of admissibility for integer sequences. We say a sequenceis admissible if for each  , we have that
, we have that  . Then the elements
. Then the elements
where  is an admissible sequence, form a basis (the Serre–Cartan basis) for the mod 2 Steenrod algebra. There is a similar basis for the case
is an admissible sequence, form a basis (the Serre–Cartan basis) for the mod 2 Steenrod algebra. There is a similar basis for the case  consisting of the elements
consisting of the elements
such that
Hopf algebra structure and the Milnor basis
The Steenrod algebra has more structure than a graded Fp-algebra. It is also a Hopf algebra, so that in particular there is a diagonal or comultiplication map
induced by the Cartan formula for the action of the Steenrod algebra on the cup product.
It is easier to describe than the product map, and is given by
These formulas imply that the Steenrod algebra is co-commutative.
The linear dual of ψ makes the (graded) linear dual A* of A into an algebra. {{harvtxt|Milnor|1958}} proved, for p = 2, that A* is a polynomial algebra, with one generator ξk of degree 2k − 1, for every k, and for p > 2 the dual Steenrod algebra A* is the tensor product of the polynomial algebra in generators ξk of degree 2pk - 2 (k ≥ 1) and the exterior algebra in generators τk of degree 2pk - 1 (k ≥ 0). The monomial basis for A* then gives another choice of basis for A, called the Milnor basis. The dual to the Steenrod algebra is often more convenient to work with, because the multiplication is (super) commutative. The comultiplication for A* is the dual of the product on A; it is given by
where ξ0=1, and
if p>2
The only primitive elements of A* for p=2 are the  , and these are dual to the
, and these are dual to the  (the only indecomposables of A).
(the only indecomposables of A).
Relation to formal groups
The dual Steenrod algebras are supercommutative Hopf algebras, so their spectra are algebra supergroup schemes. These group schemes are closely related to the automorphisms of 1-dimensional additive formal groups. For example, if p=2 then the dual Steenrod algebra is the group scheme of automorphisms of the 1-dimensional additive formal group scheme x+y that are the identity to first order. These automorphisms are of the form
Algebraic construction
{{harvtxt|Smith|2007}} gave the following algebraic construction of the Steenrod algebra over a finite field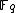 of order q. If V is a vector space over
of order q. If V is a vector space over 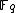 then write SV for the symmetric algebra of V. There is an algebra homomorphism
then write SV for the symmetric algebra of V. There is an algebra homomorphismwhere F is the Frobenius endomorphism of SV. If we put
or
for f∈SV then if V is infinite dimensional the elements Pi generate an algebra isomorphism to the subalgebra of the Steenrod algebra generated by the reduced p′th powers for p odd, or the even Steenrod squares Sq2i for p = 2.
Applications
The most famous early applications of the Steenrod algebra to outstanding topological problems were the solutions by J. Frank Adams of the Hopf invariant one problem and the vector fields on spheres problem. Independently Milnor and Bott, as well as Kervaire, gave a second solution of the Hopf invariant one problem, using operations in K-theory; these are the Adams operations. One application of the mod 2 Steenrod algebra that is fairly elementary is the following theorem.
Theorem. If there is a map S2n - 1 → Sn of Hopf invariant one, then n is a power of 2.
The proof uses the fact that each Sqk is decomposable for k which is not a power of 2;
that is, such an element is a product of squares of strictly smaller degree.
Connection to the Adams spectral sequence and the homotopy groups of spheres
The cohomology of the Steenrod algebra is the E2 term for the (p-local) Adams spectral sequence, whose abutment is the p-component of the stable homotopy groups of spheres. More specifically, the E2 term of this spectral sequence may be identified as
This is what is meant by the aphorism "the cohomology of the Steenrod algebra is an approximation to the stable homotopy groups of spheres."
See also
- Pontryagin cohomology operation
References
Pedagogical
- {{Citation | last=Malkiewich | first=Cary | title=The Steenrod Algebra | url=http://www.math.uiuc.edu/~cmalkiew/steenrod.pdf | deadurl=bot: unknown | archiveurl=https://web.archive.org/web/20170815173103/http://www.math.uiuc.edu/~cmalkiew/steenrod.pdf | archivedate=2017-08-15 | df= }}
References
- {{Citation | last=Ádem | first=José | authorlink=José Ádem|title=The iteration of the Steenrod squares in algebraic topology | jstor=88494 | mr=0050278 | year=1952 | journal=Proceedings of the National Academy of Sciences of the United States of America | issn=0027-8424 | volume=38 | issue=8 | pages=720–726 | doi=10.1073/pnas.38.8.720| pmid=16589167 | pmc=1063640 | bibcode=1952PNAS...38..720A }}
- {{Citation | last1=Bullett | first1=S. R. | last2=Macdonald | first2=I. G. | author2-link=Ian G. Macdonald | title=On the Adem relations | doi=10.1016/0040-9383(82)90015-5 | mr=649764 | year=1982 | journal=Topology. An International Journal of Mathematics | issn=0040-9383 | volume=21 | issue=3 | pages=329–332}}
- {{Citation | last1=Cartan | first1=Henri | title=Sur les groupes d'Eilenberg-Mac Lane. II | jstor=88981 | mr=0065161 | year=1954 | journal=Proceedings of the National Academy of Sciences of the United States of America | issn=0027-8424 | volume=40 | issue=8 | pages=704–707 | doi=10.1073/pnas.40.8.704| pmid=16589542 | pmc=534145 | bibcode=1954PNAS...40..704C }}
- {{Citation | last1=Cartan | first1=Henri | title=Sur l'itération des opérations de Steenrod | doi=10.1007/BF02564270 | mr=0068219 | year=1955 | journal=Commentarii Mathematici Helvetici | issn=0010-2571 | volume=29 | issue=1 | pages=40–58}}
- Allen Hatcher, Algebraic Topology. Cambridge University Press, 2002. Available free online from the author's home page.
- {{eom|id=S/s087550|title=Steenrod reduced power |first=S.N.|last= Malygin|first2=M.M.|last2= Postnikov}}
- {{eom|id=S/s087560|title=Steenrod square |first=S.N.|last= Malygin|first2=M.M.|last2= Postnikov}}
- {{Citation | last1=May | first1=J. Peter | author1-link=J. Peter May | title=The Steenrod Algebra and its Applications (Proc. Conf. to Celebrate N. E. Steenrod's Sixtieth Birthday, Battelle Memorial Inst., Columbus, Ohio, 1970) | chapter-url=http://www.math.uchicago.edu/~may/PAPERS/10.pdf | publisher=Springer-Verlag | location=Berlin, New York | series=Lecture Notes in Mathematics | doi=10.1007/BFb0058524 | mr=0281196 | year=1970 | volume=168 | chapter=A general algebraic approach to Steenrod operations | pages=153–231| isbn=978-3-540-05300-2 | citeseerx=10.1.1.205.6640 }}
- {{Citation | last1=Milnor | first1=John Willard | author1-link=John Milnor | title=The Steenrod algebra and its dual | jstor=1969932 | mr=0099653 | year=1958 | journal=Annals of Mathematics |series=Second Series | issn=0003-486X | volume=67 | issue=1 | pages=150–171 | doi=10.2307/1969932}}
- {{Citation | last1=Mosher | first1=Robert E. | last2=Tangora | first2=Martin C. | title=Cohomology operations and applications in homotopy theory | origyear=1968 | url=https://books.google.com/books?id=FFCaPwAACAAJ | publisher=Dover Publications | location=New York | isbn=978-0-486-46664-4 | mr=0226634 | year=2008}}
- {{eom|id=S/s087500|first=Yu.B. |last=Rudyak}}
- {{Citation | last1=Serre | first1=Jean-Pierre | author1-link=Jean-Pierre Serre | title=Cohomologie modulo 2 des complexes d'Eilenberg-MacLane | doi=10.1007/BF02564562 | mr=0060234 | year=1953 | journal=Commentarii Mathematici Helvetici | issn=0010-2571 | volume=27 | issue=1 | pages=198–232}}
- {{Citation | last1=Smith | first1=Larry | editor1-last=Hubbuck | editor1-first=John | editor2-last=Hu'ng | editor2-first=Nguyễn H. V. | editor3-last=Schwartz | editor3-first=Lionel | title=Proceedings of the School and Conference in Algebraic Topology | journal=Geom. Topol. Monogr | arxiv=0903.4997 | series=Geometry & Topology Monographs | mr=2402812 | year=2007 | volume=11 | chapter=An algebraic introduction to the Steenrod algebra | pages=327–348 | doi=10.2140/gtm.2007.11.327}}
- {{Citation | last1=Steenrod | first1=N. E. | title=Products of cocycles and extensions of mappings | jstor=1969172 | mr=0022071 | year=1947 | journal=Annals of Mathematics |series=Second Series | issn=0003-486X | volume=48 | issue=2 | pages=290–320 | doi=10.2307/1969172}}
- {{Citation | last1=Steenrod | first1=N. E. | title=Homology groups of symmetric groups and reduced power operations | jstor=88780 | mr=0054964 | year=1953 | journal=Proceedings of the National Academy of Sciences of the United States of America | issn=0027-8424 | volume=39 | issue=3 | pages=213–217 | doi=10.1073/pnas.39.3.213| pmid=16589250 | pmc=1063756 | bibcode=1953PNAS...39..213S }}
- {{Citation | last1=Steenrod | first1=N. E. | title=Cyclic reduced powers of cohomology classes | jstor=88781 | mr=0054965 | year=1953 | journal=Proceedings of the National Academy of Sciences of the United States of America | issn=0027-8424 | volume=39 | issue=3 | pages=217–223 | doi=10.1073/pnas.39.3.217| pmid=16589251 | pmc=1063757 | bibcode=1953PNAS...39..217S }}
- {{Citation | last1=Steenrod | first1=N. E. | editor1-last=Epstein | editor1-first=D. B. A. | editor1-link=David B. A. Epstein | title=Cohomology operations | url=https://books.google.com/books?id=CF3bt4oYZ2oC | publisher=Princeton University Press | series= Annals of Mathematics Studies | isbn=978-0-691-07924-0 | mr=0145525 | year=1962 | volume=50}}
- {{Citation | last=Wu|first=Wen-tsün|authorlink=Wu Wenjun | title=Sur les puissances de Steenrod | publisher=La Bibliothèque Nationale et Universitaire de Strasbourg | series=Colloque de Topologie de Strasbourg | mr=0051510 | year=1952 | volume=IX}}
- Manuel Antonio Rosales Guerrero
- Manuel Antonio Sanclemente
- Manuel Antonio Santiago Tarin
- Manuel António de Almeida
- Manuel António Marques Machado
- Manuel António Vassalo e Silva
- Manuel Antônio de Almeida
- Manuel Antônio Álvares de Azevedo
- Manuela Oschmann
- Manuela Pesko
- Manuela Pfrunder
- Manuel A. Quintana
- Manuel A. Quintana y Sáenz de Gaona
- Manuela Ramin-Osmundsen
- Manuel Aranda da Silva
- Manuel Araneta Roxas II
- Manuel Araullo
- Manuel Arboleda
- Manuel Arce y Ochotorena
- Manuel Argüello Mora
- Manuel Aringarosa
- Manuel Armijo
- Manuel A. Roxas II
- Manuel A. Roxas, II
- Manuel Arredondo y Pelegrin