- Background and statement
- Development
- References
The conjecture has been proven for n ≥ 5 by Jeff Xia.[3][4] The 4-particle case remains an open problem.[5]
Background and statement
Solutions  of the n-body problem
of the n-body problem 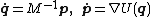 (where M are the masses and U denotes the gravitational potential) are said to have a singularity if there is a sequence of times
(where M are the masses and U denotes the gravitational potential) are said to have a singularity if there is a sequence of times  converging to a finite
converging to a finite  where
where 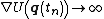 . That is, the forces and accelerations become infinite at some finite point in time.
. That is, the forces and accelerations become infinite at some finite point in time.
A collision singularity occurs if  tends to a definite limit when
tends to a definite limit when  . If the limit does not exist the singularity is called a pseudocollision or noncollision singularity.
. If the limit does not exist the singularity is called a pseudocollision or noncollision singularity.
Paul Painlevé showed that for n = 3 any solution with a finite time singularity experiences a collision singularity. However, he failed at extending this result beyond 3 bodies. His 1895 Stockholm lectures end with the conjecture that
For n ≥ 4 the n-body problem admits noncollision singularities.[6][7]
Development
Edvard Hugo von Zeipel proved in 1908 that if there is a collision singularity, then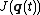 tends to a definite limit as
tends to a definite limit as 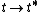 , where
, where 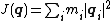 is the moment of inertia.[8] This implies that a necessary condition for a noncollision singularity is that the velocity of at least one particle becomes unbounded (since the positions
is the moment of inertia.[8] This implies that a necessary condition for a noncollision singularity is that the velocity of at least one particle becomes unbounded (since the positions  remain finite up to this point).[1]
remain finite up to this point).[1]Mather and McGehee managed to prove in 1975 that a noncollision singularity can occur in the co-linear 4-body problem (that is, with all bodies on a line), but only after an infinite number of (regularized) binary collisions.[9]
Donald G. Saari proved in 1977 that for almost all (in the sense of Lebesgue measure) initial conditions in the plane or space for 2, 3 and 4-body problems there are singularity-free solutions.[10]In 1984 Joe Gerver gave an argument for a noncollision singularity in the planar 5-body problem with no collisions.[11] He later found a proof for the 3n body case.[12]
Finally, in his 1988 doctoral dissertation, Jeff Xia demonstrated a 5-body configuration that experiences a noncollision singularity.[3][4]
Joe Gerver has given a heuristic model for the existence of 4-body singularities[13] but at present no formal proof exists.
In his 2013 doctoral thesis at University of Maryland, Jinxin Xue considered a simplified model for the planar four-body problem case of the Painlevé conjecture. Based on a model of Gerver, he proved that there is a Cantor set of initial conditions which lead to solutions of the Hamiltonian system whose velocities are accelerated to infinity within finite time avoiding all earlier collisions. [14]
References
2. ^Florin Diacu, Philip Holmes, Celestial Encounters: The Origins of Chaos and Stability. Princeton University Press, 1996
3. ^1 Zhihong Xia. The Existence of Noncollision Singularities in Newtonian Systems. Annals of Mathematics. Second Series, Vol. 135, No. 3 (May, 1992), pp. 411–468
4. ^1 Donald G. Saari and Zhihong (Jeff) Xia. Off to Infinity in Finite Time. Notices of the AMS, vol 42, no. 5, 1993, pp. 538–546
5. ^Edward Belbruno. Capture Dynamics and Chaotic Motions in Celestial Mechanics. Princeton University Press, 2004 p. 8
6. ^P. Painlevé, Lecons sur la théorie analytique des équations différentielles, ParisÖ Hermann, 1897
7. ^Oeuvres de Paul Painlevé, Tome I, Paris Ed. Centr. Nat. Rech. Sci., 1972
8. ^H. von Zeipel, Sur les singularités du problème des corps, Arkiv för Mat. Astron. Fys. 4, (1908), 1–4.
9. ^J. Mather and R. McGehee, Solutions of the collinear four-body problem which become unbounded in finite time, Dynamical Systems Theory and Applications (J. Moser, ed.), Berlin: Springer-Verlag, 1975, 573–589.
10. ^Donald G. Saari. A global existence theorem for the four-body problem of Newtonian mechanics, J. Differential Equations 26 (1977), 80–111.
11. ^J. L. Gerver, A possible model for a singularity without collisions in the five-body problem, J. Diff. Eq. 52 (1984), 76–90.
12. ^J. L. Gerver, The existence of pseudocollisions in the plane, J. Diff. Eq. 89 (1991), 1-68.
13. ^Joseph L. Gerver, Noncollision Singularities: Do Four Bodies Suffice?, Exp. Math. (2003), 187–198.
14. ^Xue, J. & Dolgopyat, D. Commun. Math. Phys. (2016) 345: 797. https://doi.org/10.1007/s00220-016-2688-6
- OoH
- OOH
- OOh
- Ooh, Aah and You
- Ooh, Aah, Just A Little Bit
- Ooh Aah...Just a Little Bit
- Ooh Aah Just a Little Bit
- Ooh Aah... Just A Little Bit
- Ooh Aah (Tamara song)
- Ooh Ahh (GRITS song)
- Ooh Ahh (Tamara Jaber song)
- Oohara Sayaka
- Oohara Takashi
- Ooh arr
- Ooh Baby (Mario song)
- O-o-h Child
- Oohira
- Ooh, it's am
- Ooh La La (album)
- Ooh La La (Crash Test Dummies album)
- Ooh La La (disambiguation)
- Ooh La La (Faces song)
- Ooh La La (Odd Man Out episode)
- Ooh La La (song)
- OOH Lincoln L.F.C.