“Quantum cylindrical quadrupole”的意思、由来-开放百科全书

词条

Quantum cylindrical quadrupole

释义

Azimuthal equation
References
See also
External links

The solution to the Schrödinger equation, the wavefunction, describes the quantum mechanical properties of a particle on microscopic scales. Measurable quantities such as position, momentum and energy are all derived from the wavefunction.^[1]

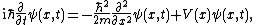
where is the reduced Planck constant, is the mass of the particle, 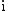is the imaginary unit and is time.

One peculiar potential that can be solved exactly is when the electric quadrupole moment is the dominant term of an infinitely long cylinder of charge.

It can be shown that the Schrödinger equation is solvable for a cylindrically symmetric electric quadrupole, thus indicating that the quadrupole term of an infinitely long cylinder can be quantized.

In the physics of classical electrodynamics, it can be easily shown that the scalar potential and associated mechanical potential energy of a cylindrically symmetric quadrupole is as follows:

(SI units)

Cylindrical symmetry should be used when solving the equation. The time independent Schrödinger equation becomes the following in cylindrical symmetry.

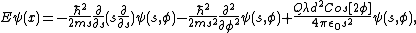
Using the technique Separation of Variables, the above equation can be written as two ordinary differential equations in both the radial and azimuthal directions. The radial equation is Bessel's equation as can be seen below. If one changes variables to 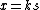, Bessel's equation is exactly obtained.

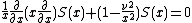
Azimuthal equation

The azimuthal equation is given by

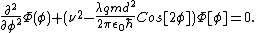
This is the Mathieu equation,

with and .

The solution of the Mathieu equation is expressed in terms of the Mathieu cosine 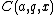and the Mathieu sine for unique a and q. This indicates that the quadrupole moment can be quantized in order of the Mathieu characteristic values and .

In general, Mathieu functions are not periodic. The term q must be that of a characteristic value in order for Mathieu functions to be periodic. It can be shown that the solution of the radial equation highly depends on what characteristic values are seen in this case.

References

1. ^Davies, p. 1

External links

MULTIPOLE EXPANSION
The nonvanishing coefficients of the dipole moment expansion in axially symmetric molecules

1 : Quantum mechanics

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。

Azimuthal equation

References

See also

External links