- Research contributions
- Professional service
- Awards and honors
- Selected publications Papers Books
- References
- External links
| name = William Goldman
| birth_date = {{birth date and age|1955|11|17}}
| birth_place = Kansas City, United States
| nationality = American
| field = Mathematics
| work_institutions = University of Maryland-College Park
| alma_mater = Princeton University
University of California, Berkeley
| doctoral_advisors = Morris Hirsch
William Thurston
}}
William Mark Goldman (born 1955 in Kansas City, Missouri) is a professor of mathematics at the University of Maryland, College Park (since 1986). He received a B.A. in mathematics from Princeton University in 1977, and a Ph.D. in mathematics from the University of California, Berkeley in 1980.
Research contributions
Goldman has investigated geometric structures, in various incarnations, on manifolds since his undergraduate thesis, "Affine manifolds and projective geometry on manifolds" (supervised by William Thurston and Dennis Sullivan). This work led to work with Morris Hirsch and David Fried on affine structures on manifolds, and work in real projective structures on compact surfaces. In particular he proved that the space of convex real projective structures on a closed orientable surface of genus  is homeomorphic to an open cell of dimension
is homeomorphic to an open cell of dimension 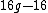 . With Suhyoung Choi, he proved that this space is a connected component (the "Hitchin component") of the space of equivalence classes of representations of the fundamental group in
. With Suhyoung Choi, he proved that this space is a connected component (the "Hitchin component") of the space of equivalence classes of representations of the fundamental group in  . Combining this with Suhyoung Choi's convex decomposition theorem, this led to a complete classification of convex real projective structures on compact surfaces.
. Combining this with Suhyoung Choi's convex decomposition theorem, this led to a complete classification of convex real projective structures on compact surfaces.
His doctoral dissertation, "Discontinuous groups and the Euler class" (supervised by Morris W. Hirsch), characterizes discrete embeddings of surface groups in 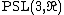 in terms of maximal Euler class, proving a converse to the Milnor–Wood inequality for
in terms of maximal Euler class, proving a converse to the Milnor–Wood inequality for
flat bundles. Shortly thereafter he showed that the space of
representations of the fundamental group of a closed orientable surface of genus  in
in 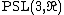 has
has  connected components, distinguished by the Euler class.
connected components, distinguished by the Euler class.
With David Fried, he classified compact quotients of Euclidean 3-space by discrete groups of affine transformations, showing that all such manifolds are finite quotients of torus bundles over the circle. The noncompact case is much more interesting, as Grigory Margulis found complete affine manifolds with nonabelian free fundamental group. In his 1990 doctoral thesis, Todd Drumm found examples which are solid handlebodies using polyhedra which have since been called "crooked planes."
He found examples (non-Euclidean nilmanifolds and solvmanifolds) of closed 3-manifolds which fail to admit flat conformal structures.
Generalizing Scott Wolpert's work on the Weil–Petersson symplectic structure on the space of hyperbolic structures on surfaces, he found an algebraic-topological description of a symplectic structure on spaces of representations of a surface group in a reductive Lie group. Traces of representations of the corresponding curves on the surfaces generate a Poisson algebra, whose Lie bracket has a topological description in terms of the intersections of curves. Furthermore, the Hamiltonian vector fields of these trace functions define flows generalizing the Fenchel–Nielsen flows on Teichmüller space. This symplectic structure is invariant under the natural action of the mapping class group, and using the relationship between Dehn twists and the generalized Fenchel–Nielsen flows, he proved the ergodicity of the action of the mapping class group on the SU(2)-character variety with respect to symplectic Lebesgue measure.
Following suggestions of Pierre Deligne, he and John Millson proved that the variety of representations of the fundamental group of a compact Kähler manifold has singularities defined by systems of homogeneous quadratic equations. This leads to various local rigidity results for actions on Hermitian symmetric spaces.
With John Parker, he examined the complex hyperbolic ideal triangle group representations. These are representations of hyperbolic ideal triangle groups to the group of holomorphic isometries of the complex hyperbolic plane such that each standard generator of the triangle group maps to a C-reflection and the products of pairs of generators to parabolics. The space of representations for a given triangle group (modulo conjugacy) is parametrized by a half-open interval. They showed that the representations in a particular range were discrete and conjectured that a representation would be discrete if and only if it was in a specified larger range. This has become known as the Goldman–Parker conjecture and was eventually proven by Richard Schwartz.
Professional service
Professor Goldman also heads a research group at the University of Maryland called the Experimental Geometry Lab, a team developing software (primarily in Mathematica) to explore geometric structures and dynamics in low dimensions.
He served on the Board of Governors for The Geometry Center at the University of Minnesota from 1994 to 1996.
He served as Editor-In-Chief of Geometriae Dedicata from 2003 until 2013.
Awards and honors
In 2012 he became a fellow of the American Mathematical Society.[1]
Selected publications
Papers
- William Goldman, "On the polynomial cohomology of affine manifolds", Invent. Math. 65 (1981/82), no. 3, 453–457.
- William Goldman and Morris Hirsch, "A generalization of Bieberbach's theorem", Invent. Math. 65 (1981/82), no. 1, 1–11.
- David Fried and William Goldman, "Three-dimensional affine crystallographic groups", Advances in Mathematics 47 (1983), 1--49.
- William Goldman, "Conformally flat manifolds with nilpotent holonomy and the uniformization problem for 3-manifolds", Transactions of the American Mathematical Society 278 (1983), 573–583.
- William Goldman, "The symplectic nature of fundamental groups of surfaces," Advances in Mathematics 54 (1984), 200–225.
- William Goldman, "Invariant functions on Lie groups and Hamiltonian flows of surface group representations", Invent. Math. 85 (1986), no. 2, 263–302.
- William Goldman and John J. Millson, "Local rigidity of discrete groups acting on complex hyperbolic space", Invent. Math. 88 (1987), no. 3, 495–520.
- William Goldman, "Geometric structures on manifolds and varieties of representations", Geometry of group representations (Boulder CO, 1987), 169–198, Contemp. Math., 74, Amer. Math. Soc., Providence, RI, 1988.
- William Goldman, "Topological components of spaces of representations", Invent. Math. 93 (1988), no. 3, 557–607.
- William Goldman and John Millson, "The deformation theory of representations of fundamental groups of Kaehler manifolds," Publications Mathematiques d'Institut des Hautes Etudes Scientifiques, 67 (1988), 43–96.
- William Goldman Convex real projective structures on compact surfaces, Journal of Differential Geometry 31 (1990), 791–845.
- William Goldman and John Parker, "Complex hyperbolic ideal triangle groups", J. Reine Angew. Math. 425 (1992), 71–86.
- William Goldman, "Ergodic theory on moduli spaces", Ann. of Math. (2) 146 (1997), no. 3, 475–507.
- Suhyoung Choi and William Goldman, "The Classification of Real Projective Structures on compact surfaces", Bull. Amer. Math. Soc. 34 (2) (1997), 161–170.
- Todd Drumm and William Goldman, "The Geometry of Crooked Planes", Topology 38 (2) (1999), 323–351.
- William Goldman, Michael Kapovich, and Bernhard Leeb; "Complex hyperbolic manifolds homotopy equivalent to a Riemann surface", Comm. Anal. Geom. 9 (2001), no. 1, 61–95.
- William Goldman, "Action of the modular group on real SL(2)-characters of a one- holed torus", Geometry and Topology 7 (2003), 443–486
- William Goldman, WHAT IS...a Projective Structure?, Notices of the AMS 54 (1) (2007), 30–33
- William Goldman, François Labourie and Gregory Margulis, "Proper affine actions and geodesic flows of hyperbolic surfaces", Ann. of Math. 170 (2009), 1051–1083.
- William Goldman, "Locally homogeneous geometric manifolds", Proceedings of the 2010 International Congress of Mathematicians, Hyderabad, India (2010), 717–744, Hindustan Book Agency, New Delhi, India
- William Goldman, "Higgs bundles and geometric structures on surfaces", in The Many Facets of Geometry: a Tribute to Nigel Hitchin, O. García- Prada, J.P. Bourgignon, and S. Salamon (eds.), Oxford University Press (2010) 129 – 163
- Virginie Charette, Todd Drumm and William Goldman, "Affine deformations of the three-holed sphere", Geometry & Topology 14 (2010), 1355-1382
- Wiliam Goldman and Eugene Xia, "Ergodicity of mapping class group action on SU(2)- character varieties", in “Geometry, rigidity, and group actions”, Chicago Lectures in Math., University of Chicago Press (2011)
Books
- William Goldman, Complex hyperbolic geometry. Oxford Mathematical Monographs. Oxford Science Publications. The Clarendon Press, Oxford University Press, New York, 1999. xx+316 pp. {{ISBN|0-19-853793-X}}
References
External links
- Faculty page at the University of Maryland, College Park
- Hao Zhi
- Hao Zhou
- Haozhuang
- Haozhuang (disambiguation)
- Haozhuang Township
- Haozhuang Township, Hebei
- HAP1 cells
- HAP-302
- Hapag LLoyd Flug
- Hapag-Lloyd Fluggesellschaft mBH
- Hapag-Lloyd Flugzeug GmbH
- Hapa language
- Hapalanthus
- Hapalia aenealis
- Hapalia bambusalis
- Hapalia bifossata
- Hapalia carbonifusalis
- Hapalia cascalis
- Hapalia conisanalis
- Hapalia conistolalis
- Hapalia denticulosa
- Hapalia distictalis
- Hapalia dorsivittata
- Hapalia endotrichialis
- Hapalia fasciata