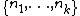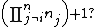- The problem
- History
- Examples
- Connection to Egyptian fractions
- Number of solutions
- References
- External links
In number theory, Znám's problem asks which sets of k integers have the property that each integer in the set is a proper divisor of the product of the other integers in the set, plus 1. Znám's problem is named after the Slovak mathematician Štefan Znám, who suggested it in 1972, although other mathematicians had considered similar problems around the same time. One closely related problem drops the assumption of properness of the divisor, and will be called the improper Znám problem hereafter.
One solution to the improper Znám problem is easily provided for any k: the first k terms of Sylvester's sequence have the required property. {{harvtxt|Sun|1983}} showed that there is at least one solution to the (proper) Znám problem for each k ≥ 5. Sun's solution is based on a recurrence similar to that for Sylvester's sequence, but with a different set of initial values.
The Znám problem is closely related to Egyptian fractions. It is known that there are only finitely many solutions for any fixed k. It is unknown whether there are any solutions to Znám's problem using only odd numbers, and there remain several other open questions.
The problem
Znám's problem asks which sets of integers have the property that each integer in the set is a proper divisor of the product of the other integers in the set, plus 1. That is, given k, what sets of integers
are there, such that, for each i, ni divides but is not equal to
A closely related problem concerns sets of integers in which each integer in the set is a divisor, but not necessarily a proper divisor, of one plus the product of the other integers in the set. This problem does not seem to have been named in the literature, and will be referred to as the improper Znám problem. Any solution to Znám's problem is also a solution to the improper Znám problem, but not necessarily vice versa.
History
Znám's problem is named after the Slovak mathematician Štefan Znám, who suggested it in 1972. {{harvtxt|Barbeau|1971}} had posed the improper Znám problem for k = 3, and {{harvtxt|Mordell|1973}}, independently of Znám, found all solutions to the improper problem for k ≤ 5. {{harvtxt|Skula|1975}} showed that Znám's problem is unsolvable for k < 5, and credited J. Janák with finding the solution {2, 3, 11, 23, 31} for k = 5.
Examples
One solution to k = 5 is {2, 3, 7, 47, 395}. A few calculations will show that
| 3 × 7 × 47 × 395 | + 1 = | 389866, | which is divisible by but unequal to 2, | |
| 2 × 7 × 47 × 395 | + 1 = | 259911, | which is divisible by but unequal to 3, | |
| 2 × 3 × 47 × 395 | + 1 = | 111391, | which is divisible by but unequal to 7, | |
| 2 × 3 × 7 × 395 | + 1 = | 16591, | which is divisible by but unequal to 47, and | |
| 2 × 3 × 7 × 47 | + 1 = | 1975, | which is divisible by but unequal to 395. |
An interesting "near miss" for k = 4 is the set {2, 3, 7, 43}, formed by taking the first four terms of Sylvester's sequence. It has the property that each integer in the set divides the product of the other integers in the set, plus 1, but the last member of this set is equal to the product of the first three members plus one, rather than being a proper divisor. Thus, it is a solution to the improper Znám problem, but not a solution to Znám's problem as it is usually defined.
Connection to Egyptian fractions
Any solution to the improper Znám problem is equivalent (via division by the product of the xi's) to a solution to the equation
where y as well as each xi must be an integer, and conversely any such solution corresponds to a solution to the improper Znám problem. However, all known solutions have y = 1, so they satisfy the equation
That is, they lead to an Egyptian fraction representation of the number one as a sum of unit fractions. Several of the cited papers on Znám's problem study also the solutions to this equation. {{harvtxt|Brenton|Hill|1988}} describe an application of the equation in topology, to the classification of singularities on surfaces, and {{harvtxt|Domaratzki|Ellul|Shallit|Wang|2005}} describe an application to the theory of nondeterministic finite automata.
Number of solutions
As {{harvtxt|Janák|Skula|1978}} showed, the number of solutions for any k is finite, so it makes sense to count the total number of solutions for each k.
Brenton and Vasiliu calculated that the number of solutions for small values of k, starting with k = 5, forms the sequence
2, 5, 18, 96 {{OEIS|id=A075441}}.
Presently, a few solutions are known for k = 9 and k = 10, but it is unclear how many solutions remain undiscovered for those values of k.
However, there are infinitely many solutions if k is not fixed:
{{harvtxt|Cao|Jing|1998}} showed that there are at least 39 solutions for each k ≥ 12, improving earlier results proving the existence of fewer solutions ({{harvnb|Cao|Liu|Zhang|1987}}, {{harvnb|Sun|Cao|1988}}). {{harvtxt|Sun|Cao|1988}} conjecture that the number of solutions for each value of k grows monotonically with k.It is unknown whether there are any solutions to Znám's problem using only odd numbers. With one exception, all known solutions start with 2. If all numbers in a solution to Znám's problem or the improper Znám problem are prime, their product is a primary pseudoperfect number {{harv|Butske|Jaje|Mayernik|2000}}; it is unknown whether infinitely many solutions of this type exist.
References
{{refbegin|2}}- {{citation
| last = Barbeau | first = G. E. J.
| title = Problem 179
| journal = Canadian Mathematical Bulletin
| volume = 14
| year = 1971
| issue = 1
| pages = 129}}.
- {{citation
| last = Brenton| first = Lawrence
| last2 = Hill | first2 = Richard
| title = On the Diophantine equation 1=Σ1/ni + 1/Πni and a class of homologically trivial complex surface singularities
| journal = Pacific Journal of Mathematics
| url = http://projecteuclid.org/Dienst/UI/1.0/Summarize/euclid.pjm/1102689567
| volume = 133
| issue = 1
| year = 1988
| pages = 41–67
| mr = 0936356
| doi=10.2140/pjm.1988.133.41}}.
- {{citation
| doi = 10.2307/3219178
| last = Brenton| first = Lawrence
| last2 = Vasiliu| first2 = Ana
| title = Znám's problem
| journal = Mathematics Magazine
| volume = 75
| issue = 1
| pages = 3–11
| year = 2002
| jstor = 3219178}}.
- {{citation
| last = Butske| first = William
| last2= Jaje|first2= Lynda M.
| last3 = Mayernik| first3 = Daniel R.
| title = On the equation
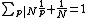 , pseudoperfect numbers, and perfectly weighted graphs
, pseudoperfect numbers, and perfectly weighted graphs| journal = Mathematics of Computation
| volume = 69
| year = 2000
| pages = 407–420
| url = http://www.ams.org/mcom/2000-69-229/S0025-5718-99-01088-1/home.html
| mr = 1648363 | doi=10.1090/S0025-5718-99-01088-1}}.
- {{citation
| last = Cao |first= Zhen Fu
| last2 = Jing| first2= Cheng Ming
| title = On the number of solutions of Znám's problem
| journal = J. Harbin Inst. Tech.
| volume = 30
| year = 1998
| issue = 1
| pages = 46–49
| mr = 1651784}}.
- {{citation
| last = Cao| first = Zhen Fu
| last2 = Liu| first2 = Rui
| last3 = Zhang| first3= Liang Rui
| title = On the equation
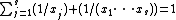 and Znám's problem
and Znám's problem| journal = Journal of Number Theory
| volume = 27
| year = 1987
| issue = 2
| pages = 206–211
| mr = 0909837
| doi = 10.1016/0022-314X(87)90062-X}}.
- {{citation
| last = Domaratzki| first = Michael
| last2 = Ellul | first2 = Keith
| last3 = Shallit| first3 = Jeffrey
| last4 = Wang| first4 = Ming-Wei
| title = Non-uniqueness and radius of cyclic unary NFAs
| journal = International Journal of Foundations of Computer Science
| volume = 16
| issue = 5
| pages = 883–896
| year = 2005
| url = http://www.cs.umanitoba.ca/~mdomarat/pubs/DESW_dcfs.ps
| mr = 2174328
| doi = 10.1142/S0129054105003352}}.
- {{citation
| last = Janák| first = Jaroslav
| last2= Skula| first2= Ladislav | authorlink2 = Ladislav Skula
| title = On the integers
 for which
for which 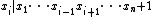
| journal = Math. Slovaca
| volume = 28
| year = 1978
| issue = 3
| pages = 305–310
| mr = 0534998}}.
- {{citation
| last = Mordell| first = L. J.
| title = Systems of congruences
| journal = Canadian Mathematical Bulletin
| volume = 16
| year = 1973
| pages = 457–462
| mr = 0332650
| doi = 10.4153/CMB-1973-077-3}}.
- {{citation
| last = Skula| first= Ladislav | authorlink = Ladislav Skula
| title = On a problem of Znám
| type = Russian, Slovak summary
| journal = Acta Fac. Rerum Natur. Univ. Comenian. Math.
| volume = 32
| year = 1975
| pages = 87–90
| mr = 0539862}}.
- {{citation
| last = Sun| first= Qi
| title = On a problem of Š. Znám
| journal = Sichuan Daxue Xuebao
| year = 1983
| issue = 4
| pages = 9–12
| mr = 0750288}}.
- {{citation
| last = Sun| first = Qi
| last2= Cao| first2 = Zhen Fu
| title = On the equation
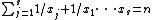 and the number of solutions of Znám's problem
and the number of solutions of Znám's problem| journal = Northeastern Mathematics Journal
| volume = 4
| year = 1988
| issue = 1
| pages = 43–48
| mr = 0970644}}.{{refend}}
External links
- {{cite web
| author = Primefan
| url = http://primefan.tripod.com/ZnamProbSols.html
| title = Solutions to Znám's Problem}}
- {{mathworld | title = Znám's Problem | urlname = ZnamsProblem}}
- Interball
- Interball, space project
- Inter (band)
- Interbank Burundi
- Interbank foreign exchange market
- Interbank lending market
- Interbank markets
- Interbank Mobile Payment System
- Interbasin transfer
- Inter-basin transfer
- Interbasin water diversion
- Interbasin water transfer
- Interbay, Florida
- Interbay, Seattle, Washington
- Interbay, Tampa
- Interbay (Tampa)
- Interbay, Tampa, Florida
- Interbay Yard
- Interbeat interval
- Interbedded
- Interbedding
- Interbellum Generation
- Interbellum period
- Interbellum Poland
- Interbeton