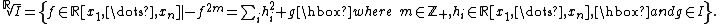- Definition
- References
The real radical of an ideal I in a polynomial ring with real coefficients is the largest ideal containing I with the same vanishing locus.
It plays a similar role in real algebraic geometry that the radical of an ideal plays in algebraic geometry over an algebraically closed field.
More specifically, the nullstellensatz says that when I is an ideal in a polynomial ring with coefficients coming from an algebraically closed field, the radical of I is the set of polynomials vanishing on the vanishing locus of I. In real algebraic geometry, the nullstellensatz fails as the real numbers are not algebraically closed. However, one can recover a similar theorem, the real nullstellensatz, by using the real radical in place of the (ordinary) radical.
Definition
The real radical of an ideal I in a polynomial ring 
over the real numbers, denoted by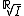 
, is defined as
The Positivstellensatz then implies that 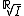is the set of all polynomials that vanish on the real variety defined by the vanishing of 
.
References
- Marshall, Murray Positive polynomials and sums of squares. Mathematical Surveys and Monographs, 146. American Mathematical Society, Providence, RI, 2008. xii+187 pp. {{ISBN|978-0-8218-4402-1}}; 0-8218-4402-4
- Limited partnership
- Limited Partnership
- Limited resource
- Limited-run manufacturing
- Limited Slip Differential
- Limited-slip differential
- Limited (solitaire)
- Limited-stop
- Limited Test Ban Treaty
- Limited voting
- Limited war
- Limiter
- Limit inferior
- Limiting
- Limiting factor
- Limiting magnitude
- Limiting nutrient
- Limiting reactant
- Limiting reagent
- Limiting recursive
- Limiting resource
- Limiting value
- Limiting velocity
- Limit load (physics)
- Limit (math)