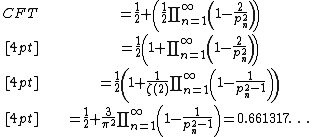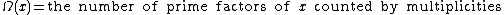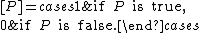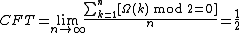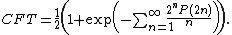“Feller–Tornier constant”的意思、由来-开放百科全书

词条

Feller–Tornier constant

释义

Omega function
Prime zeta function
See also
References

In mathematics, the Feller–Tornier constant C_FT is the density of the set of all integers that have an even number of prime factors (counted by multiplicities).^[1]

It is named after William Feller (1906–1970) and Erhard Tornier (1894–1982)^[2]

Omega function

The Omega function is given by

The Iverson bracket is

With these notations, we have

Prime zeta function

The prime zeta function P is give by

The Feller–Tornier constant satisfies

References

1. ^{{cite web|url=http://mathworld.wolfram.com/Feller-TornierConstant.html |title=Feller–Tornier Constant – from Wolfram MathWorld |website=Mathworld.wolfram.com |date=2017-03-23 |accessdate=2017-03-30}}
2. ^{{cite web|url=http://oeis.org/wiki/Feller–Tornier_constant|author=Steven R. Finch|title=Mathematical Constants. (Cf. Feller–Tornier constant.)|website=Oeis.org|accessdate=2017-03-30}}

2 : Mathematical constants|Zeta and L-functions

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。

Omega function

Prime zeta function

See also

References