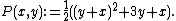“Fueter–Pólya theorem”的意思、由来-开放百科全书

词条

Fueter–Pólya theorem

释义

Introduction
Statement Proof
Fueter–Pólya conjecture
Higher dimensions
References

The Fueter–Pólya theorem, first proved by Rudolf Fueter and George Pólya, states that the only quadratic pairing functions are the Cantor polynomials.

Introduction

In 1873, Georg Cantor showed that the so-called Cantor polynomial^[1]

is a bijective mapping from to .

The polynomial given by swapping the variables is also a pairing function.

Fueter was investigating whether there are other quadratic polynomials with this property, and concluded that this is not the case assuming . He then wrote to Pólya, who showed the theorem does not require this condition.^[2]

Statement

If is a real quadratic polynomial in two variables whose restriction to is a bijection from to then it is

Proof

The original proof is surprisingly difficult, using the Lindemann–Weierstrass theorem to prove the transcendence of

for a nonzero algebraic number

.^[3]

In 2002, M. A. Vsemirnov published an elementary proof of this result.^[4]

Fueter–Pólya conjecture

The theorem states that the Cantor polynomial is the only quadratic paring polynomial of and . The Cantor polynomial can be generalized to higher degree as bijection of ℕ^k with ℕ for k > 2. The conjecture is that these are the only such pairing polynomials.

Higher dimensions

The generalization of the Cantor polynomial in higher dimensions is as follows:^[5]

The sum of these binomial coefficients yields a polynomial of degree in variables. It is an open question whether every degree polynomial which is a bijection arises as a permutation of the variables of the polynomial 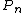.^[6]

References

1. ^G. Cantor: Ein Beitrag zur Mannigfaltigkeitslehre, J. Reine Angew. Math., Band 84 (1878), Pages 242–258
2. ^Rudolf Fueter, Georg Pólya: Rationale Abzählung der Gitterpunkte, Vierteljschr. Naturforsch. Ges. Zürich 58 (1923), Pages 280–386
3. ^Craig Smoryński: Logical Number Theory I, Springer-Verlag 1991, {{ISBN|3-540-52236-0}}, Chapters I.4 and I.5: The Fueter–Pólya Theorem I/II
4. ^M. A. Vsemirnov, Two elementary proofs of the Fueter–Pólya theorem on pairing polynomials.St. Petersburg Math. J. 13 (2002), no. 5, pp. 705–715. Correction: ibid. 14 (2003), no. 5, p. 887.
5. ^P. Chowla: On some Polynomials which represent every natural number exactly once, Norske Vid. Selsk. Forh. Trondheim (1961), volume 34, pages 8–9
6. ^Craig Smoryński: Logical Number Theory I, Springer-Verlag 1991, {{ISBN|3-540-52236-0}}, Chapter I.4, Conjecture 4.3

2 : Mathematical theorems|Number theory

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。