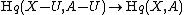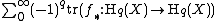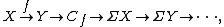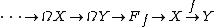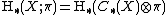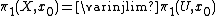“Glossary of algebraic topology”的意思、由来-开放百科全书

词条

Glossary of algebraic topology

释义

!$@
A
B
C
D
E
F
G
H
I
J
K
L
M
N
O
P
Q
R
S
T
U
V
W
Notes
References
External links

This is a glossary of properties and concepts in algebraic topology in mathematics.

See also: glossary of topology, list of algebraic topology topics, glossary of category theory, glossary of differential geometry and topology, Timeline of manifolds.

Convention: Throughout the article, I denotes the unit inverval, Sⁿ the n-sphere and Dⁿ the n-disk. Also, throughout the article, spaces are assumed to be reasonable; this can be taken to mean for example, a space is a CW complex or compactly generated weakly Hausdorff space. Similarly, no attempt is made to be definitive about the definition of a spectrum. A simplicial set is not thought of as a space; i.e., we generally distinguish between simplicial sets and their geometric realizations.
Inclusion criterion: As there is no glossary of homological algebra in Wikipedia right now, this glossary also includes some few concepts in homological algebra (e.g., chain homotopy); some concepts in geometric topology are also fair game. On the other hand, the items that appear in glossary of topology are generally omitted. Abstract homotopy theory and motivic homotopy theory are also outside the scope. Glossary of category theory covers (or will cover) concepts in theory of model categories.

!$@

{{glossary}}{{term|*}}{{defn|1=The base point of a based space.}}{{term|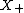

}}{{defn|1=For an unbased space X, X₊ is the based space obtained by adjoining a disjoint base point.}}{{glossary end}}

A

{{glossary}}{{term|absolute neighborhood retract}}{{term|abstract}}{{defn|no=1|Abstract homotopy theory}}{{term|Adams}}{{defn|no=1|1=John Frank Adams.}}{{defn|no=2|1=The Adams spectral sequence.}}{{defn|no=3|1=The Adams conjecture.}}{{defn|no=4|1=The Adams e-invariant.}}{{defn|no=5|1=The Adams operations.}}{{term|Alexander duality}}{{term|Alexander trick}}{{defn|The Alexander trick produces a section of the restriction map

, Top denoting a homeomorphism group; namely, the section is given by sending a homeomorphism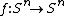

to the homeomorphism

.

This section is in fact a homotopy inverse.^[1]}}

{{term|Analysis Situs}}{{term|aspherical space}}{{term|assembly map}}{{term|Atiyah}}{{defn|no=1|1=Michael Atiyah.}}{{defn|no=2|1=Atiyah duality.}}{{defn|no=3|1=The Atiyah–Hirzebruch spectral sequence.}}{{glossary end}}

B

{{glossary}}{{term|bar construction}}{{term|based space}}{{defn|1=A pair (X, x₀) consisting of a space X and a point x₀ in X.}}{{term|Betti number}}{{term|Bockstein homomorphism}}{{term|Borel–Moore homology}}{{term|Borsuk's theorem}}{{term|Bott}}{{defn|no=1|Raoul Bott.}}{{defn|no=2|The Bott periodicity theorem for unitary groups say: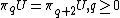

.}}{{defn|no=3|The Bott periodicity theorem for orthogonal groups say:

.}}{{term|Brouwer fixed point theorem}}{{defn|1=The Brouwer fixed-point theorem says that any map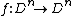

has a fixed point.}}{{glossary end}}

C

{{glossary}}{{term|cap product}}{{term|Čech cohomology}}{{term|cellular}}{{defn|no=1|A map ƒ:X→Y between CW complexes is cellular if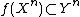

for all n.}}{{defn|no=2|The cellular approximation theorem says that every map between CW complexes is homotopic to a cellular map between them.}}{{defn|no=3|The cellular homology is the (canonical) homology of a CW complex. Note it applies to CW complexes and not to spaces in general. A cellular homology is highly computable; it is especially useful for spaces with natural cell decompositions like projective spaces or Grassmannian.}}{{term|chain homotopy}}{{defn|1=Given chain maps

between chain complexes of modules, a chain homotopy s from f to g is a sequence of module homomorphisms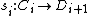

satisfying

.}}{{term|chain map}}{{defn|1=A chain map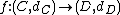

between chain complexes of modules is a sequence of module homomorphisms

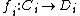that commutes with the differentials; i.e.,

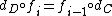.}}{{term|chain homotopy equivalence}}{{defn|1=A chain map that is an isomorphism up to chain homotopy; that is, if ƒ:C→D is a chain map, then it is a chain homotopy equivalence if there is a chain map g:D→C such that gƒ and ƒg are chain homotopic to the identity homomorphisms on C and D, respectively.}}{{term|change of fiber}}{{defn|The change of fiber of a fibration p is a homotopy equivalence, up to homotopy, between the fibers of p induced by a path in the base.}}{{term|character variety}}{{defn|1=The character variety^[2] of a group π and an algebraic group G (e.g., a reductive complex Lie group) is the geometric invariant theory quotient by G:

.}}

{{term|characteristic class}}{{defn|1=Let Vect(X) be the set of isomorphism classes of vector bundles on X. We can view

as a contravariant functor from Top to Set by sending a map ƒ:X → Y to the pullback ƒ^* along it. Then a characteristic class is a natural transformation from Vect to the cohomology functor H^*. Explicitly, to each vector bundle E we assign a cohomology class, say, c(E). The assignment is natural in the sense that ƒ^*c(E) = c(ƒ^*E).}}{{term|chromatic homotopy theory}}{{defn|1=chromatic homotopy theory.}}{{term|class}}{{defn|no=1|Chern class.}}{{defn|no=2|Stiefel–Whitney class.}}{{term|classifying space}}{{defn|1=Loosely speaking, a classifying space is a space representing some contravariant functor defined on the category of spaces; for example,

is the classifying space in the sense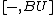

is the functor

that sends a space to the set of isomorphism classes of real vector bundles on the space.}}{{term|clutching}}{{term|cobar spectral sequence}}{{term|cobordism}}{{defn|no=1|1=See cobordism.}}{{defn|no=2|1=A cobordism ring is a ring whose elements are cobordism classes.}}{{defn|no=3|See also h-cobordism theorem, s-cobordism theorem.}}{{term|coefficient ring}}{{defn|1=If E is a ring spectrum, then the coefficient ring of it is the ring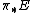

.}}{{term|cofiber sequence}}{{defn|1=A cofiber sequence is any sequence that is equivalent to the sequence

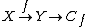for some ƒ where

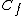is the reduced mapping cone of ƒ (called the cofiber of ƒ).}}{{term|cofibrant approximation}}{{term|cofibration}}{{defn|1=A map

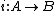is a cofibration if it satisfies the property: given

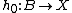and homotopy

such that

, there is a homotopy

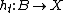such that

.^[3] A cofibration is injective and is a homeomorphism onto its image.}}{{term|coherent homotopy}}{{term|cohomotopy group}}{{defn|1=For a based space X, the set of homotopy classes

is called the n-th cohomotopy group of X.}}{{term|cohomology operation}}{{term|completion}}{{term|complex bordism}}{{term|complex-oriented}}{{defn|1=A multiplicative cohomology theory E is complex-oriented if the restriction map E²(CP^∞) → E²(CP¹) is surjective.}}{{term|cone}}{{defn|1=The cone over a space X is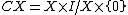

. The reduced cone is obtained from the reduced cylinder

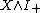by collapsing the top.}}{{term|connective}}{{defn|A spectrum E is connective if

for all negative integers q.}}{{term|configuration space}}{{term|1=constant}}{{defn|1=A constant sheaf on a space X is a sheaf

on X such that for some set A and some map

, the natural map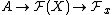

is bijective for any x in X.}}{{term|contractible space}}{{defn|1=A space is contractible if the identity map on the space is homotopic to the constant map.}}{{term|covering}}{{defn|no=1|A map p: Y → X is a covering or a covering map if each point of x has a neighborhood N that is evenly covered by p; this means that the pre-image of N is a disjoint union of open sets, each of which maps to N homeomorphically.}}{{defn|no=2|It is n-sheeted if each fiber p⁻¹(x) has exactly n elements.}}{{defn|no=3|It is universal if Y is simply connected.}}{{defn|no=4|A morphism of a covering is a map over X. In particular, an automorphism of a covering p:Y→X (also called a deck transformation) is a map Y→Y over X that has inverse; i.e., a homeomorphism over X.}}{{defn|no=5|A G-covering is a covering arising from a group action on a space X by a group G, the covering map being the quotient map from X to the orbit space X/G. The notion is used to state the universal property: if X admits a universal covering (in particular connected), then

is the set of isomorphism classes of G-coverings.

In particular, if G is abelian, then the left-hand side is (cf. nonabelian cohomology.)}}

{{term|cup product}}{{term|CW complex}}{{defn|1=A CW complex is a space X equipped with a CW structure; i.e., a filtration

such that (1) X⁰ is discrete and (2) Xⁿ is obtained from X^n-1 by attaching n-cells.}}

D

{{glossary}}{{term|deck transformation}}{{defn|Another term for an automorphism of a covering.}}{{term|delooping}}{{term|degeneracy cycle}}{{term|degree}}{{glossary end}}

E

{{glossary}}{{term|Eckmann–Hilton argument}}{{defn|1=The Eckmann–Hilton argument.}}{{term|Eckmann–Hilton duality}}{{term|Eilenberg–MacLane spaces}}{{defn|1=Given an abelian group π, the Eilenberg–MacLane spaces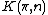

are characterized by

.}}

{{term|Eilenberg–Steenrod axioms}}{{defn|1=The Eilenberg–Steenrod axioms are the set of axioms that any cohomology theory (singular, cellular, etc.) must satisfy. Weakening the axioms (namely dropping the dimension axiom) leads to a generalized cohomology theory.}}{{term|Eilenberg–Zilber theorem}}{{term|1=E_n-algebra|2=E_n-algebra}}{{term|equivariant algebraic topology}}{{defn|1=Equivariant algebraic topoloy is the study of spaces with (continuous) group action.}}{{term|exact}}{{defn|1=A sequence of pointed sets

is exact if the image of f coincides with the pre-image of the chosen point of Z.}}{{term|excision}}{{defn|1=The excision axiom for homology says: if

and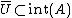

, then for each q,

is an isomorphism.}}

F

{{glossary}}{{term|factorization homology}}{{term|fiber-homotopy equivalence}}{{defn|1=Given D→B, E→B, a map ƒ:D→E over B is a fiber-homotopy equivalence if it is invertible up to homotopy over B. The basic fact is that if D→B, E→B are fibrations, then a homotopy equivalence from D to E is a fiber-homotopy equivalence.}}{{term|fibration}}{{defn|1=A map p:E → B is a fibration if for any given homotopy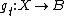

and a map

such that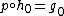

, there exists a homotopy

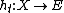such that

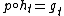. (The above property is called the homotopy lifting property.) A covering map is a basic example of a fibration.}}{{term|fibration sequence}}{{defn|1=One says

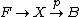is a fibration sequence to mean that p is a fibration and that F is homotopy equivalent to the homotopy fiber of p, with some understanding of base points.}}{{term|finitely dominated}}{{term|fundamental class}}{{term|fundamental group}}{{defn|1=The fundamental group of a space X with base point x₀ is the group of homotopy classes of loops at x₀. It is precisely the first homotopy group of (X, x₀) and is thus denoted by

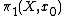.}}{{term|fundamental groupoid}}{{defn|1=The fundamental groupoid of a space X is the category whose objects are the points of X and whose morphisms x → y are the homotopy classes of paths from x to y; thus, the set of all morphisms from an object x₀ to itself is, by definition, the fundament group

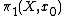.}}{{term|free}}{{defn|1=Synonymous with unbased. For example, the free path space of a space X refers to the space of all maps from I to X; i.e.,

while the path space of a based space X consists of such map that preserve the base point (i.e., 0 goes to the base point of X).}}{{term|Freudenthal suspension theorem}}{{defn|1=For a nondegenerately based space X, the Freudenthal suspension theorem says: if X is (n-1)-connected, then the suspension homomorphism

is bijective for q < 2n - 1 and is surjective if q = 2n - 1.}}

G

{{glossary}}{{term|G-fibration}}{{defn|1=A G-fibration with some topological monoid G. An example is Moore's path space fibration.}}{{term|Γ-space}}{{term|generalized cohomology theory}}{{defn|1=A generalized cohomology theory is a contravariant functor from the category of pairs of spaces to the category of abelian groups that satisfies all of the Eilenberg–Steenrod axioms except the dimension axiom.}}{{term|genus}}{{term|group completion}}{{term|grouplike}}{{defn|1=An H-space X is said to be group-like or grouplike if

is a group; i.e., X satisfies the group axioms up to homotopy.}}{{term|Gysin sequence}}{{glossary end}}

H

{{glossary}}{{term|Hilton–Milnor theorem}}{{defn|1=The Hilton–Milnor theorem.}}{{term|H-space}}{{defn|1=An H-space is a based space that is a unital magma up to homotopy.}}{{term|homologus}}{{defn|1=Two cycles are homologus if they belong to the same homology class.}}{{term|homotopy category}}{{defn|1=Let C be a subcategory of the category of all spaces. Then the homotopy category of C is the category whose class of objects is the same as the class of objects of C but the set of morphisms from an object x to an object y is the set of the homotopy classes of morphisms from x to y in C. For example, a map is a homotopy equivalence if and only if it is an isomorphism in the homotopy category.}}{{term|homotopy colimit}}{{term|homotopy over a space B}}{{defn|A homotopy h_t such that for each fixed t, h_t is a map over B.}}{{term|homotopy equivalence}}{{defn|no=1|A map ƒ:X→Y is a homotopy equivalence if it is invertible up to homotopy; that is, there exists a map g: Y→X such that g ∘ ƒ is homotopic to th identity map on X and ƒ ∘ g is homotopic to the identity map on Y.}}{{defn|no=2|Two spaces are said to be homotopy equivalent if there is a homotopy equivalence between the two. For example, by definition, a space is contractible if it is homotopy equivalent to a point space.}}{{term|homotopy excision theorem}}{{defn|1=The homotopy excision theorem is a substitute for the failure of excision for homotopy groups.}}{{term|homotopy fiber}}{{defn|1=The homotopy fiber of a based map ƒ:X→Y, denoted by Fƒ, is the pullback of

along f.}}{{term|homotopy fiber product}}{{defn|1=A fiber product is a particular kind of a limit. Replacing this limit lim with a homotopy limit holim yields a homotopy fiber product.}}{{term|homotopy group}}{{defn|no=1|For a based space X, let

, the set of homotopy classes of based maps. Then

is the set of path-connected components of X,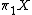

is the fundamental group of X and

are the (higher) n-th homotopy groups of X.}}{{defn|no=2|For based spaces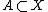

, the relative homotopy group

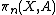is defined as

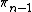of the space of paths that all start at the base point of X and end somewhere in A. Equivalently, it is the

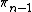of the homotopy fiber of

.}}{{defn|no=3|If E is a spectrum, then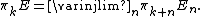

}}{{defn|no=4|If X is a based space, then the stable k-th homotopy group of X is

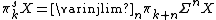. In other words, it is the k-th homotopy group of the suspension spectrum of X.}}{{term|homotopy quotient}}{{defn|1=If G is a Lie group acting on a manifold X, then the quotient space

is called the homotopy quotient (or Borel construction) of X by G, where EG is the universal bundle of G.}}{{term|homotopy spectral sequence}}{{term|homotopy sphere}}{{term|Hopf}}{{defn|no=1|1=Heinz Hopf.}}{{defn|no=2|1=Hopf invariant.}}{{defn|no=3|1=The Hopf index theorem.}}{{defn|no=4|1=Hopf construction.}}{{term|Hurewicz}}{{defn|1=The Hurewicz theorem establishes a relationship between homotopy groups and homology groups.}}{{glossary end}}

I

{{glossary}}{{term|infinite loop space}}{{term|infinite loop space machine}}{{term|infinite mapping telescope}}{{term|integration along the fiber}}{{term|isotopy}}{{glossary end}}

J

{{glossary}}{{term|J-homomorphism}}{{defn|See J-homomorphism.}}{{term|join}}{{defn|The join of based spaces X, Y is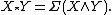

}}{{glossary end}}

K

{{glossary}}{{term|k-invariant}}{{term|Kan complex}}{{defn|See Kan complex.}}{{term|Kervaire invariant}}{{defn|1=The Kervaire invariant.}}{{term|Koszul duality}}{{defn|1=Koszul duality.}}{{term|Künneth formula}}{{glossary end}}

L

{{glossary}}{{term|Lazard ring}}{{defn|1=The Lazard ring L is the (huge) commutative ring together with the formal group law ƒ that is universal among all the formal group laws in the sense that any formal group law g over a commutative ring R is obtained via a ring homomorphism L → R mapping ƒ to g. According to Quillen's theorem, it is also the coefficient ring of the complex bordism MU. The Spec of L is called the moduli space of formal group laws.}}{{term|Lefschetz fixed point theorem}}{{defn|1=The Lefschetz fixed point theorem says: given a finite simplicial complex K and its geometric realization X, if a map

has no fixed point, then the Lefschetz number of f; that is,

is zero. For example, it implies the Brouwer fixed-point theorem since the Lefschetz number of 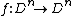is, as higher homologies vanish, one.}}

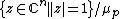{{term|lens space}}{{defn|1=The lens space is the quotient space

where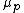

is the group of p-th roots of unity acting on the unit sphere by

.}}{{term|Leray spectral sequence}}{{term|local coefficient}}{{defn|no=1|1=A module over the group ring

for some based space B; in other words, an abelian group together with a homomorphism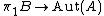

.}}{{defn|no=2|1=The local coefficient system over a based space B with an abelian group A is a fiber bundle over B with discrete fiber A. If B admits a universal covering

, then this meaning coincides with that of 1. in the sense: every local coefficient system over B can be given as the associated bundle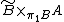

.}}{{term|local sphere}}{{defn|1=The localization of a sphere at some prime number}}{{term|localization}}{{term|locally constant sheaf}}{{defn|1=A locally constant sheaf on a space X is a sheaf such that each point of X has an open neighborhood on which the sheaf is constant.}}{{term|loop space}}{{defn|The loop space

of a based space X is the space of all loops starting and ending at the base point of X.}}{{glossary end}}

M

{{glossary}}{{term|Madsen–Weiss theorem}}{{term|mapping}}{{defn|no=1|1=The mapping cone (or cofiber) of a map ƒ:X→Y is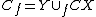

.}}{{defn|no=2|1=The mapping cylinder of a map ƒ:X→Y is

. Note:

.}}{{defn|no=3|1=The reduced versions of the above are obtained by using reduced cone and reduced cylinder.}}{{defn|no=4|1=The mapping path space P_p of a map p:E→B is the pullback of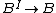

along p. If p is fibration, then the natural map E→P_p is a fiber-homotopy equivalence; thus, roughly speaking, one can replace E by the mapping path space without changing the homotopy type of the fiber.}}{{term|Mayer–Vietoris sequence}}{{term|model category}}{{defn|1=A presentation of an ∞-category.^[4] See also model category.}}{{term|Moore space}}{{term|multiplicative}}{{defn|1=A generalized cohomology theory E is multiplicative if E^*(X) is a graded ring. For example, the ordinary cohomology theory and the complex K-theory are multiplicative (in fact, cohomology theories defined by E_∞-rings are multiplicative.) }}{{glossary end}}

N

{{glossary}}{{term|n-cell}}{{defn|1=Another term for an n-disk.}}{{term|n-connected}}{{defn|1=A based space X is n-connected if

for all integers q ≤ n. For example, "1-connected" is the same thing as "simply connected".}}{{term|n-equivalent}}{{term|NDR-pair}}{{defn|1=A pair of spaces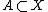

is said to be an NDR-pair (=neighborhood deformation retract pair) if there is a map

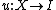and a homotopy

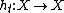such that

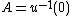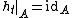and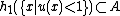

If A is a closed subspace of X, then the pair 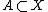is an NDR-pair if and only if is a cofibration.

}}

{{term|nilpotent}}{{defn|no=1|1=nilpotent space; for example, a simply connected space is nilpotent.}}{{defn|no=2|1=The nilpotent theorem.}}{{term|normalized}}{{defn|1=Given a simplicial group G, the normalized chain complex NG of G is given by

with the n-th differential given by

; intuitively, one throws out degenerate chains.^[5] It is also called the Moore complex.}}{{glossary end}}

O

{{glossary}}{{term|obstruction cocycle}}{{term|obstruction theory}}{{defn|1=Obstruction theory is the collection of constructions and calculations indicating when some map on a submanifold (subcomplex) can or cannot be extended to the full manifold. These typically involve the Postnikov tower, killing homotopy groups, obstruction cocycles, etc.}}{{term|of finite type}}{{defn|1=A CW complex is of finite type if there are only finitely many cells in each dimension.}}{{term|operad}}{{defn|1=The portmanteau of “operations” and “monad”. See operad.}}{{term|orbit category}}{{term|orientation}}{{defn|no=1|1=The orientation covering (or orientation double cover) of a manifold is a two-sheeted covering so that each fiber over x corresponds to two different ways of orienting a neighborhood of x.}}{{defn|no=2|1=An orientation of a manifold is a section of an orientation covering; i.e., a consistent choice of a point in each fiber.}}{{defn|no=3|1=An orientation character (also called the first Stiefel–Whitney class) is a group homomorphism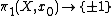

that corresponds to an orientation covering of a manifold X (cf. #covering.)}}{{defn|no=4|1=See also orientation of a vector bundle as well as orientation sheaf.}}{{glossary end}}

P

{{glossary}}{{term|p-adic homotopy theory}}{{defn|1=The p-adic homotopy theory.}}{{term|path class}}{{defn|An equivalence class of paths (two paths are equivalent if they are homotopic to each other).}}{{term|path lifting}}{{defn|1=A path lifting function for a map p: E → B is a section of

where

is the mapping path space of p. For example, a covering is a fibration with a unique path lifting function. By formal consideration, a map is a fibration if and only if there is a path lifting function for it.}}{{term|path space}}{{defn|1=The path space of a based space X is

, the space of based maps, where the base point of I is 0. Put in another way, it is the (set-theoretic) fiber of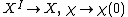

over the base point of X. The projection

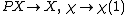is called the path space fibration, whose fiber over the base point of X is the loop space

. See also mapping path space.}}{{term|phantom map}}{{term|Poincaré}}{{defn|1=The Poincaré duality theorem says: given a manifold M of dimension n and an abelian group A, there is a natural isomorphism

.}}

{{term|Pontrjagin–Thom construction}}{{term|Postnikov system}}{{defn|1=A Postnikov system is a sequence of fibrations, such that all preceding manifolds have vanishing homotopy groups below a given dimension.}}{{term|principal fibration}}{{defn|1=Usually synonymous with G-fibration.}}{{term|profinite}}{{defn|1=profinite homotopy theory; it studies profinite spaces.}}{{term|properly discontinuous}}{{defn|Not particularly a precise term. But it could mean, for example, that G is discrete and each point of the G-space has a neighborhood V such that for each g in G that is not the identity element, gV intersects V at finitely many points.}}{{term|pullback}}{{defn|1=Given a map p:E→B, the pullback of p along ƒ:X→B is the space

(succinctly it is the equalizer of p and f). It is a space over X through a projection.}}{{term|Puppe sequence}}{{defn|1=The Puppe sequence refers ro either of the sequences

where are homotopy cofiber and homotopy fiber of f.}}

{{term|pushout}}{{defn|1=Given

and a map

, the pushout of X and B along f is

;

that is X and B are glued together along A through f. The map f is usually called the attaching map.

The important example is when B = Dⁿ, A = S^n-1; in that case, forming such a pushout is called attaching an n-cell (meaning an n-disk) to X.}}

Q

{{glossary}}{{term|quasi-fibration}}{{defn|1=A quasi-fibration is a map such that the fibers are homotopy equivalent to each other.}}{{term|Quillen}}{{defn|no=1|1=Daniel Quillen}}{{defn|no=2|1=Quillen’s theorem says that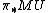

is the Lazard ring.}}{{glossary end}}

R

{{glossary}}{{term|rational}}{{defn|no=1|1=The rational homotopy theory.}}{{defn|no=2|1=The rationalization of a space X is, roughly, the localization of X at zero. More precisely, X₀ together with j: X → X₀ is a rationalization of X if the map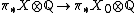

induced by j is an isomorphism of vector spaces and

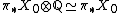.}}{{defn|no=3|1=The rational homotopy type of X is the weak homotopy type of X₀.}}{{term|Reidemeister}}{{defn|1=Reidemeister torsion.}}{{term|reduced}}{{defn|1=The reduced suspension of a based space X is the smash product

. It is related to the loop functor by

where

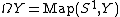is the loop space.}}{{term|ring spectrum}}{{defn|1=A ring spectrum is a spectrum that satisfying the ring axioms, either on nose or up to homotopy. For example, a complex K-theory is a ring spectrum.}}{{glossary end}}

S

{{glossary}}{{term|Samelson product}}{{term|Serre}}{{defn|no=1|1=Jean-Pierre Serre.}}{{defn|no=2|1=Serre class.}}{{defn|no=3|1=Serre spectral sequence.}}{{term|simple}}{{term|simple-homotopy equivalence}}{{defn|A map ƒ:X→Y between finite simplicial complexes (e.g., manifolds) is a simple-homotopy equivalence if it is homotopic to a composition of finitely many elementary expansions and elementary collapses. A homotopy equivalence is a simple-homotopy equivalence if and only if its Whitehead torsion vanishes.}}{{term|simplicial approximation}}{{defn|1=See simplicial approximation theorem.}}{{term|simplicial complex}}{{defn|1=See simplicial complex; the basic example is a triangulation of a manifold.}}{{term|simplicial homology}}{{defn|1=A simplicial homology is the (canonical) homology of a simplicial complex. Note it applies to simplicial complexes and not to spaces; cf. #singular homology.}}{{term|signature invariant}}{{term|singular}}{{defn|no=1|1=Given a space X and an abelian group π, the singular homology group of X with coefficients in π is

where 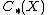is the singular chain complex of X; i.e., the n-th degree piece is the free abelian group generated by all the maps 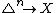from the standard n-simplex to X. A singular homology is a special case of a simplicial homology; indeed, for each space X, there is the singular simplicial complex of X ^[6] whose homology is the singular homology of X.}}

{{defn|no=2|The singular simplices functor is the functor

from the category of all spaces to the category of simplicial sets, that is the right adjoint to the geometric realization functor.}}{{defn|no=3|The singular simplicial complex of a space X is the normalized chain complex of the singular simplex of X.}}{{term|slant product}}{{term|small object argument}}{{term|smash product}}{{defn|1=The smash product of based spaces X, Y is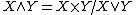

. It is characterized by the adjoint relation

.}}

{{term|Spanier–Whitehead}}{{defn|1=The Spanier–Whitehead duality.}}{{term|spectrum}}{{defn|1=Roughly a sequence of spaces together with the maps (called the structure maps) between the consecutive terms; see spectrum (topology).}}{{term|sphere bundle}}{{defn|1=A sphere bundle is a fiber bundle whose fibers are spheres.}}{{term|sphere spectrum}}{{defn|The sphere spectrum is a spectrum consisting of a sequence of spheres

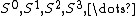together with the maps between the spheres given by suspensions. In short, it is the suspension spectrum of

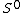.}}{{term|stable homotopy group}}{{defn|1=See #homotopy group.}}{{term|Steenrod homology}}{{defn|1=Steenrod homology.}}{{term|Steenrod operation}}{{term|Sullivan}}{{defn|no=1|1=Dennis Sullivan.}}{{defn|no=2|1=The Sullivan conjecture.}}{{defn|no=3|1={{citation| title=Infinitesimal computations in topology|year=1977|url=http://www.numdam.org/item?id=PMIHES_1977__47__269_0}} - introduces rational homotopy theory (along with Quillen's paper).}}{{defn|no=4|The Sullivan algebra in the rational homotopy theory.}}{{term|suspension spectrum}}{{defn|The suspension spectrum of a based space X is the spectrum given by

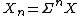.}}{{term|symmetric spectrum}}{{defn|1=See symmetric spectrum.}}{{glossary end}}

T

{{glossary}}{{term|Thom}}{{defn|no=1|1=René Thom.}}{{defn|no=2|1=If E is a vector bundle on a paracompact space X, then the Thom space

of E is obtained by first replacing each fiber by its compactification and then collapsing the base X.}}{{defn|no=3|1=The Thom isomorphism says: for each orientable vector bundle E of rank n on a manifold X, a choice of an orientation (the Thom class of E) induces an isomorphism

.}}

U

{{glossary}}{{term|universal coefficient}}{{defn|1=The universal coefficient theorem.}}{{term|up to homotopy}}{{defn|1=A statement holds in the homotopy category as opposed to the category of spaces.}}{{glossary end}}

V

{{glossary}}{{term|van Kampen}}{{defn|1=The van Kampen theorem says: if a space X is path-connected and if x₀ is a point in X, then

where the colimit runs over some open cover of X consisting of path-connected open subsets containing x₀ such that the cover is closed under finite intersections.}}

W

{{glossary}}{{term|Waldhausen S-construction}}{{defn|1=Waldhausen S-construction.}}{{term|Wall's finiteness obstruction}}{{term|weak equivalence}}{{defn|1=A map ƒ:X→Y of based spaces is a weak equivalence if for each q, the induced map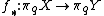

is bijective.}}{{term|wedge}}{{defn|1=For based spaces X, Y, the wedge product

of X and Y is the coproduct of X and Y; concretely, it is obtained by taking their disjoint union and then identifying the respective base points.}}{{term|well pointed}}{{defn|1=A based space is well pointed (or non-degenerately based) if the inclusion of the base point is a cofibration.}}{{term|Whitehead}}{{defn|no=1|J. H. C. Whitehead.}}{{defn|no=2|Whitehead's theorem says that for CW complexes, the homotopy equivalence is the same thing as the weak equivalence.}}{{defn|no=3|Whitehead group.}}{{defn|no=4|Whitehead product.}}{{term|winding number}}{{glossary end}}

Notes

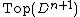1. ^Let r, s denote the restriction and the section. For each f in

, define

. Then

.
2. ^Despite the name, it may not be an algebraic variety in the strict sense; for example, it may not be irreducible. Also, without some finiteness assumption on G, it is only a scheme.
3. ^{{harvnb|Hatcher|loc=Ch. 4. H.}}
4. ^http://mathoverflow.net/questions/2185/how-to-think-about-model-categories
5. ^https://ncatlab.org/nlab/show/Moore+complex
6. ^http://ncatlab.org/nlab/show/singular+simplicial+complex

References

J.F. Adams, Stable Homotopy and Generalized Homology, Chicago Lectures in Mathematics, The University of Chicago Press, 1974.
Adams, J. (1978), Infinite loop spaces, Princeton University Press, Princeton, New Jersey.
{{citation | last1 = Bott | first1 = Raoul | authorlink = Raoul Bott | last2=Tu |first2= Loring | title = Differential Forms in Algebraic Topology | year = 1982 | publisher = Springer | location = New York | isbn = 0-387-90613-4}}
{{citation|title=Homotopy Limits, Completions and Localizations|volume=304|series=Lecture Notes in Mathematics|first1=A. K.|last1=Bousfield|first2=D. M.|last2=Kan|publisher=Springer|year=1987|isbn=9783540061052}}
James F. Davis, Paul Kirk, Lecture Notes in Algebraic Topology
Fulton, William, Algebraic Topology
Allen Hatcher, Algebraic topology.
{{Cite journal|title = Rational homotopy theory: a brief introduction|journal = |arxiv = math/0604626|date = 2006-04-28|first = Kathryn|last = Hess|bibcode = 2006math......4626H}}
[https://math.berkeley.edu/~amathew/ATnotes.pdf algebraic topology], Lectures delivered by Michael Hopkins and Notes by Akhil Mathew, Fall 2010, Harvard
Lurie, J. Algebraic K-Theory and Manifold Topology (Math 281)
Lurie, J. Chromatic Homotopy Theory (252x)
May, J. A Concise Course in Algebraic Topology
May, J. (with K. Ponto) More concise algebraic topology: localization, completion, and model categories
May and Sigurdsson, Parametrized homotopy theory (despite the title, it contains a significant amount of general results.)
Dennis Sullivan, Geometric Topology, the 1970 MIT notes
{{cite book|author=George William Whitehead|authorlink=George W. Whitehead|title=Elements of homotopy theory|url=https://books.google.com/books?id=wlrvAAAAMAAJ|accessdate=September 6, 2011|edition=3rd|series=Graduate Texts in Mathematics|volume=61|year=1978|publisher=Springer-Verlag|location=New York-Berlin|isbn=978-0-387-90336-1|pages=xxi+744|mr=0516508 }}
Kirsten Graham Wickelgren, 8803 Stable Homotopy Theory

External links

Algebraic Toplogy: A guide to literature

2 : Algebraic topology|Glossaries of mathematics

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。