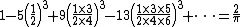“Gustav Conrad Bauer”的意思、由来-开放百科全书

Gustav Conrad Bauer (18 November 1820, Augsburg – 3 April 1906, Munich) was a German mathematician,^[1] known for the Bauer-Muir transformation^[2]^[3] and Bauer's conic sections. He earned a footnote in the history of science as the doctoral advisor (Doktorvater) of Heinrich Burkhardt, who became one of the two referees of Albert Einstein's doctoral dissertation.

Education and family

Gustav Bauer passed in 1837 his Abitur at Augsburg's Gymnasium bei St. Anna. He continued his studies of mathematics at the Polytechnischen Schule Augsburg and also the universities of Erlangen, Vienna and Berlin. At Humboldt University in Berlin, Bauer received in 1842 his Promotierung under Peter Gustav Lejeune Dirichlet. From 1842 Gustav Bauer continued his studies in Paris under Joseph Liouville, as well as other mathematicians.

In 1862 Gustav Bauer married Amalie, daughter of the Archivrat and Professor Honorarius Nathanael von Schlichtegroll. The marriage produced two daughters and a son Gustav junior, who became a well-known engineer.

Professional career

At the beginning of his professional employment, Bauer applied for a civil service position as a schoolteacher but became a private tutor from 1845 to 1853 in the royal house of Prince Mihail Sturdza and his successor Prince Grigore Alexandru Ghica in what is now Rumania. In 1857 Bauer spent three months in England and upon his return to Germany became a Privatdozent for the Mathematics Faculty of the Ludwig Maximilian University of Munich. There he received his Habilitation and became in 1865 professor extraordinarius, in 1869 professor ordinarius, and in 1900 professor emeritus.

Bauer's mathematical research dealt with algebra, geometric problems, spherical harmonics, the gamma function, and generalized continued fractions. In 1871 Bauer was elected a full member of the Bayerische Akademie der Wissenschaften. In 1884 he was elected a member of the Academy of Sciences Leopoldina. His doctoral students include Heinrich Burkhardt, Eduard Ritter von Weber, and Christian August Vogler.^[4]

Footnotes in the history of mathematics

In Ramanujan's first letter to G. H. Hardy, one of the theorems that impressed Hardy was:

However, Bauer proved the theorem in 1859.^[5]^[6] Using a result of Bauer on generalized continued fractions, Oskar Perron published in 1952 the first proof of another formula of Ramanujan.^[1]^[7]

Selected publications

Sources

References

1. ^¹{{NDB|1|638|638|Bauer, Gustav|Georg Faber|116084642}}
2. ^{{cite journal|author=Jacobsen, Lisa|title=On the Bauer-Muir transformation for continued fractions and its applications|journal=Journal of Mathematical Analysis and Applications|volume=152|issue=2|year=1990|pages=496–514|url=http://www.sciencedirect.com/science/article/pii/0022247X9090080Y|doi=10.1016/0022-247X(90)90080-Y}}
3. ^{{cite journal|author=Bauer, G.|title=Von einem Kettenbruche Euler's und einem Theorem von Wallis|journal=Abhandlungen der Mathematisch-Physikalischen Classe der Königlich Bayerische Akademie der Wissenschaften|year=1872|volume=11|pages=96–116|url=https://babel.hathitrust.org/cgi/pt?id=hvd.32044106313281;view=1up;seq=425}}
4. ^{{MathGenealogy|id=61850}}
5. ^{{cite journal|author=Bauer, G.|title=Von den Coefficienten der Reihen von Kugelfunctionen einer Variablen|journal=J. Reine Angew. Math.|year=1859|volume=56|issue=56|pages=101–121|url=https://babel.hathitrust.org/cgi/pt?id=mdp.39015013784650;view=1up;seq=109|doi=10.1515/crll.1859.56.101}}
6. ^{{cite book|author=Berndt, Bruce C.|authorlink=Bruce C. Berndt|title=Ramanujan's Notebooks, Part 2|page=24|year=1999|publisher=Springer|url=https://books.google.com/books?id=z8Kjb0_pgx8C&pg=PA24|isbn=9780387967943}}
7. ^{{cite journal|author=Perron, O.|title=Über eine Formel von Ramanujan|year=1952|journal=Sitz. Bayer. Akad. Wiss. München Math. Phys. Kl.|pages=197–213}}

Education and family

Professional career

Footnotes in the history of mathematics

Selected publications

Sources

References

External links