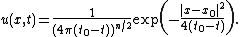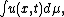“Huisken's monotonicity formula”的意思、由来-开放百科全书

In differential geometry, Huisken's monotonicity formula states that, if an {{mvar|n}}-dimensional surface in {{math|(n + 1)}}-dimensional Euclidean space undergoes the mean curvature flow, then its convolution with an appropriately scaled and time-reversed heat kernel is non-increasing.^[1]^[2] The result is named after Gerhard Huisken, who published it in 1990.^[3]

Specifically, the {{math|(n + 1)}}-dimensional time-reversed heat kernel converging to a point {{math|x₀}} at time {{math|t₀}} may be given by the formula^[1]

Then Huisken's monotonicity formula gives an explicit expression for the derivative

where {{mvar|μ}} is the area element of the evolving surface at time {{mvar|t}}. The expression involves the negation of another integral, whose integrand is non-negative, so the derivative is non-positive.

Typically, {{math|x₀}} and {{math|t₀}} are chosen as the time and position of a singularity of the evolving surface, and the monotonicity formula can be used to analyze the behavior of the surface as it evolves towards this singularity. In particular, the only surfaces for which the convolution with the heat kernel remains constant rather than decreasing are ones that stay self-similar as they evolve, and the monotonicity formula can be used to classify these surfaces.

References

1. ^¹{{citation | last = Mantegazza | first = Carlo | doi = 10.1007/978-3-0348-0145-4 | isbn = 978-3-0348-0144-7 | mr = 2815949 | contribution = 3.1 The Monotonicity Formula for Mean Curvature Flow | pages = 49–52 | publisher = Birkhäuser/Springer | location = Basel | series = Progress in Mathematics | title = Lecture notes on mean curvature flow | url = https://books.google.com/books?id=l2muz9qOAosC&pg=PA51 | volume = 290 | year = 2011| citeseerx = 10.1.1.205.9269 }}.
2. ^{{citation | last = Bellettini | first = Giovanni | contribution = 4 Huisken's monotonicity formula | doi = 10.1007/978-88-7642-429-8 | isbn = 978-88-7642-428-1 | mr = 3155251 | pages = 59–68 | publisher = Edizioni della Normale | location = Pisa | series = Appunti. Scuola Normale Superiore di Pisa (Nuova Serie) [Lecture Notes. Scuola Normale Superiore di Pisa (New Series)] | title = Lecture notes on mean curvature flow, barriers and singular perturbations | volume = 12 | year = 2013}}.
3. ^{{citation | last = Huisken | first = Gerhard | authorlink = Gerhard Huisken | issue = 1 | journal = Journal of Differential Geometry | mr = 1030675 | pages = 285–299 | title = Asymptotic behavior for singularities of the mean curvature flow | url = http://projecteuclid.org/euclid.jdg/1214444099 | volume = 31 | year = 1990| doi = 10.4310/jdg/1214444099 }}.
4. ^{{citation | last = Perelman | first = Grigori | author-link = Grigori Perelman | arxiv = math/0211159 | title = The entropy formula for the Ricci flow and its geometric applications | year = 2002| bibcode = 2002math.....11159P}}.
5. ^{{citation | last1 = Cao | first1 = Huai-Dong | last2 = Hamilton | first2 = Richard S. | last3 = Ilmanen | first3 = Tom | arxiv = math/0404165 | quotation = There are also two monotonicity formulas of shrinking or localizing type ... Either of these can be seen as the analogue of Huisken’s monotonicity formula for mean curvature flow. | title = Gaussian densities and stability for some Ricci solitons | year = 2004| bibcode = 2004math......4165C}}.