“Intensity measure”的意思、由来-开放百科全书

词条

Intensity measure

释义

Definition
Properties
References

In probability theory, an intensity measure is a measure that is derived from a random measure. The intensity measure is a non-random measure and is defined as the expectation value of the random measure of a set, hence it corresponds to the average volume the random measure assigns to a set. The intensity measure contains important information about the properties of the random measure. A Poisson point process, interpreted as a random measure, is for example uniquely determined by its intensity measure. ^[1]

Definition

Let be a random measure on the measurable space and denote the expected value of a random element with .

The intensity measure

of is defined as

for all .^[2] ^[3]

Note the difference in notation between the expectation value of a random element , denoted by and the intensity measure of the random measure , denoted by .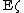

Properties

The intensity measure 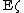is always s-finite and satisfies

for every positive measurable function on .^[3]

References

^[1]^[2]^[3]

2 : Measures (measure theory)|Probability theory

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。