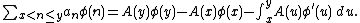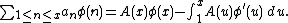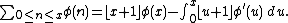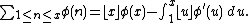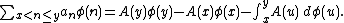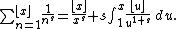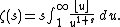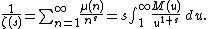- Formula Variations
- Examples Harmonic numbers Representation of Riemann's zeta function Reciprocal of Riemann zeta function
- See also
- References
In mathematics, Abel's summation formula, introduced by Niels Henrik Abel, is intensively used in number theory and the study of special functions to compute series.
Formula
Let 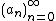 be a sequence of real or complex numbers. Define the partial sum function
be a sequence of real or complex numbers. Define the partial sum function  by
by
for any real number  . Fix real numbers
. Fix real numbers  , and let
, and let  be a continuously differentiable function on
be a continuously differentiable function on  . Then:
. Then:
The formula is derived by applying integration by parts for a Riemann–Stieltjes integral to the functions  and
and  .
.
Variations
Taking the left endpoint to be  gives the formula
gives the formula
If the sequence  is indexed starting at
is indexed starting at  , then we may formally define
, then we may formally define  . The previous formula becomes
. The previous formula becomes
A common way to apply Abel's summation formula is to take the limit of one of these formulas as  . The resulting formulas are
. The resulting formulas are
These equations hold whenever both limits on the right-hand side exist and are finite.
A particularly useful case is the sequence  for all
for all  . In this case,
. In this case, 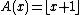 . For this sequence, Abel's summation formula simplifies to
. For this sequence, Abel's summation formula simplifies to
Similarly, for the sequence  and
and  for all
for all  , the formula becomes
, the formula becomes
Upon taking the limit as  , we find
, we find
assuming that both terms on the right-hand side exist and are finite.
Abel's summation formula can be generalized to the case where  is only assumed to be continuous if the integral is interpreted as a Riemann–Stieltjes integral:
is only assumed to be continuous if the integral is interpreted as a Riemann–Stieltjes integral:
By taking  to be the partial sum function associated to some sequence, this leads to the summation by parts formula.
to be the partial sum function associated to some sequence, this leads to the summation by parts formula.
Examples
Harmonic numbers
If  for
for  and
and  then
then 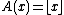 and the formula yields
and the formula yields
The left-hand side is the harmonic number 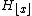 .
.
Representation of Riemann's zeta function
Fix a complex number  . If
. If  for
for  and
and 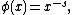 then
then 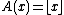 and the formula becomes
and the formula becomes
If 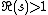 , then the limit as
, then the limit as  exists and yields the formula
exists and yields the formula
This may be used to derive Dirichlet's theorem that 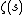 has a simple pole with residue 1 at {{math|s {{=}} 1}}.
has a simple pole with residue 1 at {{math|s {{=}} 1}}.
Reciprocal of Riemann zeta function
The technique of the previous example may also be applied to other Dirichlet series. If 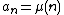 is the Möbius function and
is the Möbius function and  , then
, then 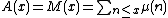 is Mertens function and
is Mertens function and
This formula holds for 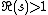 .
.
See also
- Summation by parts
References
- {{citation|first=Tom|last=Apostol|authorlink=Tom Apostol|title=Introduction to Analytic Number Theory|publisher=Springer-Verlag|series=Undergraduate Texts in Mathematics|year=1976}}.
- Cops (animated TV series)
- Cops (cartoon)
- Cops, Crooks and Civilians
- Cops & Doughnuts
- Cops episodes
- Cops Is Always Right
- Cops is Tops
- Cops LAC
- C.O.P.S. 'N' Crooks
- Copson, Andrew
- COPS (reality TV series)
- Cops (reality TV series)
- Cops & Robbers
- Copssh
- Cops Shot the Kid
- Cops Shot The Kid
- Cops (song)
- Cops Suey
- Copster Hill
- COPS (TV series)
- Cops (TV series)
- COPS (U.S. animated TV series)
- Cops (U.S. TV series)
- Cops (video game)
- Coptacra