“Operator ideal”的意思、由来-开放百科全书

词条

Operator ideal

释义

Formal definition
Properties and examples
References

In functional analysis, a branch of mathematics, an operator ideal is a special kind of class of continuous linear operators between Banach spaces. If an operator belongs to an operator ideal 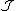, then for any operators and which can be composed with as 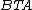, then 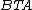is class 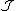as well. Additionally, in order for 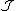to be an operator ideal, it must contain the class of all finite-rank Banach space operators.

Formal definition

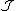Let denote the class of continuous linear operators acting between two Banach spaces. For any subclass of and any two Banach spaces and over the same field 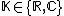, denote by 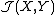the set of continuous linear operators of the form . In this case, we say that 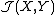is a component of 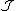. An operator ideal is a subclass 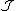of , containing every identity operator acting on a 1-dimensional Banach space, such that for any two Banach spaces and over the same field , the following two conditions for 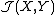are satisfied:

(1) If then ; and

(2) if and are Banach spaces over with and , and if , then .

Properties and examples

Operator ideals enjoy the following nice properties.

Every component 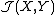of an operator ideal forms a linear subspace of , although in general this need not be norm-closed.
Every operator ideal contains all finite-rank operators. In particular, the finite-rank operators form the smallest operator ideal.
For each operator ideal 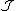, every component of the form 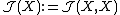forms an ideal in the algebraic sense.

Furthermore, some very well-known classes are norm-closed operator ideals, i.e., operator ideals whose components are always norm-closed. These include but are not limited to the following.

Compact operators
Weakly compact operators
Finitely strictly singular operators
Strictly singular operators
Completely continuous operators

References

Pietsch, Albrecht: Operator Ideals, Volume 16 of Mathematische Monographien, Deutscher Verlag d. Wiss., VEB, 1978.

1 : Functional analysis

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。