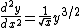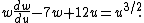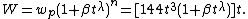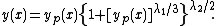- Transformations
- Sommerfeld's approximation
- Solution near origin
- Approach by Majorana
- References
In mathematics, the Thomas–Fermi equation for the neutral atom is a second order non-linear ordinary differential equation, named after Llewellyn Thomas and Enrico Fermi,[1][2] which can be derived by applying the Thomas–Fermi model to atoms. The equation reads
subject to the boundary conditions
If  approaches zero as
approaches zero as  becomes large, this equation models the charge distribution of a neutral atom as a function of radius
becomes large, this equation models the charge distribution of a neutral atom as a function of radius  . Solutions where
. Solutions where  becomes zero at finite
becomes zero at finite  model positive ions.[3] For solutions where
model positive ions.[3] For solutions where  becomes large and positive as
becomes large and positive as  becomes large, it can be interpreted as a model of a compressed atom, where the charge is squeezed into a smaller space. In this case the atom ends at the value of
becomes large, it can be interpreted as a model of a compressed atom, where the charge is squeezed into a smaller space. In this case the atom ends at the value of  for which
for which  .[4][5]
.[4][5]
Transformations
Introducing the transformation 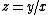 converts the equation to
converts the equation to
This equation is similar to Lane–Emden equation with polytropic index  except the sign difference.
except the sign difference.
The original equation is invariant under the transformation 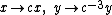 . Hence, the equation can be made equidimensional by introducing
. Hence, the equation can be made equidimensional by introducing  into the equation, leading to
into the equation, leading to
so that the substitution 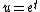 reduces the equation to
reduces the equation to
If 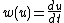 then the above equation becomes
then the above equation becomes
But this first order equation has no known explicit solution, hence, the approach turns to either numerical or approximate methods.
Sommerfeld's approximation
The equation has a particular solution  , which satisfies the boundary condition that
, which satisfies the boundary condition that  as
as 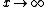 , but not the boundary condition y(0)=1. This particular solution is
, but not the boundary condition y(0)=1. This particular solution is
Arnold Sommerfeld used this particular solution and provided an approximate solution which can satisfy the other boundary condition in 1932.[6] If the transformation 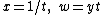 is introduced, the equation becomes
is introduced, the equation becomes
The particular solution in the transformed variable is then 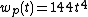 . So one assumes a solution of the form
. So one assumes a solution of the form 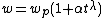 and if this is substituted in the above equation and the coefficients of
and if this is substituted in the above equation and the coefficients of  are equated, one obtains the value for
are equated, one obtains the value for  , which is given by the roots of the equation
, which is given by the roots of the equation 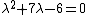 . The two roots are
. The two roots are  . Since this solution already satisfies the second boundary condition, to satisfy the first boundary condition one writes
. Since this solution already satisfies the second boundary condition, to satisfy the first boundary condition one writes
The first boundary condition will be satisfied if  as
as 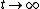 . This condition is satisfied if
. This condition is satisfied if 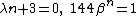 and since
and since  , Sommerfeld found the approximation as
, Sommerfeld found the approximation as  . Therefore, the approximate solution is
. Therefore, the approximate solution is
This solution predicts the correct solution accurately for large  , but still fails near the origin.
, but still fails near the origin.
Solution near origin
Enrico Fermi[7] provided the solution for  and later extended by Baker.[8] Hence for
and later extended by Baker.[8] Hence for  ,
,
where  .[9][10]
.[9][10]
Approach by Majorana
It has been reported by Esposito[11] that the Italian physicist Ettore Majorana found in 1928 a semi-analytical series solution to the Thomas-Fermi equation for the neutral atom, which however remained unpublished until 2001.
Using this approach it is possible to compute the constant B mentioned above to practically arbitrarily high accuracy; for example, its value to 100 digits is  .
.
References
2. ^Bender, Carl M., and Steven A. Orszag. Advanced mathematical methods for scientists and engineers I: Asymptotic methods and perturbation theory. Springer Science & Business Media, 2013.
3. ^pp. 9-12, N. H. March (1983). "1. Origins – The Thomas–Fermi Theory". In S. Lundqvist and N. H. March. Theory of The Inhomogeneous Electron Gas. Plenum Press. {{ISBN|978-0-306-41207-3}}.
4. ^March 1983, p. 10, Figure 1.
5. ^p. 1562, R. P. Feynman, N. Metropolis, and E. Teller. "Equations of State of Elements Based on the Generalized Thomas-Fermi Theory". Physical Review 75, #10 (May 15, 1949), pp. 1561-1573.
6. ^Sommerfeld, A. "Integrazione asintotica dell’equazione differenziale di Thomas–Fermi." Rend. R. Accademia dei Lincei 15 (1932): 293.
7. ^Fermi, Enrico. "Eine statistische Methode zur Bestimmung einiger Eigenschaften des Atoms und ihre Anwendung auf die Theorie des periodischen Systems der Elemente." Zeitschrift für Physik A Hadrons and Nuclei 48.1 (1928): 73–79.
8. ^Baker, Edward B. "The application of the Fermi–Thomas statistical model to the calculation of potential distribution in positive ions." Physical Review 36.4 (1930): 630.
9. ^Comment on: “Series solution to the Thomas–Fermi equation” [Phys. Lett. A 365 (2007) 111], Francisco M.Fernández, Physics Letters A 372, 28 July 2008, 5258-5260, {{doi|10.1016/j.physleta.2008.05.071}}.
10. ^The analytical solution of the Thomas-Fermi equation for a neutral atom, G I Plindov and S K Pogrebnya, Journal of Physics B: Atomic and Molecular Physics 20 (1987), L547, {{doi|10.1088/0022-3700/20/17/001}}.
11. ^{{Cite journal|last=Esposito|first=Salvatore|date=|year=2002|title=Majorana solution of the Thomas-Fermi equation|url=https://arxiv.org/abs/physics/0111167|journal=American Journal of Physics|volume=70|pages=852|via=}}
- Naseer Ahmed Faruqui
- Naseer Malik
- Naseer malli
- Naseeruddin Shah
- Naseerullah Khan Babar
- Naselje Veljko Vlahović
- Nasella laevissima
- Nasella pulchra
- Naselle
- Naser al-Shaer
- Naser-ed-din Shah
- Naser-e Khosraw
- Naserke
- Naser Khader
- Naser Khosro
- Naser Khusraw
- Naser Makarem Shirazi
- Naser Maleknia
- Naser Mohammed ibn Kelaoun
- Naser Nouraei
- Naser Taghvaee
- Naser Taghvai
- Naser Taghvaie
- Naser Taqvaee
- Nas Escobar