- Statement
- The case when
and 
is a metaplectic cusp form - References
In representation theory of mathematics, the Waldspurger formula relates the special values of two L-functions of two related admissible irreducible representations. Let {{Var|k}} be the base field, {{Var|f}} be an automorphic form over {{Var|k}}, π be the representation associated via the Jacquet–Langlands correspondence with {{Var|f}}. Goro Shimura (1976) proved this formula, when {{nowrap|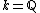
}} and {{Var|f}} is a cusp form; Günter Harder made the same discovery at the same time in an unpublished paper. Marie-France Vignéras (1980) proved this formula, when {{nowrap|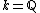}} and {{Var|f}} is a newform. Jean-Loup Waldspurger, for whom the formula is named, reproved and generalized the result of Vignéras in 1985 via a totally different method which was widely used thereafter by mathematicians to prove similar formulas.
Statement
Let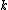 
be a number field, 
be its adele ring, 
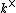be the subgroup of invertible elements of , 
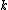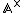be the subgroup of the invertible elements of , 
be three quadratic characters over 
, 
, 
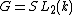be the space of all cusp forms over 
, 
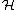be the Hecke algebra of 
. Assume that, 
is an admissible irreducible representation from to , the central character of π is trivial, 
when 
is an archimedean place, 
is a subspace of such that 
. We suppose further that, 
is the Langlands 
-constant [ {{harv | Langlands | 1970 }}; {{harv | Deligne | 1972 }} ] associated to and 
at 
. There is a 
such that 
.
Definition 1. The Legendre symbol 
.
- Comment. Because all the terms in the right either have value +1, or have value −1, the term in the left can only take value in the set {+1, −1}.
Definition 2. Let 
be the discriminant of . 
.
Definition 3. Let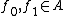 
. 
.
Definition 4. Let 
be a maximal torus of 
, 
be the center of , 
. 
.
- Comment. It's not obvious though, in fact, the function
is a generalization of the Gauss sum.
Let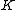 
be a field such that 
. One can choose a K-subspace
of such that (i) 
; (ii) 
. De facto, there is only one such modulo homothety. Let 
be two maximal tori of such that 
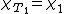and 
. We can choose two elements 
of such that 
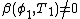and 
.
Definition 5. Let 
be the discriminants of 
. 
.
- Comment. When the two characters{{clarify|date=March 2019|reason=Does this refer to D1,D2 or X1,X2? This should be stated in the text, and be made clear in the later comments following.}} coincide with each other, the right hand side of Definition 5 becomes trivial.
We take 
to be the set {all the finite 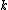-places 
doesn't map non-zero vectors invariant under the action of 
to zero}, 
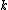to be the set of { all -places 
is real, or finite and special }.
Theorem [ {{harv | Waldspurger | 1985 }}, Thm 4, p. 235 ]. Let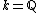 
. We assume that, (i) 
; (ii) for 
, 
. Then, there is a constant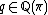 
such that
Comments:
- (i) The formula in the theorem is the well-known Waldspurger formula. It is of global-local nature, in the left with a global part, in the right with a local part. By 2017, mathematicians often call it the classic Waldspurger's formula.
- (ii) It is worthwhile to notice that, when the two characters are equal, the formula can be greatly simplified.
- (iii) [ {{harv | Waldspurger | 1985 }}, Thm 6, p. 241 ] When one of the two characters is
, Waldspurger's formula becomes much more simple. Without loss of generality, we can assume that, 
and 
. Then, there is an element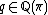 
such that 
.
The case when and is a metaplectic cusp form
Let p be prime number,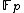 
be the field with p elements, 
be the integer ring of 
. Assume that, 
, D is squarefree of even degree and coprime to N, the prime factorization of 
is 
. We take 
to the set {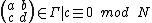 
}, 
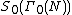to be the set of all cusp forms of level N and depth 0. Suppose that, 
.
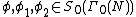Definition 1. Let 
be the Legendre symbol of c modulo d, 
. Metaplectic morphism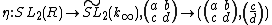 
.
Definition 2. Let 
. Petersson inner product 
.
Definition 3. Let 
. Gauss sum 
.
Let 
be the Laplace eigenvalue of . There is a constant 
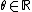such that 
.
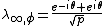Definition 4. Assume that, 
. Whittaker function 
.
Definition 5. Fourier-Whittaker expansion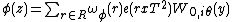 
. One calls 
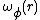the Fourier-Whittaker coefficients of .
Definition 6. Atkin-Lehner operator 
with 
.
Definition 7. Assume that, is a Hecke eigenform. Atkin-Lehner eigenvalue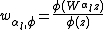 
with 
.
Definition 8. 
.
Let 
be the metaplectic version of , {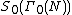 
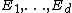} be a nice Hecke eigenbasis for with respect to the Petersson inner product. We note the Shimura correspondence by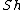 
.
Theorem [ {{harv | Altug | Tsimerman | 2010 }}, Thm 5.1, p. 60 ]. Suppose that, 
, 
is a quadratic character with 
. Then,
.
References
- {{citation | last = Waldspurger | first = Jean-Loup | title = Sur les valeurs de certaines L-fonctions automorphes en leur centre de symétrie | journal = Compositio Mathematica | volume = 54 | issue = 2 | year = 1985 | pages = 173–242 }}
- {{citation | last = Vignéras| first = Marie-France | chapter = Valeur au centre de symétrie des fonctions L associées aux formes modulaire | title = Séminarie de Théorie des Nombres, Paris 1979-1980 | series = Progress in Math.| publisher = Birkhäuser | year = 1981 | pages = 331–356 }}
- {{citation | last = Shimura | first = Gorô | title = On special values of zeta functions associated with cusp forms | journal = Communications on Pure and applied Math. | volume = 29 | year = 1976 | pages = 783–804 }}
- {{cite arxiv |ref= harv |last1= Altug |first1= Salim Ali |last2= Tsimerman |first2= Jacob | title = Metaplectic Ramanujan conjecture over function fields with applications to quadratic forms |eprint= 1008.0430v3 |date= 2010 }}
- {{cite book |ref= harv |last= Langlands |first= Robert |title= On the Functional Equation of the Artin L-Functions |year= 1970 }}
- {{cite conference |ref= harv |last= Deligne |first= Pierre | title = Les constantes des équations fonctionelle des fonctions L |book-title= Modular Functions of One Variable II |pages = 501–597 |conference= International Summer School on Modular functions |location= Antwerp |year= 1972 }}
- Soviet montage
- Soviet montage theory
- Soviet Moon
- Soviet motorway M27
- Soviet movies
- Soviet MVP (ice hockey)
- Soviet national bandy team
- Soviet national football team
- Soviet National League
- Soviet Naval Infantry
- Soviet naval reactors
- Soviet non conformist
- Soviet nuclear forces
- Soviet nuclear research
- Soviet Occupation
- Soviet occupation
- Soviet occupation denial
- Soviet occupation of Baltic states
- Soviet occupation of Bessarabia
- Soviet occupation of Bessarabia and Northern Bukovina
- Soviet occupation of Czechoslovakia
- Soviet occupation of Estonia
- Soviet occupation of Finland
- Soviet occupation of Finnish Karelia
- Soviet occupation of latvia