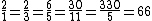- The wild number problem
- History of The Wild Number Problem
- Real wild numbers
- The wild numbers in OEIS
- Sequences of pseudo-wild numbers
- References
The wild number problem
In the novel The Wild Numbers, The Wild Number Problem is formulated as follows:
- Beauregard had defined a number of deceptively simple operations, which, when applied to a whole number, at first resulted in fractions. But if the same steps were repeated often enough, the eventual outcome was once again a whole number. Or, as Beauregard cheerfully observed: “In all numbers lurks a wild number, guaranteed to emerge when you provoke them long enough” . 0 yielded the wild number 11, 1 brought forth 67, 2 itself, 3 suddenly manifested itself as 4769, 4, surprisingly, brought forth 67 again. Beauregard himself had found fifty different wild numbers. The money prize was now awarded to whoever found a new one.[5]
But it has not been specified what those "deceptively simple operations" are. Consequently, there is no way of knowing how those numbers 11, 67, etc. were obtained and no way of finding what the next wild number would be.
History of The Wild Number Problem
The novel The Wild Numbers has constructed a fictitious history for The Wild Number Problem. The important milestones in this history can be summarised as follows.
| Date | Event |
|---|---|
| 1823 | Anatole Millechamps de Beauregard poses the Wild Number Problem in its original form. |
| 1830's | The problem is generalised: How many wild numbers are there? Are there infinitely many wild numbers? It was conjectured that all numbers are wild. |
| 1907 | Heinrich Riedel disproves the conjecture by showing that 3 is not a wild number. Later he also proves that there are infinitely many non-wild numbers. |
| Early 1960s | Dimitri Arkanov sparks renewed interest in the almost forgotten problem by discovering a fundamental relationship between wild numbers and prime numbers. |
| The present | Isaac Swift finds a solution. |
Real wild numbers
In mathematics, the multiplicative semigroup, denoted by W0, generated by the set 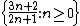 is called the Wooley semigroup in honour of the American mathematician Trevor D. Wooley. The multiplicative semigroup, denoted by W, generated by the set
is called the Wooley semigroup in honour of the American mathematician Trevor D. Wooley. The multiplicative semigroup, denoted by W, generated by the set  is called the wild semigroup. The set of integers in W0 is itself a multiplicative semigroup. It is called the Wooley integer semigroup and members of this semigroup are called Wooley integers. Similarly, the set of integers in W is itself a multiplicative semigroup. It is called the wild integer semigroup and members of this semigroup are called wild numbers.[6]
is called the wild semigroup. The set of integers in W0 is itself a multiplicative semigroup. It is called the Wooley integer semigroup and members of this semigroup are called Wooley integers. Similarly, the set of integers in W is itself a multiplicative semigroup. It is called the wild integer semigroup and members of this semigroup are called wild numbers.[6]
The wild numbers in OEIS
The On-Line Encyclopedia of Integer Sequences (OEIS) has an entry with the identifying number {{OEIS link|A058883}} relating to the wild numbers. According to OEIS, "apparently these are completely fictional and there is no mathematical explanation". However, the OEIS has some entries relating to pseudo-wild numbers carrying well-defined mathematical explanations.[4]
Sequences of pseudo-wild numbers
Even though the sequence of wild numbers is entirely fictional, several mathematicians have tried to find rules that would generate the sequence of the fictional wild numbers. All these attempts have resulted in failures. However, in the process, certain new sequences of integers were created having similar wild and erratic behavior. These well-defined sequences are referred to as sequences of pseudo-wild numbers. A good example of this is the one discovered by the Dutch mathematician Floor van Lamoen. This sequence is defined as follows:[7][8]
For a rational number p/q let
.
For a positive integer n, the n-th pseudo-wild number is the number obtained by iterating f, starting at n/1, until an integer is reached, and if no integer is reached the pseudo-wild number is 0.
For example, taking n=2, we have
and so the 2-nd pseudo-wild number is 66. The first few pseudo-wild numbers are
66, 66, 462, 180, 66, 31395, 714, 72, 9, 5.
References
2. ^{{cite book|last1=Michele Emmer (Editor)|title=Imagine Math 2: Between Culture and Mathematics|date=2013|publisher=Springer Science & Business Media|isbn=9788847028890|pages=37–38}}
3. ^{{cite journal | last1 = Applegate | first1 = David | author1-link = David Applegate | last2 = Lagarias | first2 = Jeffrey C. | author2-link = Jeffrey Lagarias | doi = 10.1016/j.jnt.2005.06.010 | issue = 1 | journal = Journal of Number Theory | mr = 2204740 | pages = 146–159 | title = The {{math|3x + 1}} semigroup | volume = 117 | year = 2006}}
4. ^1 {{cite web|title=A058883 : The "Wild Numbers", from the novel of the same title (Version 1).|url=https://oeis.org/A058883|website=OEIS|publisher=The OEIS Foundation.|accessdate=19 March 2016}}
5. ^{{cite book|last1=Philibert Schogt|title=The Wild Numbers: A Novel|date=March 23, 2000|publisher=Four Walls Eight Windows|isbn=978-1568581668|edition=First|page=34}}
6. ^{{cite journal|last1=Jeffrey C. Lagarias|title=Wild and Wooley Numbers|journal=American Mathematical Monthly|date=February 2006|volume=113|issue=2|pages=98–108|doi=10.2307/27641862|url=https://www.maa.org/sites/default/files/pdf/upload_library/22/Ford/lagarias97.pdf|accessdate=28 March 2016|jstor=27641862}}
7. ^{{cite arxiv |eprint=1211.6583|last1=Schogt|first1=Philibert|title=The Wild Number Problem: Math or fiction?|class=math.HO|year=2012}}
8. ^{{cite web|title=A059175 |url=https://oeis.org/A059175|website=OEIS|publisher=The OEIS Foundation.|accessdate=30 March 2016}}
- London Pride Sightseeing
- London Prize Ring rules
- London Probation
- London Probation Area
- London Program of Hofstra
- London Progressive Journal
- London Proms
- London proper
- London Property Letter
- London Pubs
- London quarter
- London Quarterly
- London Quarterly and Holborn Review
- London / Queen's Club
- London Rail Authority
- London Rail Concession
- London Reception Centre
- London Regatta Centre
- London Regional Children’s Museum
- London Regional Select Committee
- London R. Ferebee
- London Ribfest
- London River
- London Riverside Parklands
- London Road (Brighton) station