1. Definition

2. Example

In mathematics, the base flow of a random dynamical system is the dynamical system defined on the "noise" probability space that describes how to "fast forward" or "rewind" the noise when one wishes to change the time at which one "starts" the random dynamical system.

Definition

In the definition of a random dynamical system, one is given a family of maps 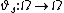on a probability space . The measure-preserving dynamical system 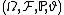is known as the base flow of the random dynamical system. The maps 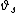are often known as shift maps since they "shift" time. The base flow is often ergodic.

The parameter may be chosen to run over

• (a two-sided continuous-time dynamical system);
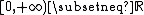• (a one-sided continuous-time dynamical system);
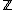• (a two-sided discrete-time dynamical system);
• (a one-sided discrete-time dynamical system).

Each map 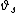is required

• to be a 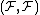-measurable function: for all ,
• to preserve the measure : for all , .

Furthermore, as a family, the maps 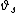satisfy the relations

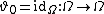• , the identity function on ;
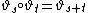• for all and for which the three maps in this expression are defined. In particular, if 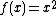exists.

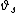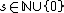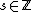In other words, the maps form a commutative monoid (in the cases and ) or a commutative group (in the cases and ).

Example

In the case of random dynamical system driven by a Wiener process 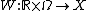, where is the two-sided classical Wiener space, the base flow 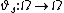would be given by

.

This can be read as saying that 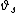"starts the noise at time instead of time 0".

• ECBEA
• E. C. Benedict
• EC Bentley
• E. C. Bingham
• EC Biotechnology Directive
• ECB key
• ECB mode
• ECB National Academy
• Ecbolemia
• Ecbolium linneanum
• ECB Premier League
• ECB Premier Leagues
• ECB Recreation XI
• E. C. Bridgman
• ECBT
• E C Buley
• EC Buley
• Ecca Pass
• EC-card
• Eccard Freiherr von Gablenz
• E. C. Case
• ECCC
• ECC Chipkill
• ECC (disambiguation)
• ECCE