“Carathéodory's extension theorem”的意思、由来-开放百科全书

词条

Carathéodory's extension theorem

释义

Semi-ring and ring
Definitions Properties Motivation Example
Statement of the theorem
Examples
Non-uniqueness of extension
See also
References

In measure theory, Carathéodory's extension theorem (named after the mathematician Constantin Carathéodory) states that any pre-measure defined on a given ring R of subsets of a given set Ω can be extended to a measure on the σ-algebra generated by R, and this extension is unique if the pre-measure is σ-finite. Consequently, any pre-measure on a ring containing all intervals of real numbers can be extended to the Borel algebra of the set of real numbers. This is an extremely powerful result of measure theory, and leads, for example, to the Lebesgue measure.

Semi-ring and ring

Definitions

For a given set , we may define a semi-ring as a subset of 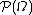, the power set of , which has the following properties:

For all 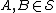, we have 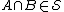(closed under pairwise intersections)
For all 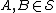, there exist disjoint sets 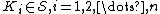, such that 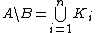(relative complements can be written as finite disjoint unions).

The first property can be replaced with since .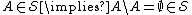

With the same notation, we define a ring as a subset of the power set of which has the following properties:

For all 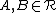, we have 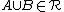(closed under pairwise unions)
For all 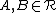, we have (closed under relative complements).

Thus, any ring on is also a semi-ring.

Sometimes, the following constraint is added in the measure theory context:

is the disjoint union of a countable family of sets in .

A field of sets (respectively, a semi-field) is a ring (respectively, a semi-ring) that also contains as one of its elements.

Properties

Arbitrary (possibly uncountable) intersections of rings on Ω are still rings on Ω.
If A is a non-empty subset of 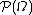, then we define the ring generated by A (noted R(A)) as the smallest ring containing A. It is straightforward to see that the ring generated by A is equivalent to the intersection of all rings containing A.
For a semi-ring S, the set containing all finite disjoint unions of sets of S is the ring generated by S:

(One can show that R(S) is simply the set containing all finite unions of sets in S).

A content μ defined on a semi-ring S can be extended on the ring generated by S. Such an extension is unique. The extended content can be written:

for , with the A_i in S.

In addition, it can be proved that μ is a pre-measure if and only if the extended content is also a pre-measure, and that any pre-measure on R(S) that extends the pre-measure on S is necessarily of this form.

Motivation

In measure theory, we are not interested in semi-rings and rings themselves, but rather in σ-algebras generated by them. The idea is that it is possible to build a pre-measure on a semi-ring S (for example Stieltjes measures), which can then be extended to a pre-measure on R(S), which can finally be extended to a measure on a σ-algebra through Caratheodory's extension theorem. As σ-algebras generated by semi-rings and rings are the same, the difference does not really matter (in the measure theory context at least). Actually, Carathéodory's extension theorem can be slightly generalized by replacing ring by semi-field.^[1]

The definition of semi-ring may seem a bit convoluted, but the following example shows why it is useful (moreover it allows us to give an explicit representation of the smallest ring containing some semi-ring).

Example

Think about the subset of 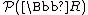defined by the set of all half-open intervals [a, b) for a and b reals. This is a semi-ring, but not a ring. Stieltjes measures are defined on intervals; the countable additivity on the semi-ring is not too difficult to prove because we only consider countable unions of intervals which are intervals themselves. Proving it for arbitrary countable unions of intervals is accomplished using Caratheodory's theorem.

Statement of the theorem

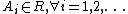Let be a ring on and let {{nowrap|μ: R → [0, + ∞]}} be a pre-measure on R, i.e. for all sets for which there exists a countable decomposition in disjoint sets , we have .

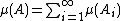Let σ(R) be the σ-algebra generated by R. The pre-measure condition is a necessary condition for to be the restriction to R of a measure on .

The Carathéodory's extension theorem states that it is also sufficient^[2], i.e. there exists a measure {{nowrap|μ′: σ(R) → [0, + ∞]}} such that {{nowrap|μ′}} is an extension of μ. (That is, {{nowrap|μ′ {{!}}_R {{=}} μ}}). Moreover, if μ is σ-finite then the extension {{nowrap|μ′}} is unique (and also σ-finite).^[3]

Examples

Non-uniqueness of extension

Here are some examples where there is more than one extension of a pre-measure to the generated σ-algebra.

For the first example, take the algebra generated by all half-open intervals [a,b) on the real, and give such intervals measure infinity if they are non-empty. The Caratheodory extension gives all non-empty sets measure infinity. Another extension is given by counting measure.

Here is a second example, closely related to the failure of some forms of Fubini's theorem for spaces that are not σ-finite.

Suppose that X is the unit interval with Lebesgue measure and Y is the unit interval with the discrete counting measure. Let the ring R be generated by products A×B where A is Lebesgue measurable and B is any subset, and give this set the measure μ(A)card(B). This has a very large number of different extensions to a measure; for example:

The measure of a subset is the sum of the measures of its horizontal sections. This is the smallest possible extension. Here the diagonal has measure 0.
The measure of a subset is where n(x) is the number of points of the subset with given x-coordinate. The diagonal has measure 1.
The Caratheodory extension, which is the largest possible extension. Any subset of finite measure is contained in some union of a countable number of horizontal lines and in some set whose projection to the x-axis has measure 0. In particular the diagonal has measure infinity.

References

1. ^{{cite book|last1=Klenke|first1=Achim|title=Probability Theory|date=2014|isbn=978-1-4471-5360-3|page=Theorem 1.53|doi=10.1007/978-1-4471-5361-0|series=Universitext}}
2. ^Vaillant, Theorem 4
3. ^Ash, p19

* Noel Vaillant, Caratheodory's Extension, on probability.net. A clear demonstration of the theorem through exercises.

{{cite book | author=Robert B. Ash | title=Probability and Measure theory | publisher=Academic Press; 2 edition | year=1999 | isbn=978-0-12-065202-0}}

1 : Theorems in measure theory

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。