1. Definition

2. Intuition

3. Coarse Maps

4. Examples

5. See also

6. References

"Coarse space" redirects here. For the use of "coarse space" in numerical analysis, see coarse problem.

In the mathematical fields of geometry and topology, a coarse structure on a set X is a collection of subsets of the cartesian product X × X with certain properties which allow the large-scale structure of metric spaces and topological spaces to be defined.

The concern of traditional geometry and topology is with the small-scale structure of the space: properties such as the continuity of a function depend on whether the inverse images of small open sets, or neighborhoods, are themselves open. Large-scale properties of a space—such as boundedness, or the degrees of freedom of the space—do not depend on such features. Coarse geometry and coarse topology provide tools for measuring the large-scale properties of a space, and just as a metric or a topology contains information on the small-scale structure of a space, a coarse structure contains information on its large-scale properties.

Properly, a coarse structure is not the large-scale analog of a topological structure, but of a uniform structure.

Definition

A coarse structure on a set X is a collection E of subsets of X × X (therefore falling under the more general categorization of binary relations on X) called controlled sets, and so that E possesses the identity relation, is closed under taking subsets, inverses, and finite unions, and is closed under composition of relations. Explicitly:

1. Identity/diagonal

The diagonal Δ = {(x, x) : x in X} is a member of E—the identity relation.

2. Closed under taking subsets

If E is a member of E and F is a subset of E, then F is a member of E.

3. Closed under taking inverses

If E is a member of E then the inverse (or transpose) E ⁻¹ = {(y, x) : (x, y) in E} is a member of E—the inverse relation.

4. Closed under taking unions

If E and F are members of E then the union of E and F is a member of E.

5. Closed under composition

If E and F are members of E then the product E o F = {(x, y) : there is a z in X such that (x, z) is in E, (z, y) is in F} is a member of E—the composition of relations.

A set X endowed with a coarse structure E is a coarse space.

The set E[K] is defined as {x in X : there is a y in K such that (x, y) is in E}. We define the section of E by x to be the set E[{x}], also denoted E _x. The symbol E^y denotes the set E ⁻¹[{y}]. These are forms of projections.

Intuition

The controlled sets are "small" sets, or "negligible sets": a set A such that A × A is controlled is negligible, while a function f : X → X such that its graph is controlled is "close" to the identity. In the bounded coarse structure, these sets are the bounded sets, and the functions are the ones that are a finite distance from the identity in the uniform metric.

Coarse Maps

Given a set S and a coarse structure X, we say that the maps and are close if is a controlled set. A subset B of X is said to be bounded if is a controlled set.

For coarse structures X and Y, we say that is coarse if for each bounded set B of Y the set 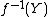is bounded in X and for each controlled set E of X the set is controlled in Y.^[1] X and Y are said to be coarsely equivalent if there exists coarse maps and 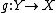such that is close to and is close to .

Examples

• The bounded coarse structure on a metric space (X, d) is the collection E of all subsets E of X × X such that sup{d(x, y) : (x, y) is in E} is finite.

With this structure, the integer lattice Zⁿ is coarsely equivalent to n-dimensional Euclidean space.

• A space X where X × X is controlled is called a bounded space. Such a space is coarsely equivalent to a point. A metric space with the bounded coarse structure is bounded (as a coarse space) if and only if it is bounded (as a metric space).
• The trivial coarse structure only consists of the diagonal and its subsets.

In this structure, a map is a coarse equivalence if and only if it is a bijection (of sets).

• The C₀ coarse structure on a metric space X is the collection of all subsets E of X × X such that for all ε > 0 there is a compact set K of X such that d(x, y) < ε for all (x, y) in E − K × K. Alternatively, the collection of all subsets E of X × X such that {(x, y) in E : d(x, y) ≥ ε} is compact.
• The discrete coarse structure on a set X consists of the diagonal together with subsets E of X × X which contain only a finite number of points (x, y) off the diagonal.
• If X is a topological space then the indiscrete coarse structure on X consists of all proper subsets of X × X, meaning all subsets E such that E [K] and E ⁻¹[K] are relatively compact whenever K is relatively compact.

See also

• uniform space
• quasi-isometry

References

• John Roe, Lectures in Coarse Geometry, University Lecture Series Vol. 31, American Mathematical Society: Providence, Rhode Island, 2003. Corrections to Lectures in Coarse Geometry
• {{ cite journal

• Caffè americano
• Caffè espresso
• Caffè Gambrinus
• Caffè Michelangiolo
• Caffè pagato
• Caffè Pascucci
• Caffè Pedrocchi
• Caffè Quadri
• Caffé d'orzo
• Caffé Vittoria
• Cafiero
• Cafilon
• Cafinitrina
• Cafipel
• CA flag
• Caflisch
• CAF Marathon
• CAF Maroc Telecom African Youth Championship U-17 Morocco 2013
• Cafnan, Mechell
• CAF Nations Cup
• CAFOs
• Ca 'Foscari
• CAFR
• Cafre
• CAFRE