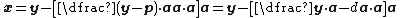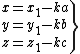1. Converting general problem to distance-from-origin problem

2. Restatement using linear algebra

3. Why this is the closest point

4. Closest point and distance for a hyperplane and arbitrary point

5. See also

6. References

In Euclidean space, the point on a plane 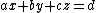that is closest to the origin has the Cartesian coordinates , where

.{{Citation needed|date=January 2016}}

The distance between the origin and point is .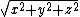

If what is desired is the distance from a point not at the origin to the nearest point on a plane, this can be found by a change of variables that moves the origin to coincide with the given point.

Converting general problem to distance-from-origin problem

Suppose we wish to find the nearest point on a plane to the point (), where the plane is given by 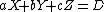. We define 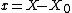, , 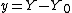, 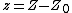and 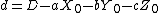, to obtain 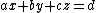as the plane expressed in terms of the transformed variables. Now the problem has become one of finding the nearest point on this plane to the origin, and its distance from the origin. The point on the plane in terms of the original coordinates can be found from this point using the above relationships between and , between and , and between and ; the distance in terms of the original coordinates is the same as the distance in terms of the revised coordinates.

Restatement using linear algebra

The formula for the closest point to the origin may be expressed more succinctly using notation from linear algebra. The expression 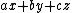in the definition of a plane is a dot product 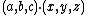, and the expression 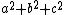appearing in the solution is the squared norm 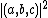. Thus, if 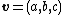is a given vector, the plane may be described as the set of vectors for which 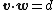and the closest point on this plane is the vector

.^[1][2]

The Euclidean distance from the origin to the plane is the norm of this point,

.

Why this is the closest point

In either the coordinate or vector formulations, one may verify that the given point lies on the given plane by plugging the point into the equation of the plane.

To see that it is the closest point to the origin on the plane, observe that is a scalar multiple of the vector defining the plane, and is therefore orthogonal to the plane.

Thus, if is any point on the plane other than itself, then the line segments from the origin to and from to form a right triangle, and by the Pythagorean theorem the distance from the origin to is

.

Since must be a positive number, this distance is greater than 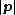, the distance from the origin to .^[2]

Alternatively, it is possible to rewrite the equation of the plane using dot products with in place of the original dot product with (because these two vectors are scalar multiples of each other) after which the fact that is the closest point becomes an immediate consequence of the Cauchy–Schwarz inequality.^[1]

Closest point and distance for a hyperplane and arbitrary point

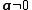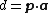The vector equation for a hyperplane in -dimensional Euclidean space through a point with normal vector is or where .^[3]

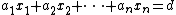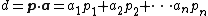The corresponding Cartesian form is where .^[3]

The closest point on this hyperplane to an arbitrary point is

and the distance from to the hyperplane is

.^[3]

Written in Cartesian form, the closest point is given by for where

,

and the distance from to the hyperplane is

.

Thus in the point on a plane 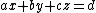closest to an arbitrary point 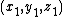is given by

where

,

and the distance from the point to the plane is

.

See also

• Distance from a point to a line
• Hesse normal form
• Skew lines#Distance

References

• 986 CE
• 98.6 (disambiguation)
• 986 (year)
• (98713) 2000 XH43
• 987 AD
• 987 CE
• 98.7 Sea FM
• 987 (year)
• 98866 Giannabussolari
• 9887 Ashikaga
• 988 AD
• 988 Appella
• 988 CE
• 988 (year)
• 9898 Yoshiro
• 989 AD
• 989 CE
• 989 (year)
• 98 CE
• 98 (disambiguation)
• 98five Sonshine FM
• 98 KUPD
• 98 Piscium
• 98th (Argyllshire Highlanders) Regiment of Foot
• 98th Aviation Troop Command