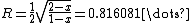- Cartesian coordinates
- Related polyhedra Great inverted pentagonal hexecontahedron
- See also
- References
- External links
In geometry, the great inverted snub icosidodecahedron is a uniform star polyhedron, indexed as U69. It is given a Schläfli symbol sr{5/3,3}.
Cartesian coordinates
Cartesian coordinates for the vertices of a great inverted snub icosidodecahedron are all the even permutations of
(±2α, ±2, ±2β),
(±(α−βτ−1/τ), ±(α/τ+β−τ), ±(−ατ−β/τ−1)),
(±(ατ−β/τ+1), ±(−α−βτ+1/τ), ±(−α/τ+β+τ)),
(±(ατ−β/τ−1), ±(α+βτ+1/τ), ±(−α/τ+β−τ)) and
(±(α−βτ+1/τ), ±(−α/τ−β−τ), ±(−ατ−β/τ+1)),
with an even number of plus signs, where
α = ξ−1/ξ
and
β = −ξ/τ+1/τ2−1/(ξτ),
where τ = (1+{{radic|5}})/2 is the golden mean and
ξ is the greater positive real solution to ξ3−2ξ=−1/τ, or approximately 1.2224727.
Taking the odd permutations of the above coordinates with an odd number of plus signs gives another form, the enantiomorph of the other one.
The circumradius for unit edge length is
where 
is the appropriate root of 
. The four positive real roots of the sextic in 
are the circumradii of the snub dodecahedron (U29), great snub icosidodecahedron (U57), great inverted snub icosidodecahedron (U69), and great retrosnub icosidodecahedron (U74).
Related polyhedra
Great inverted pentagonal hexecontahedron
{{Uniform polyhedra db|Uniform dual polyhedron stat table|Gisid}}The great inverted pentagonal hexecontahedron is a nonconvex isohedral polyhedron. It is composed of 60 self-intersecting pentagonal faces, 150 edges and 92 vertices.
It is the dual of the uniform great inverted snub icosidodecahedron.
{{-}}See also
- List of uniform polyhedra
- Great snub icosidodecahedron
- Great retrosnub icosidodecahedron
References
- {{Citation | last1=Wenninger | first1=Magnus | author1-link=Magnus Wenninger | title=Dual Models | publisher=Cambridge University Press | isbn=978-0-521-54325-5 |mr=730208 | year=1983}} p. 126
External links
- {{mathworld | urlname = GreatInvertedPentagonalHexecontahedron| title =Great inverted pentagonal hexecontahedron}}
- {{mathworld | urlname = GreatInvertedSnubIcosidodecahedron | title = Great inverted snub icosidodecahedron}}
- Patriarch Photius I of Constantinople
- Patriarch Photius of Alexandria
- Patriarch Politianus of Alexandria
- Patriarch Polyeuctus
- Patriarch Proclus of Constantinople
- Patriarch Raphael I Bidawid
- Patriarch Sabbas of Alexandria
- Patriarch Sarkis of Jerusalem
- Patriarchs (bible)
- Patriarch Sergius
- Patriarch Sergius I
- Patriarch Sergius XVII
- Patriarch's Hope
- Patriarch Silvester of Alexandria
- Patriarch Sisinnius I of Constantinople
- Patriarchs (Judaism)
- Patriarchs of Aquileia
- Patriarchs of Eritrea
- Patriarchs of the east
- Patriarch Sophronius III of Alexandria
- Patriarch Sophronius II of Alexandria
- Patriarch Sophronius I of Alexandria
- Patriarch Sophronius IV of Alexandria
- Patriarchs (solitaire)
- Patriarch State of Friuli