“Handle decompositions of 3-manifolds”的意思、由来-开放百科全书

词条

Handle decompositions of 3-manifolds

释义

Heegaard splittings
Examples
Orientability
Heegaard genus
References

In mathematics, a handle decomposition of a 3-manifold allows simplification of the original 3-manifold into pieces which are easier to study.

Heegaard splittings

An important method used to decompose into handlebodies is the Heegaard splitting, which gives us a decomposition in two handlebodies of equal genus.^[1]

Examples

As an example: lens spaces are orientable 3-spaces, and allow decomposition into two solid-tori which are genus-one-handlebodies. The genus one non-orientable space is a space which is the union of two solid Klein bottles and corresponds to the twisted product of the 2-sphere and the 1-sphere: .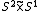

Orientability

Each orientable 3-manifold is the union of exactly two orientable handlebodies; meanwhile, each non-orientable one needs three orientable handlebodies.

Heegaard genus

The minimal genus of the glueing boundary determines what is known as the Heegaard genus. For non-orientable spaces an interesting invariant is the tri-genus.

References

1. ^{{cite book|title=Quantum Invariants of Knots and 3-manifolds|first=Vladimir G. |last=Turaev|publisher=Walter de Gruyter|year= 1994|isbn=3-11-013704-6}}

J.C. Gómez Larrañaga, W. Heil, V.M. Núñez. Stiefel-Whitney surfaces and decompositions of 3-manifolds into handlebodies, Topology Appl. 60 (1994), 267-280.
J.C. Gómez Larrañaga, W. Heil, V.M. Núñez. Stiefel-Whitney surfaces and the trigenus of non-orientable 3-manifolds, Manuscripta Math. 100 (1999), 405-422.

2 : 3-manifolds|Topology

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。