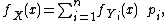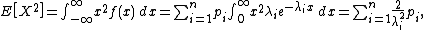“Hyperexponential distribution”的意思、由来-开放百科全书

In probability theory, a hyperexponential distribution is a continuous probability distribution whose probability density function of the random variable X is given by

where each Y_i is an exponentially distributed random variable with rate parameter λ_i, and p_i is the probability that X will take on the form of the exponential distribution with rate λ_i.^[1] It is named the hyperexponential distribution since its coefficient of variation is greater than that of the exponential distribution, whose coefficient of variation is 1, and the hypoexponential distribution, which has a coefficient of variation smaller than one. While the exponential distribution is the continuous analogue of the geometric distribution, the hyperexponential distribution is not analogous to the hypergeometric distribution. The hyperexponential distribution is an example of a mixture density.

An example of a hyperexponential random variable can be seen in the context of telephony, where, if someone has a modem and a phone, their phone line usage could be modeled as a hyperexponential distribution where there is probability p of them talking on the phone with rate λ₁ and probability q of them using their internet connection with rate λ₂.

Properties

Since the expected value of a sum is the sum of the expected values, the expected value of a hyperexponential random variable can be shown as

The standard deviation exceeds the mean in general (except for the degenerate case of all the λs being equal), so the coefficient of variation is greater than 1.

Fitting

A given probability distribution, including a heavy-tailed distribution, can be approximated by a hyperexponential distribution by fitting recursively to different time scales using Prony's method.^[3]

See also

References

1. ^{{Cite journal | last1 = Singh | first1 = L. N. | last2 = Dattatreya | first2 = G. R. | doi = 10.1080/15501320701259925 | title = Estimation of the Hyperexponential Density with Applications in Sensor Networks | journal = International Journal of Distributed Sensor Networks | volume = 3 | issue = 3 | pages = 311 | year = 2007 | pmid = | pmc = }}
2. ^{{cite book|author1=H.T. Papadopolous |author2=C. Heavey |author3=J. Browne |title=Queueing Theory in Manufacturing Systems Analysis and Design|year=1993|publisher=Springer|isbn=9780412387203|page=35|url=https://books.google.com/books?id=9pf5MCf9VDYC&pg=PA35}}
3. ^{{Cite journal | last1 = Feldmann | first1 = A. | authorlink1 = Anja Feldmann| last2 = Whitt | first2 = W. | authorlink2 = Ward Whitt| doi = 10.1016/S0166-5316(97)00003-5 | title = Fitting mixtures of exponentials to long-tail distributions to analyze network performance models | journal = Performance Evaluation | volume = 31 | issue = 3–4 | pages = 245 | year = 1998 | pmid = | pmc = | url = http://www.columbia.edu/~ww2040/FittingMixturesPerfEval98.pdf}}