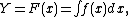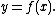请输入您要查询的百科知识：

　

词条 Integraph
释义

  1. History

  2. Description

  3. Mechanism

  4. See also

  5. References

{{refimprove|date=May 2015}}

An Integraph is a mechanical analog computing device for plotting the integral of a graphically defined function.

History

It was invented independently about 1880 by the British physicist Sir Charles Vernon Boys and by Bruno Abdank-Abakanowicz, a Polish-Lithuanian mathematician/electrical engineer from the Russian Empire. Abakanowicz's design was constructed by Coradi of Zurich.

Description

The input to the integraph is a tracing point that is moved to trace the input curve. The output is defined by the path a disk that rolls along the paper without slipping. The mechanism sets the angle of the output disk based on the position of the input curve: if the input is zero, the disk is angled to roll straight, parallel to the x axis on the Cartesian plane. If the input is above zero the disk is angled slightly toward the positive y direction, such that the y value of its position increases as it rolls in that direction. If the input is below zero, the disk is angled the other way such that its y position decreases as it rolls.

The hardware consists of a rectangular carriage which moves left to right on rollers. Two sides of the carriage run parallel to the x axis. The other two sides are parallel to the y axis. Along the trailing vertical (y axis) rail slides a smaller carriage holding a tracing point. Along the leading vertical rail slides a second smaller carriage to which is affixed a small, sharp disc, which rests and rolls (but does not slide) on the graphing paper. The trailing carriage is connected both with a point in the center of the carriage and the disc on the leading rail by a system of sliding crossheads and wires, such that the tracing point must follow the disc's tangential path.

Mechanism

The integraph plots (traces) the integral curve

when we are given the differential curve,

The mathematical basis of the mechanism depends on the following considerations:[1] For any point {{math|(x, y)}} of the differential curve, construct the auxiliary triangle with vertices {{math|(x, y), (x, 0)}} and {{math|(x − 1, 0)}}. The hypotenuse of this right triangle intersects the {{math|X}}-axis making an angle the value of whose tangent is {{math|y}}. This hypotenuse is parallel to the tangent line of the integral curve at {{math|(X, Y)}} that corresponds to {{math|(x, y)}}.

The integraph may be used to obtain a quadrature of the circle. If the differential curve is the unit circle, the integral curve intersects the lines {{math|1=X = ± 1}} at points that are equally spaced at a distance of {{pi}}/2.[1]

See also

  • Mathematical instrument
  • Planimeter

References

1. ^1 {{citation|first=Felix|last=Klein|title=Famous Problems of Elementary Geometry|year=1956|origyear=1930|publisher=Dover|pages=78–80}}
  • Granville, William. Elements of Differential and Integral Calculus, New Edition. Ginn and Company. NY, NY, 1934
  • Bruno Abdank-Abakanowicz, Les intégraphes, la courbe intégrale et ses applications: étude sur un nouveau système d'intégrateurs mécaniques.

Gauthier-Villars, 1886 [https://books.google.com/books?id=j489AAAAYAAJ&dq=Les%20Int%C3%A9graphes%201886&pg=PR3#v=onepage&q=Les%20Int%C3%A9graphes%201886&f=false available at Google Books]

{{Authority control}}

3 : 1880 introductions|Mathematical tools|Computer-related introductions in the 1880s
随便看

　

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。

　

Copyright © 2023 OENC.NET All Rights Reserved
京ICP备2021023879号 更新时间：2024/11/13 12:33:21