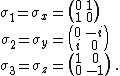- Examples
- Symmetry
- Properties
- See also
- References
In mathematics, an involutory matrix is a matrix that is its own inverse. That is, multiplication by matrix A is an involution if and only if A2 = I. Involutory matrices are all square roots of the identity matrix. This is simply a consequence of the fact that any nonsingular matrix multiplied by its inverse is the identity.[1]
Examples
The 2 × 2 real matrix 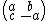 is involutory provided that
is involutory provided that  [2]
[2]
The Pauli matrices in M(2,C) are involutory:
One of the three classes of elementary matrix is involutory, namely the row-interchange elementary matrix. A special case of another class of elementary matrix, that which represents multiplication of a row or column by −1, is also involutory; it is in fact a trivial example of a signature matrix, all of which are involutory.
Some simple examples of involutory matrices are shown below.
where
I is the identity matrix (which is trivially involutory);
R is an identity matrix with a pair of interchanged rows;
S is a signature matrix.
Clearly, any block-diagonal matrices constructed from involutory matrices will also be involutory, as a consequence of the linear independence of the blocks.
Symmetry
An involutory matrix which is also symmetric is an orthogonal matrix, and thus represents an isometry (a linear transformation which preserves Euclidean distance). Conversely every orthogonal involutory matrix is symmetric.[3]
As a special case of this, every reflection matrix is an involutory.
Properties
The determinant of an involutory matrix over any field is ±1.[4]
If A is an n × n matrix, then A is involutory if and only if ½(A + I) is idempotent. This relation gives a bijection between involutory matrices and idempotent matrices.[4]
If A is an involutory matrix in M(n ,ℝ), a matrix algebra over the real numbers, then the subalgebra {x I + y A: x,y ∈ ℝ} generated by A is isomorphic to the split-complex numbers.
if A and B are two involutory matrices which commute with each other then AB is also involutory.
if A is involutory matrix then every natural power of A is involutory. In fact, An will be equal to A if n is odd and I if n is even.
See also
- Affine involution
References
2. ^Peter Lancaster & Miron Tismenetsky (1985) The Theory of Matrices, 2nd edition, pp 12,13 Academic Press {{ISBN|0-12-435560-9}}
3. ^{{citation | last = Govaerts | first = Willy J. F. | doi = 10.1137/1.9780898719543 | isbn = 0-89871-442-7 | location = Philadelphia, PA | mr = 1736704 | page = 292 | publisher = Society for Industrial and Applied Mathematics (SIAM) | title = Numerical methods for bifurcations of dynamical equilibria | url = https://books.google.com/books?id=rqvYq19qwiwC&pg=PA292 | year = 2000}}.
4. ^1 {{citation | last = Bernstein | first = Dennis S. | contribution = 3.15 Facts on Involutory Matrices | edition = 2nd | isbn = 978-0-691-14039-1 | location = Princeton, NJ | mr = 2513751 | pages = 230–231 | publisher = Princeton University Press | title = Matrix Mathematics | url = https://books.google.com/books?id=-c0NxJg4vHMC&pg=PA230 | year = 2009}}.
- Wendo kolosoy
- Wendol
- Wendonai
- Wendoree Park
- Wendoree Park, New South Wales
- Wendorf, Mecklenburg-Vorpommern
- Wendouree center for the perfoming arts
- Wendouree Centre for the Performing Arts
- Wendouree, Victoria
- Wendouree Village, Victoria
- Wendover AFB
- Wendover Air Force Base
- Wendover Air Force Range
- Wendover Airport
- Wendover Arm Canal
- Wendover Army Air Field
- Wendover Ave
- Wendover Avenue
- Wendover Avenue (Greensboro)
- Wendover Church of England Junior School
- Wendover Cutoff
- Wendover Cut-off
- Wendover (disambiguation)
- Wendover Field
- Wendover Middle School