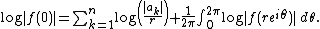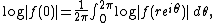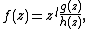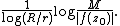“Jensen's formula”的意思、由来-开放百科全书

词条

Jensen's formula

释义

The statement
Poisson–Jensen formula
References

In the mathematics, and specifically complex analysis, Jensen's formula, introduced by {{harvs|txt|authorlink=Johan Jensen (mathematician)|first=Johan|last= Jensen|year=1899}}, relates the average magnitude of an analytic function on a circle with the number of its zeros inside the circle. It forms an important statement in the study of entire functions.

The statement

Suppose that ƒ is an analytic function in a region in the complex plane which contains the closed disk D of radius r about the origin, a₁, a₂, ..., a_n are the zeros of ƒ in the interior of D repeated according to multiplicity, and ƒ(0) ≠ 0. Jensen's formula states that

This formula establishes a connection between the moduli of the zeros of the function ƒ inside the disk D and the average of log |f(z)| on the boundary circle |z| = r, and can be seen as a generalisation of the mean value property of harmonic functions. Namely, if f has no zeros in D, then Jensen's formula reduces to

which is the mean-value property of the harmonic function .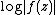

An equivalent statement of Jensen's formula that is frequently used is

where denotes the number of zeros of in the disc of radius centered at the origin.

Jensen's formula may be generalized for functions which are merely meromorphic on D. Namely, assume that

where g and h are analytic functions in D having zeros at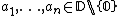

and

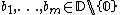
respectively, then Jensen's formula for meromorphic functions states that

Jensen's formula can be used to estimate the number of zeros of analytic function in a circle. Namely, if f is a function analytic in a disk of radius R centered at z₀ and if |f| is bounded by M on the boundary of that disk, then the number of zeros of f in a circle of radius r<R centered at the same point z₀ does not exceed

Jensen's formula is an important statement in the study of value distribution of entire and meromorphic functions. In particular, it is the starting point of Nevanlinna theory.

Poisson–Jensen formula

Jensen's formula is a consequence of the more general Poisson–Jensen formula, which in turn follows from Jensen's formula by applying a Möbius transformation to z. It was introduced and named by Rolf Nevanlinna. If f is a function which is analytic in the unit disk, with zeros a₁, a₂, ..., a_n located in the interior of the unit disk, then for every 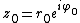in the unit disk the Poisson–Jensen formula states that

Here,

is the Poisson kernel on the unit disk.

If the function f has no zeros in the unit disk, the Poisson-Jensen formula reduces to

which is the Poisson formula for the harmonic function .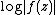

References

{{citation | authorlink=Lars Ahlfors | first = Lars V. | last=Ahlfors | title = Complex analysis. An introduction to the theory of analytic functions of one complex variable | edition=3rd | publisher = McGraw–Hill | year = 1979 | isbn=0-07-000657-1 | series=International Series in pure and applied Mathematics | location=Düsseldorf | zbl=0395.30001 }}
{{citation | last1=Jensen | first1=J. | title=Sur un nouvel et important théorème de la théorie des fonctions | language=fr | year=1899 | journal=Acta Mathematica | issn=0001-5962 | volume=22 | pages=359–364 | issue=1 | doi=10.1007/BF02417878 | jfm=30.0364.02 | mr=1554908}}
{{citation | last=Ransford | first=Thomas | title=Potential theory in the complex plane | series=London Mathematical Society Student Texts | volume=28 | location=Cambridge | publisher=Cambridge University Press | year=1995 | isbn=0-521-46654-7 | zbl=0828.31001 }}

1 : Theorems in complex analysis

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。