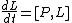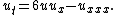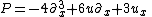1. Definition

2. Isospectral property
     Link with the inverse scattering method  

3. Example – Korteweg–de Vries

4. Example – Kovalevskaya

5. Equations with a Lax pair

6. References

In mathematics, in the theory of integrable systems, a Lax pair is a pair of time-dependent matrices or operators that satisfy a corresponding differential equation, called the Lax equation. Lax pairs were introduced by Peter Lax to discuss solitons in continuous media. The inverse scattering transform makes use of the Lax equations to solve such systems.

Definition

A Lax pair is a pair of matrices or operators 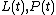dependent on time and acting on a fixed Hilbert space, and satisfying Lax's equation:

where 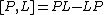is the commutator.

Often, as in the example below, depends on in a prescribed way, so this is a nonlinear equation for as a function of .

Isospectral property

It can then be shown that the eigenvalues and more generally the spectrum of L are independent of t. The matrices/operators L are said to be isospectral as varies.

The core observation is that the matrices 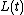are all similar by virtue of

where is the solution of the Cauchy problem

where I denotes the identity matrix. Note that if P(t) is skew-adjoint, U(t,s) will be unitary.

In other words, to solve the eigenvalue problem Lψ = λψ at time t, it is possible to solve the same problem at time 0 where L is generally known better, and to propagate the solution with the following formulas:

(no change in spectrum)

Link with the inverse scattering method

The above property is the basis for the inverse scattering method. In this method, L and P act on a functional space (thus ψ = ψ(t,x)), and depend on an unknown function u(t,x) which is to be determined. It is generally assumed that u(0,x) is known, and that P does not depend on u in the scattering region where .

The method then takes the following form:

1. Compute the spectrum of 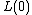, giving and ,
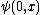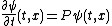2. In the scattering region where is known, propagate in time by using with initial condition ,
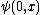3. Knowing in the scattering region, compute 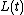and/or .

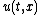Example – Korteweg–de Vries

The Korteweg–de Vries equation

can be reformulated as the Lax equation

with

(a Sturm–Liouville operator)

where all derivatives act on all objects to the right. This accounts for the infinite number of first integrals of the KdV equation.

Example – Kovalevskaya

The previous example used an infinite dimensional Hilbert space. Examples are also possible with finite dimensional Hilbert spaces. These include Kovalevskaya top and the generalization to include an electric Field 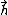.^[1]

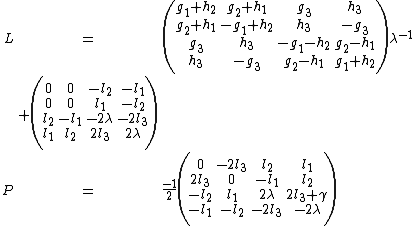
Equations with a Lax pair

Further examples of systems of equations that can be formulated as a Lax pair include:

• Benjamin–Ono equation
• One-dimensional cubic non-linear Schrödinger equation
• Davey–Stewartson system
• Integrable systems with contact Lax pairs^[2]
• Kadomtsev–Petviashvili equation
• Korteweg–de Vries equation
• KdV hierarchy
• Modified Korteweg–de Vries equation
• Sine-Gordon equation
• Toda lattice
• Lagrange, Euler, and Kovalevskaya tops

References

• {{citation|first=P.|last= Lax|title=Integrals of nonlinear equations of evolution and solitary waves|journal=Comm. Pure Applied Math.|volume=21|year=1968|pages= 467–490|doi=10.1002/cpa.3160210503|issue=5 }} [https://archive.org/details/integralsofnonli00laxp archive]
• P. Lax and R.S. Phillips, Scattering Theory for Automorphic Functions[https://projecteuclid.org/euclid.bams/1183546232], (1976) Princeton University Press.

• Sparse code
• Sparse coding
• Sparse disk image
• Sparse encoding
• Sparse equation
• Sparse files
• Sparse image
• .sparseimage format
• Sparse index
• Sparse language
• Sparsely-spotted stingaree
• Sparse matrix representation
• Sparse multicast
• Sparse set
• Sparse system
• Sparsetooth dogfish
• Sparsh
• Sparsha
• Sparsh (film)
• Sparsholt
• Sparsholt College
• Sparsholt College, Hampshire
• Sparsholt, Hampshire
• Sparsity of effects principle
• Sparsity-of-effects principle