1. Kodaira dimension
    Rational surfaces  Quadric surfaces  Rational cubic surfaces  Rational quartic surfaces  Other rational surfaces in space  Other families of rational surfaces  Non-rational ruled surfaces  Class VII surfaces 

2. Kodaira dimension
    K3 surfaces  Enriques surfaces  Abelian surfaces  Other classes of dimension- surfaces 

3. Kodaira dimension

4. Kodaira dimension (surfaces of general type)

5. Families of surfaces with members in multiple classes

6. See also

7. References

8. External links

This is a list of named algebraic surfaces, compact complex surfaces, and families thereof, sorted according to the Enriques–Kodaira classification.

Kodaira dimension

Rational surfaces

• Projective plane

Quadric surfaces

• Cone (geometry)
• Cylinder
• Ellipsoid
• Hyperboloid
• Paraboloid
• Sphere
• Spheroid

Rational cubic surfaces

• Cayley nodal cubic surface, a certain cubic surface with 4 nodes
• Cayley's ruled cubic surface
• Clebsch surface or Klein icosahedral surface
• Fermat cubic
• Monkey saddle
• Parabolic conoid
• Plücker's conoid
• Whitney umbrella

Rational quartic surfaces

• Châtelet surfaces
• Dupin cyclides, inversions of a cylinder, torus, or double cone in a sphere
• Gabriel's horn
• Right circular conoid
• Roman surface or Steiner surface, a realization of the real projective plane in real affine space
• Tori, surfaces of revolution generated by a circle about a coplanar axis

Other rational surfaces in space

• Boy's surface, a sextic realization of the real projective plane in real affine space
• Enneper surface, a nonic minimal surface
• Henneberg surface, a minimal surface of degree 15
• Bour's minimal surface, a surface of degree 16
• Richmond surfaces, a family of minimal surfaces of variable degree

Other families of rational surfaces

• Coble surfaces
• Del Pezzo surfaces, surfaces with an ample anticanonical divisor
• Hirzebruch surfaces, rational ruled surfaces
• Segre surfaces, intersections of two quadrics in projective 4-space
• Unirational surfaces of characteristic 0
• Veronese surface, the Veronese embedding of the projective plane into projective 5-space
• White surfaces, the blow-up of the projective plane at 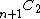points by the linear system of degree- curves through those points
  • Bordiga surfaces, the White surfaces determined by families of quartic curves

Non-rational ruled surfaces

Class VII surfaces

• Vanishing second Betti number:
  • Hopf surfaces
  • Inoue surfaces; several other families discovered by Inoue have also been called "Inoue surfaces"
• Positive second Betti number:
  • Enoki surfaces
  • Inoue–Hirzebruch surfaces
  • Kato surfaces

Kodaira dimension

K3 surfaces

• Kummer surfaces
  • Tetrahedroids, special Kummer surfaces
  • Wave surface, a special tetrahedroid
• Plücker surfaces, birational to Kummer surfaces
• Weddle surfaces, birational to Kummer surfaces
• Smooth quartic surfaces
• Supersingular K3 surfaces

Enriques surfaces

• Reye congruences, the locus of lines that lie on two out of three general quadric surfaces in projective space

Abelian surfaces

• Horrocks–Mumford surfaces, surfaces of degree 10 in projective 4-space that are the zero locus of sections of the rank-two Horrocks–Mumford bundle

Other classes of dimension- surfaces

• Non-classical Enriques surfaces, a variation on the notion of Enriques surfaces that only exist in characteristic two
• Hyperelliptic surfaces or bielliptic surfaces; quasi-hyperelliptic surfaces are a variation of this notion that exist only in characteristics two and three
• Kodaira surfaces

Kodaira dimension

• Dolgachev surfaces

Kodaira dimension (surfaces of general type)

• Barlow surfaces
• Beauville surfaces
• Burniat surfaces
• Campedelli surfaces; surfaces of general type with the same Hodge numbers as Campedelli surfaces are called numerical Campidelli surfaces
• Castelnuovo surfaces
• Catanese surfaces
• Fake projective planes or Mumford surfaces, surfaces with the same Betti numbers as projective plane but not isomorphic to it
• Fano surface of lines on a non-singular 3-fold; sometimes, this term is taken to mean del Pezzo surface
• Godeaux surfaces; surfaces of general type with the same Hodge numbers as Godeaux surfaces are called numerical Godeaux surfaces
• Horikawa surfaces
• Todorov surfaces

Families of surfaces with members in multiple classes

• Surfaces that are also Shimura varieties:
  • Hilbert modular surfaces
  • Humbert surfaces
  • Picard modular surfaces
  • Shioda modular surfaces
• Elliptic surfaces, surfaces with an elliptic fibration; quasielliptic surfaces constitute a modification this idea that occurs in finite characteristic
  • Raynaud surfaces and generalized Raynaud surfaces, certain quasielliptic counterexamples to the conclusions of the Kodaira vanishing theorem
• Exceptional surfaces, surfaces whose Picard number achieve the bound set by the central Hodge number h^1,1
• Kähler surfaces, complex surfaces with a Kähler metric; equivalently, surfaces for which the first Betti number b₁ is even
• Minimal surfaces, surfaces that can't be obtained from another by blowing up at a point; they have no connection with the minimal surfaces of differential geometry
• Nodal surfaces, surfaces whose only singularities are nodes
  • Cayley's nodal cubic, which has 4 nodes
  • Kummer surfaces, quartic surfaces with 16 nodes
  • Togliatti surface, a certain quintic with 31 nodes
  • Barth surfaces, referring to a certain sextic with 65 nodes and decic with 345 nodes
  • Labs surface, a certain septic with 99 nodes
  • Endrass surface, a certain surface of degree 8 with 168 nodes
  • Sarti surface, a certain surface of degree 12 with 600 nodes
• Quotient surfaces, surfaces that are constructed as the orbit space of some other surface by the action of a finite group; examples include Kummer, Godeaux, Hopf, and Inoue surfaces
• Zariski surfaces, surfaces in finite characteristic that admit a purely inseparable dominant rational map from the projective plane

See also

• Enriques–Kodaira classification
• List of surfaces

References

• Compact Complex Surfaces by Wolf P. Barth, Klaus Hulek, Chris A.M. Peters, Antonius Van de Ven {{isbn|3-540-00832-2}}
• Complex algebraic surfaces by Arnaud Beauville, {{isbn|0-521-28815-0}}

External links

• Mathworld has a long list of algebraic surfaces with pictures.
• Some more pictures of algebraic surfaces, especially ones with many nodes.
• Pictures of algebraic surfaces by Herwig Hauser.
• Free program SURFER to visualize algebraic surfaces in real-time, including a user gallery.

• Dichomeris latipalpis
• Dichomeris latipennella
• Dichomeris lativalvata
• Dichomeris legnotoa
• Dichomeris leontovitchi
• Dichomeris leptosaris
• Dichomeris lespedezae
• Dichomeris leucocosma
• Dichomeris leuconotella
• Dichomeris leucostena
• Dichomeris leucothicta
• Dichomeris levigata
• Dichomeris levisella
• Dichomeris ligulacea
• Dichomeris ligyra
• Dichomeris limbipunctella
• Dichomeris limbipunctellus
• Dichomeris limitellus
• Dichomeris limosella
• Dichomeris limosellus
• Dichomeris linealis
• Dichomeris lissota
• Dichomeris litoxyla
• Dichomeris liui
• Dichomeris lividula