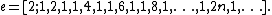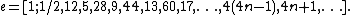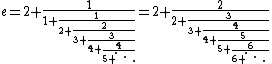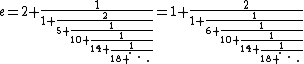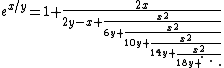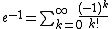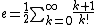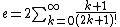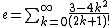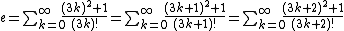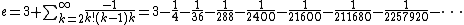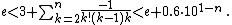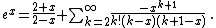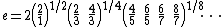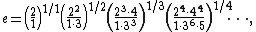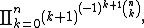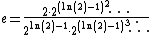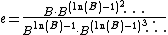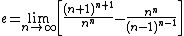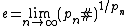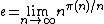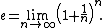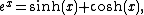- As a continued fraction
- As an infinite series
- As an infinite product
- As the limit of a sequence
- In trigonometry
- Notes
The mathematical constant {{math|e}} can be represented in a variety of ways as a real number. Since {{math|e}} is an irrational number (see proof that e is irrational), it cannot be represented as the quotient of two integers, but it can be represented as a continued fraction. Using calculus, {{math|e}} may also be represented as an infinite series, infinite product, or other sort of limit of a sequence.
As a continued fraction
Euler proved that the number {{math|e}} is represented as the infinite simple continued fraction[1] {{OEIS|id=A003417}}:
Its convergence can be tripled{{clarify|reason=By what measure?|date = April 2017}}{{cn|date = April 2017}} by allowing just one fractional number:
Here are some infinite generalized continued fraction expansions of {{math|e}}. The second is generated from the first by a simple equivalence transformation.
This last, equivalent to [1; 0.5, 12, 5, 28, 9, ...], is a special case of a general formula for the exponential function:
As an infinite series
The number {{math|e}} can be expressed as the sum of the following infinite series:
for any real number x.
In the special case where x = 1 or −1, we have:
,[2] and
Other series include the following:
[3]
where
is the {{mvar|n}}th Bell number.
Consideration of how to put upper bounds on e leads to this descending series:
which gives at least one correct (or rounded up) digit per term. That is, if 1 ≤ n, then
More generally, if x is not in {2, 3, 4, 5, ...}, then
As an infinite product
The number {{math|e}} is also given by several infinite product forms including Pippenger's product
and Guillera's product [4][5]
where the nth factor is the nth root of the product
as well as the infinite product
More generally, if 1 < B < e2 (which includes B = 2, 3, 4, 5, 6, or 7), then
As the limit of a sequence
The number {{math|e}} is equal to the limit of several infinite sequences:
and
(both by Stirling's formula).
The symmetric limit,[6]
may be obtained by manipulation of the basic limit definition of {{math|e}}.
The next two definitions are direct corollaries of the prime number theorem[7]
where 
is the nth prime and 
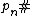is the primorial of the nth prime.
where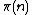 
is the prime counting function.
Also:
In the special case that 
, the result is the famous statement:
In trigonometry
Trigonometrically, {{math|e}} can be written in terms of the sum of two hyperbolic functions,
at {{math|1=x = 1}}.
Notes
2. ^{{cite web|url=http://oakroadsystems.com/math/loglaws.htm|title=It's the Law Too — the Laws of Logarithms|last=Brown|first=Stan|date=2006-08-27|publisher=Oak Road Systems|accessdate=2008-08-14|deadurl=yes|archiveurl=https://web.archive.org/web/20080813175402/http://oakroadsystems.com/math/loglaws.htm|archivedate=2008-08-13|df=}}
3. ^Formulas 2–7: H. J. Brothers, Improving the convergence of Newton's series approximation for e, The College Mathematics Journal, Vol. 35, No. 1, (2004), pp. 34–39.
4. ^J. Sondow, [https://arxiv.org/abs/math/0401406 A faster product for pi and a new integral for ln pi/2,] Amer. Math. Monthly 112 (2005) 729–734.
5. ^J. Guillera and J. Sondow, [https://arxiv.org/abs/math.NT/0506319 Double integrals and infinite products for some classical constants via analytic continuations of Lerch's transcendent,]Ramanujan Journal 16 (2008), 247–270.
6. ^H. J. Brothers and J. A. Knox, New closed-form approximations to the Logarithmic Constant e, The Mathematical Intelligencer, Vol. 20, No. 4, (1998), pp. 25–29.
7. ^S. M. Ruiz 1997
- Miguel Ángel Toma
- Miguel Ángel Álvarez
- Miguelópolis
- Mig welding
- MIG welding
- MIGY
- MIH
- Mi/h
- Miha Baloh
- Miha Brejc
- Mihael Brejc
- Mihael Milunovic
- Mihael milunovic
- Mihael Milunović
- Mihai Antonescu
- Mihai Bravu metro station
- Mihai Eminescu
- Mihai Găinușă
- Mihai I
- Mihai I of Romania
- Mihail Bulgakov
- Mihail Christodoulou Mouskos
- Mihail Eminescu
- Mihail Gorbachev
- Mihail Kogalniceanu