- Explanation
- Experimental evidence
- See also
- References
The Mikheyev–Smirnov–Wolfenstein effect (often referred to as matter effect) is a particle physics process which can act to modify neutrino oscillations in matter. Works in 1978 and 1979 by American physicist Lincoln Wolfenstein led to understanding that the oscillation parameters of neutrino are changed in matter. In 1985, the Soviet physicists Stanislav Mikheyev and Alexei Smirnov predicted that slow decrease of the matter density can resonantly enhance the neutrino mixing.{{sfn|Chela-Flores|2011|p=305}} Later in 1986, Stephen Parke of Fermilab, Hans Bethe of Cornell University, and S. Peter Rosen and James Gelb of Los Alamos National Laboratory provided analytic treatments of this effect.
Explanation
The presence of electrons in matter changes the energy levels of the propagation eigenstates (mass eigenstates) of neutrinos due to charged current coherent forward scattering of the electron neutrinos (i.e., weak interactions). The coherent forward scattering is analogous to the electromagnetic process leading to the refractive index of light in a medium. This means that neutrinos in matter have a different effective mass than neutrinos in vacuum, and since neutrino oscillations depend upon the squared mass difference of the neutrinos, neutrino oscillations may be different in matter than they are in vacuum. With antineutrinos, the conceptual point is the same but the effective charge that the weak interaction couples to (called weak isospin) has an opposite sign. If the electron density of matter changes along the path of neutrinos, the mixing of neutrinos grows to maximum at some value of the density, and then turns back; it leads to resonant conversion of one type of neutrinos to another one.
The effect is important at the very large electron densities of the Sun where electron neutrinos are produced. The high-energy neutrinos seen, for example, in Sudbury Neutrino Observatory (SNO) and in Super-Kamiokande, are produced mainly as the higher mass eigenstate in matter ν2, and remain as such as the density of solar material changes.[1] Thus, the neutrinos of high energy leaving the Sun are in a vacuum propagation eigenstate, ν2, that has a reduced overlap with the electron neutrino  seen by charged current reactions in the detectors.
seen by charged current reactions in the detectors.
Experimental evidence
For high-energy solar neutrinos the MSW effect is important, and leads to the expectation that 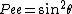 , where
, where  is the solar mixing angle. This was dramatically confirmed in the Sudbury Neutrino Observatory (SNO), which has resolved the solar neutrino problem. SNO measured the flux of Solar electron neutrinos to be ~34% of the total neutrino flux (the electron neutrino flux measured via the charged current reaction, and the total flux via the neutral current reaction). The SNO results agree well with the expectations. Earlier, Kamiokande and Super-Kamiokande measured a mixture of charged current and neutral current reactions, that also support the occurrence of the MSW effect with a similar suppression, but with less confidence.
is the solar mixing angle. This was dramatically confirmed in the Sudbury Neutrino Observatory (SNO), which has resolved the solar neutrino problem. SNO measured the flux of Solar electron neutrinos to be ~34% of the total neutrino flux (the electron neutrino flux measured via the charged current reaction, and the total flux via the neutral current reaction). The SNO results agree well with the expectations. Earlier, Kamiokande and Super-Kamiokande measured a mixture of charged current and neutral current reactions, that also support the occurrence of the MSW effect with a similar suppression, but with less confidence.
For the low-energy solar neutrinos, on the other hand, the matter effect is negligible, and the formalism of oscillations in vacuum is valid. The size of the source (i.e. the Solar core) is significantly larger than the oscillation length, therefore, averaging over the oscillation factor, one obtains 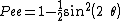 . For θ = 34° this corresponds to a survival probability of Pee ≈ 60%. This is consistent with the experimental observations of low energy Solar neutrinos by the Homestake experiment (the first experiment to reveal the solar neutrino problem), followed by GALLEX, GNO, and SAGE (collectively, gallium radiochemical experiments), and, more recently, the Borexino experiment, which observed the neutrinos from pp (< 420 keV), 7Be (862 keV), pep (1.44 MeV), and 8B (< 15 MeV) separately. The measurements of Borexino alone verify the MSW pattern; however all these experiments are consistent with each other and provide us strong evidence of the MSW effect.
. For θ = 34° this corresponds to a survival probability of Pee ≈ 60%. This is consistent with the experimental observations of low energy Solar neutrinos by the Homestake experiment (the first experiment to reveal the solar neutrino problem), followed by GALLEX, GNO, and SAGE (collectively, gallium radiochemical experiments), and, more recently, the Borexino experiment, which observed the neutrinos from pp (< 420 keV), 7Be (862 keV), pep (1.44 MeV), and 8B (< 15 MeV) separately. The measurements of Borexino alone verify the MSW pattern; however all these experiments are consistent with each other and provide us strong evidence of the MSW effect.
These results are further supported by the reactor experiment KamLAND, that is uniquely able to measure the parameters of oscillation that are also consistent with all other measurements.
The transition between the low energy regime (the MSW effect is negligible) and the high energy regime (the oscillation probability is determined by matter effects) lies in the region of about 2 MeV for the Solar neutrinos.
The MSW effect can also modify neutrino oscillations in the Earth, and future search for new oscillations and/or leptonic CP violation may make use of this property.
See also
- Neutrino oscillations
References
Citations- {{cite book
|last=Chela-Flores |first=J.
|year=2011
|title=The Science of Astrobiology
|url=https://books.google.com/books?id=BnyjmtIR7McC&pg=PA305
|pages=
|publisher=Springer Science & Business Media
|isbn=9789400716278
|ref=harv
}}
- {{cite journal
|last1=Schwarzschild |first1=B.
|year=2003
|title=Antineutrinos From Distant Reactors Simulate the Disappearance of Solar Neutrinos
|url=http://grattalab3.stanford.edu/neutrino/KamLAND/ArticlesAboutKamLAND/KL_PhysTodArt.html
|journal=Physics Today
|volume=56 |issue=3 |pages=14-16
|bibcode=2003PhT....56c..14S
|doi=10.1063/1.1570758
}}
- {{cite web
|last=Brooijmans |first=G.
|date=28 July 1998
|title=Neutrino Oscillations in Matter: the MSW Effect
|website=A New Limit on νμ → ντ Oscillations
|page=40
|publisher=Université catholique de Louvain
}}
- {{cite journal
|last1=Mikheyev |first1=S. P.
|last2=Smirnov |first2=A. Yu.
|year=1985
|title=Resonance enhancement of oscillations in matter and solar neutrino spectroscopy
|journal=Soviet Journal of Nuclear Physics
|volume=42 |issue=6 |pages=913–917
|bibcode=1985YaFiz..42.1441M
|doi=
}}
- {{cite journal
|last1=Wolfenstein |first1=L.
|year=1978
|title=Neutrino oscillations in matter
|journal=Physical Review D
|volume=17 |issue=9 |pages=2369-2374
|bibcode=1978PhRvD..17.2369W
|doi=10.1103/PhysRevD.17.2369
}}
- {{cite journal
|last1=Wolfenstein |first1=L.
|year=1979
|title=Neutrino oscillations and stellar collapse
|journal=Physical Review D
|volume=20 |issue=10 |pages=2634–2635
|bibcode=1979PhRvD..20.2634W
|doi=10.1103/PhysRevD.20.2634
}}
- {{cite journal
|last1=Parke |first1=S. J.
|year=1986
|title=Nonadiabatic level crossing in resonant neutrino oscillations
|journal=Physical Review Letters
|volume=57 |issue=10 |pages=1275–1278
|bibcode=1986PhRvL..57.1275P
|doi=10.1103/PhysRevLett.57.1275
|pmid=10033402
}}
- {{cite journal
|last1=Bethe |first1=H. A.
|year=1986
|title=Possible explanation of the solar-neutrino puzzle
|journal=Physical Review Letters
|volume=56 |issue=12 |pages=1305–1308
|bibcode=1986PhRvL..56.1305B
|doi=10.1103/PhysRevLett.56.1305
|pmid=10032627
- {{cite journal
|last1=Rosen |first1=S. P.
|last2=Gelb |first2=J. M.
|year=1986
|title=Mikheyev-Smirnov-Wolfenstein enhancement of oscillations as a possible solution to the solar-neutrino problem
|journal=Physical Review D
|volume=34 |issue=4 |pages=969–979
|bibcode=1986PhRvD..34..969R
|doi=10.1103/PhysRevD.34.969
|url=https://zenodo.org/record/1233781
|format=Submitted manuscript{{refend}}{{DEFAULTSORT:Mikheyev-Smirnov-Wolfenstein effect}}
- World War II reparations
- World War II series
- World War II ship camouflage measures of the United States Navy
- World War II songs
- World War II Tunnels
- World War II U.S. Military Sex Education
- World War II Veterans Memorial State Park
- World war II veterans still alive
- World War II Victory Matza
- World War I literature
- World War I logistics
- World War I medal abbreviations
- World War I Memorial (Berwick, Pennsylvania)
- World War I Memorial Bridge
- World War I Memorial Carillon
- World War I Memorial Flagpole (Hawkins)
- World War I memorials
- World War I Memorials and Cemeteries in Alsace
- World War I Memorial (Tiruchirappalli)
- World War I Memorial (Washington, DC)
- World War I Memorial (Washington, D.C.)
- World War I naval arms race
- World War I naval arms race (disambiguation)
- World War in France
- World War I novel