1. Opmonoidal Monads

2. Properties

3. Examples

4. References

In category theory, a monoidal monad is a monad on a monoidal category such that the functor is a lax monoidal functor and the natural transformations and are monoidal natural transformations. In other words, is equipped with coherence maps and satisfying certain properties, and the unit and multiplication are monoidal natural transformations. By monoidality of , the morphisms and are necessarily equal. This is equivalent to say that a monoidal monad is a monad in the 2-category 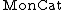of monoidal categories, lax monoidal functors, and monoidal natural transformations.

Opmonoidal Monads

Opmonoidal monads have been studied under various names. Ieke Moerdijk introduced them as "Hopf monads",^[1] while in works of Bruguières and Virelizier they are called "bimonads", by analogy to "bialgebra",^[2] reserving the term "Hopf monad" for opmonoidal monads with an antipode, in analogy to "Hopf algebras".

An opmonoidal monad is a monad in the 2-category of monoidal categories, oplax monoidal functors and monoidal natural transformations. That means a monad on a monoidal category together with coherence maps and satisfying three axioms that make an opmonoidal functor, and four more axioms that make the unit and the multiplication into opmonoidal natural transformations. Alternatively, an opmonoidal monad is a monad on a monoidal category such that the category of Eilenberg-Moore algebras has a monoidal structure for which the forgetful functor is strong monoidal.^[1][3]

An easy example for the monoidal category of vector spaces is the monad , where is a bialgebra.^[2] The multiplication and unit of define the multiplication and unit of the monad, while the comultiplication and counit of give rise to the opmonoidal structure. The algebras of this monad are right -modules, which one may tensor in the same way as their underlying vector spaces.

Properties

• The Kleisli category of a monoidal monad has a canonical monoidal structure, induced by the monoidal structure of the monad, and such that the free functor is strong monoidal. The canonical adjunction between and the Kleisli category is a monoidal adjunction with respect to this monoidal structure, this means that the 2-category 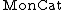has Kleisli objects for monads.
• The 2-category of monads in 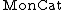is the 2-category of monoidal monads and it is isomorphic to the 2-category of monoidales (or pseudomonoids) in the category of monads , (lax) monoidal arrows between them and monoidal cells between them.^[4]
• The Eilenberg-Moore category#Algebras for a monad for an opmonoidal monad has a canonical monoidal structure such that the forgetful functor is strong monoidal.^[1] Thus, the 2-category has Eilenberg-Moore objects for monads.^[3]
• The 2-category of monads in is the 2-category of monoidal monads and it is isomorphic to the 2-category of monoidales (or pseudomonoids) in the category of monads opmonoidal arrows between them and opmonoidal cells between them.^[4]

Examples

The following monads on the category of sets, with its cartesian monoidal structure, are monoidal monads:

• The power set monad.
• The probability distributions (Giry) monad.

The following monads on the category of sets, with its cartesian monoidal structure, are not monoidal monads

• If 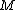is a monoid, then is a monad, but in general there is no reason to expect a monoidal structure on it (unless 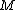is commutative).

References

• Marseilles-lès-Aubigny
• Marseilles Metro
• Marseilles, South Africa
• Marseilles Subway
• Marseilles Tarot
• Marseilles Township, Wyandot County, OH
• Marseille Subway
• Marseille Tramway
• Marseillette
• Marseille-Ventimiglia railway
• Marseillevirus
• Marseille XIII Avenir
• Marseille–Ventimiglia railway
• Marse Joe
• Mars Electric
• Mars electric
• Marsel Efroimski
• Marsel Ilhan
• Marselli Sumarno
• Marsel Rushani
• Marsel van Oosten
• Marsel İlhan
• Marsemina
• Marsenina
• Marsenina stearnsii