“Monotonically normal space”的意思、由来-开放百科全书

词条

Monotonically normal space

释义

Equivalent definitions
Definition 2 Definition 3 Definition 4
Properties
Some discussion links

In mathematics, a monotonically normal space is a particular kind of normal space, with some special characteristics, and is such that it is hereditarily normal, and any two separated subsets are strongly separated. They are defined in terms of a monotone normality operator.

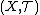A topological space is said to be monotonically normal if the following condition holds:

For every , where G is open, there is an open set 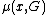such that

if 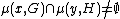then either or .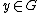

There are some equivalent criteria of monotone normality.

Equivalent definitions

Definition 2

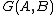A space X is called monotonically normal if it is and for each pair of disjoint closed subsets there is an open set with the properties

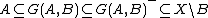and
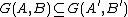, whenever 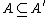and .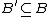

This operator is called monotone normality operator.

Note that if G is a monotone normality operator, then defined by 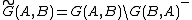is also a monotone normality operator; and satisfies

For this reason we some time take the monotone normality operator so as to satisfy the above requirement; and that facilitates the proof of some theorems and of the equivalence of the definitions as well.

Definition 3

A space X is called monotonically normal if it is ,and to each pair (A, B) of subsets of X, with 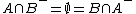, one can assign an open subset G(A, B) of X such that

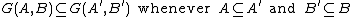Definition 4

A space X is called monotonically normal if it is and there is a function H that assigns to each ordered pair (p,C) where C is closed and p is without C, an open set H(p,C) satisfying:

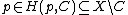
if D is closed and 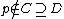then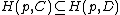
if are points in X, then .

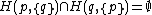Properties

An important example of these spaces would be, assuming Axiom of Choice, the linearly ordered spaces; however, it really needs axiom of choice for an arbitrary linear order to be normal (see van Douwen's paper). Any generalised metric is monotonically normal even without choice. An important property of monotonically normal spaces is that any two separated subsets are strongly separated there. Monotone normality is hereditary property and a monotonically normal space is always normal by the first condition of the second equivalent definition.

We list up some of the properties :

A closed map preserves monotone normality.
A monotonically normal space is hereditarily collectionwise normal.
Elastic spaces are monotonically normal.

Some discussion links

{{cite journal |last1=Heath |first1=R. W. |last2=Lutzer |first2=D. J. |last3=Zenor |first3=P. L. |date=April 1973 |title=Monotonically Normal Spaces |journal=Transactions of the American Mathematical Society |volume=178 |pages=481–493 |url=http://www.ams.org/tran/1973-178-00/S0002-9947-1973-0372826-2/S0002-9947-1973-0372826-2.pdf |doi=10.2307/1996713|jstor=1996713 }}
{{cite journal |last=Borges |first=Carlos R. |date=March 1973 |title=A Study of Monotonically Normal Spaces |journal=Proceedings of the American Mathematical Society |volume=38 |number=1 |pages=211–214 |url=http://www.ams.org/proc/1973-038-01/S0002-9939-1973-0324644-4/S0002-9939-1973-0324644-4.pdf |doi=10.2307/2038799|jstor=2038799 }}
{{cite journal |last=van Douwen |first=Eric K. |authorlink=Eric van Douwen |date=September 1985 |title=Horrors of Topology Without AC: A Nonnormal Orderable Space |journal=Proceedings of the American Mathematical Society |volume=95 |number=1 |pages=101–105 |url=http://www.ams.org/proc/1985-095-01/S0002-9939-1985-0796455-5/S0002-9939-1985-0796455-5.pdf |doi=10.2307/2045582|jstor=2045582 }}
{{cite journal |last=Gartside |first=P. M. |date=1997 |title=Cardinal Invariants of Monotonically Normal Spaces |journal=Topology and its Applications |volume=77 |issue=3 |pages=303–314 |doi=10.1016/s0166-8641(96)00086-7}}
Henno Brandsma's discussion about Monotone Normality in Topology Atlas can be viewed here

3 : Topology|Separation axioms|Properties of topological spaces

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。