- Construction
- Iterated Mycielskians
- Properties
- Cones over graphs
- References
- External links
In the mathematical area of graph theory, the Mycielskian or Mycielski graph of an undirected graph is a larger graph formed from it by a construction of {{harvs|first=Jan|last=Mycielski|authorlink=Jan Mycielski|year=1955|txt}}. The construction preserves the property of being triangle-free but increases the chromatic number; by applying the construction repeatedly to a triangle-free starting graph, Mycielski showed that there exist triangle-free graphs with arbitrarily large chromatic number.
Construction
Let the n vertices of the given graph G be v1, v2, . . . , vn. The Mycielski graph μ(G) contains G itself as a subgraph, together with n+1 additional vertices: a vertex ui corresponding to each vertex vi of G, and an extra vertex w. Each vertex ui is connected by an edge to w, so that these vertices form a subgraph in the form of a star K1,n. In addition, for each edge vivj of G, the Mycielski graph includes two edges, uivj and viuj.
Thus, if G has n vertices and m edges, μ(G) has 2n+1 vertices and 3m+n edges.
The only new triangles in μ(G) are of the form vivjuk, where vivjvk is a triangle in G. Thus, if G is triangle-free, so is μ(G).
To see that the construction increases the chromatic number 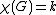 , consider a proper k-coloring of
, consider a proper k-coloring of 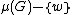 ; that is, a mapping
; that is, a mapping 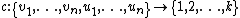 with
with 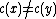 for adjacent vertices x,y. If we had
for adjacent vertices x,y. If we had 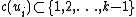 for all i, then we could define a proper (k−1)-coloring of G by
for all i, then we could define a proper (k−1)-coloring of G by  when
when  , and
, and 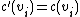 otherwise. But this is impossible for
otherwise. But this is impossible for 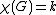 , so c must use all k colors for
, so c must use all k colors for 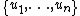 , and any proper coloring of the last vertex w must use an extra color. That is,
, and any proper coloring of the last vertex w must use an extra color. That is, 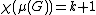 .
.
Iterated Mycielskians
Applying the Mycielskian repeatedly, starting with the one-edge graph, produces a sequence of graphs Mi = μ(Mi−1), sometimes called the Mycielski graphs. The first few graphs in this sequence are the graph M2 = K2 with two vertices connected by an edge, the cycle graph M3 = C5, and the Grötzsch graph M4 with 11 vertices and 20 edges.
In general, the graph Mi is triangle-free, (i−1)-vertex-connected, and i-chromatic. The number of vertices in Mi for i ≥ 2 is 3 × 2i−2 − 1 {{OEIS|id=A083329}}, while the number of edges for i = 2, 3, . . . is:
1, 5, 20, 71, 236, 755, 2360, 7271, 22196, 67355, ... {{OEIS|id=A122695}}.
Properties
- If G has chromatic number k, then μ(G) has chromatic number k + 1 {{harv|Mycielski|1955}}.
- If G is triangle-free, then so is μ(G) {{harv|Mycielski|1955}}.
- More generally, if G has clique number ω(G), then μ(G) has clique number max(2,ω(G)).{{harv|Mycielski|1955}}.
- If G is a factor-critical graph, then so is μ(G) {{harv|Došlić|2005}}. In particular, every graph Mi for i ≥ 2 is factor-critical.
- If G has a Hamiltonian cycle, then so does μ(G) {{harv|Fisher|McKenna|Boyer|1998}}.
- If G has domination number γ(G), then μ(G) has domination number γ(G)+1. {{harv|Fisher|McKenna|Boyer|1998}}.
Cones over graphs
A generalization of the Mycielskian, called a cone over a graph, was introduced by {{harvtxt|Stiebitz|1985}} and further studied by {{harvtxt|Tardif|2001}} and {{harvtxt|Lin|Wu|Lam|Gu|2006}}. In this construction, one forms a graph Δi(G) from a given graph G by taking the tensor product of graphs G × H, where H is a path of length i with a self-loop at one end, and then collapsing into a single supervertex all of the vertices associated with the vertex of H at the non-loop end of the path. The Mycielskian itself can be formed in this way as μ(G) = Δ2(G).
While the cone construction does not always increase the chromatic number, {{harvtxt|Stiebitz|1985}} proved that it does so when applied iteratively to K2. That is, define a sequence of families of graphs, called generalized Mycielskians, as
ℳ(2) = {K2} and ℳ(k+1) = {Δi(G) | G ∈ ℳ(k), i ∈ ℕ}.
For example, ℳ(3) is the family of odd cycles. Then each graph in ℳ(k) is k-chromatic. The proof uses methods of topological combinatorics developed by László Lovász to compute the chromatic number of Kneser graphs.
The triangle-free property is then strengthened as follows: if one only applies the cone construction Δi for i ≥ r, then the resulting graph has odd girth at least 2r + 1, that is, it contains no odd cycles of length less than 2r + 1.
Thus generalized Mycielskians provide a simple construction of graphs with high chromatic number and high odd girth.
References
- {{citation
| last = Chvátal | first = Vašek | author-link = Václav Chvátal
| contribution = The minimality of the Mycielski graph
| pages = 243–246
| publisher = Springer-Verlag
| series = Lecture Notes in Mathematics
| title = Graphs and Combinatorics (Proc. Capital Conf., George Washington Univ., Washington, D.C., 1973)
| volume = 406
| year = 1974}}.
- {{citation
| last = Došlić | first = Tomislav
| doi = 10.7151/dmgt.1279
| issue = 3
| journal = Discussiones Mathematicae Graph Theory
| mr = 2232992
| pages = 261–266
| title = Mycielskians and matchings
| volume = 25
| year = 2005}}.
- {{citation
| last1 = Fisher | first1 = David C.
| last2 = McKenna | first2 = Patricia A.
| last3 = Boyer | first3 = Elizabeth D.
| doi = 10.1016/S0166-218X(97)00126-1
| issue = 1–3
| journal = Discrete Applied Mathematics
| pages = 93–105
| title = Hamiltonicity, diameter, domination, packing, and biclique partitions of Mycielski's graphs
| volume = 84
| year = 1998}}.
- {{citation
| last1 = Lin | first1 = Wensong
| last2 = Wu | first2 = Jianzhuan
| last3 = Lam | first3 = Peter Che Bor
| last4 = Gu | first4 = Guohua
| doi = 10.1016/j.dam.2005.11.001
| issue = 8
| journal = Discrete Applied Mathematics
| pages = 1173–1182
| title = Several parameters of generalized Mycielskians
| volume = 154
| year = 2006}}.
- {{citation
| last = Mycielski | first = Jan | author-link = Jan Mycielski
| journal = Colloq. Math.
| pages = 161–162
| title = Sur le coloriage des graphes
| url = http://matwbn.icm.edu.pl/ksiazki/cm/cm3/cm3119.pdf
| volume = 3
| year = 1955}}.
- {{citation
| last = Stiebitz | first = M.
| publisher = Habilitation thesis, Technische Universität Ilmenau
| title = Beiträge zur Theorie der färbungskritschen Graphen
| year = 1985}}. As cited by {{harvtxt|Tardif|2001}}.
- {{citation
| last = Tardif | first = C.
| doi = 10.1002/jgt.1025
| issue = 2
| journal = Journal of Graph Theory
| pages = 87–94
| title = Fractional chromatic numbers of cones over graphs
| volume = 38
| year = 2001}}.
External links
- {{mathworld | title = Mycielski Graph | urlname = MycielskiGraph}}
- Cloud About Mercury
- Cloud Aerosol Transport System
- Cloud analytics
- Cloud Application Management for Platforms
- Cloud arch
- Cloud Arch
- Cloud architecture
- Cloud Atlas (2012 film)
- Cloud atlas (film)
- Cloud Atlas (film)
- Cloud Backup
- Cloud-based
- Cloud-based computing
- Cloud-based design and manufacturing
- Cloud-Based Design and Manufacturing
- Cloud-based integration
- Cloud Based Integration
- Cloud-based lending
- Cloud-based simulation
- Cloud based storage
- Cloudbaser
- Cloudbaser trike
- Cloudbaser Trikes Cloudbaser
- CloudBees
- Cloudberry Jam