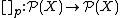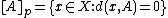“Preclosure operator”的意思、由来-开放百科全书

词条

Preclosure operator

释义

Definition
Topology
Examples
Premetrics Sequential spaces
See also
References

In topology, a preclosure operator, or Čech closure operator is a map between subsets of a set, similar to a topological closure operator, except that it is not required to be idempotent. That is, a preclosure operator obeys only three of the four Kuratowski closure axioms.

Definition

A preclosure operator on a set is a map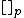

where is the power set of .

The preclosure operator has to satisfy the following properties:

(Preservation of nullary unions);
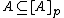(Extensivity);
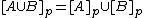(Preservation of binary unions).

The last axiom implies the following:

4. implies .

Topology

A set is closed (with respect to the preclosure) if 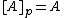. A set 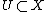is open (with respect to the preclosure) if 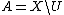is closed. The collection of all open sets generated by the preclosure operator is a pretopology.

Examples

Premetrics

Given a premetric on , then

is a preclosure on .

Sequential spaces

The sequential closure operator 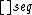is a preclosure operator. Given a topology with respect to which the sequential closure operator is defined, the topological space 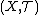is a sequential space if and only if the topology generated by 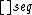is equal to , that is, if .

References

A.V. Arkhangelskii, L.S.Pontryagin, General Topology I, (1990) Springer-Verlag, Berlin. {{ISBN|3-540-18178-4}}.
B. Banascheski, Bourbaki's Fixpoint Lemma reconsidered, Comment. Math. Univ. Carolinae 33 (1992), 303-309.

1 : Closure operators

随便看