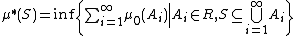“Pre-measure”的意思、由来-开放百科全书

词条

Pre-measure

释义

Definition
Extension theorem
See also
References

In mathematics, a pre-measure is a function that is, in some sense, a precursor to a bona fide measure on a given space. Indeed, one of the fundamental theorems in measure theory states that a pre-measure can be extended to a measure.

Definition

Let R be a ring of subsets (closed under union and relative complement) of a fixed set X and let μ₀: R → [0, +∞] be a set function. μ₀ is called a pre-measure if

and, for every countable (or finite) sequence {A_n}_n∈N ⊆ R of pairwise disjoint sets whose union lies in R,

.

The second property is called σ-additivity.

Thus, what is missing for a pre-measure to be a measure is that it is not necessarily defined on a sigma-algebra (or a sigma-ring).

Extension theorem

It turns out that pre-measures give rise quite naturally to outer measures, which are defined for all subsets of the space X. More precisely, if μ₀ is a pre-measure defined on a ring of subsets R of the space X, then the set function μ^∗ defined by

is an outer measure on X and the measure μ induced by μ^∗ on the σ-algebra Σ of Carathéodory-measurable sets satisfies 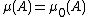for (in particular, Σ includes R). The infimum of the empty set is taken to be .

(Note that there is some variation in the terminology used in the literature. For example, Rogers (1998) uses "measure" where this article uses the term "outer measure". Outer measures are not, in general, measures, since they may fail to be σ-additive.)

References

{{cite book

}} {{MathSciNet|id=0053186}}

{{cite book

}} {{MathSciNet|id=1692618}} (See section 1.2.)

{{cite book

| author = Folland, G. B.
| title = Real Analysis
| edition = Second
| series = Pure and Applied Mathematics
| publisher = John Wiley & Sons, Inc
| location = New York
| year = 1999
| pages = 30–31
| isbn = 0-471-31716-0
}}

1 : Measures (measure theory)

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。

Definition

Extension theorem

See also

References