- Cartesian coordinates
- Images
- Symmetry constructions
- Alternate names
- Related uniform polytopes
- References
| Rectified 24-cell | ||
Schlegel diagram 8 of 24 cuboctahedral cells shown | ||
| Type | Uniform 4-polytope | |
| Schläfli symbols | r{3,4,3} = 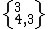 rr{3,3,4}= 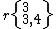 r{31,1,1} = 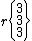 | |
| Coxeter diagrams | {{CDD|node|3|node_1|4|node|3|node}} {{CDD|node_1|3|node|3|node_1|4|node}} {{CDD|node_1|3|node|split1|nodes_11}} or {{CDD|node|splitsplit1|branch3_11|node_1}} | |
| Cells | 48 | 24 3.4.3.4 24 4.4.4 |
| Faces | 240 | 96 {3} 144 {4} |
| Edges | 288 | |
| Vertices | 96 | |
| Vertex figure | Triangular prism | |
| Symmetry groups | F4 [3,4,3], order 1152 B4 [3,3,4], order 384 D4 [31,1,1], order 192 | |
| Properties | convex, edge-transitive | |
| Uniform index | 22 23 24 | |
In geometry, the rectified 24-cell or rectified icositetrachoron is a uniform 4-dimensional polytope (or uniform 4-polytope), which is bounded by 48 cells: 24 cubes, and 24 cuboctahedra. It can be obtained by reducing the 24-cell's cells to cubes or cuboctahedra.
E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as tC24.
It can also be considered a cantellated 16-cell with the lower symmetries B4 = [3,3,4]. B4 would lead to a bicoloring of the cuboctahedral cells into 8 and 16 each. It is also called a runcicantellated demitesseract in a D4 symmetry, giving 3 colors of cells, 8 for each.
Cartesian coordinates
A rectified 24-cell having an edge length of {{radic|2}} has vertices given by all permutations and sign permutations of the following Cartesian coordinates:
(0,1,1,2) [4!/2!×23 = 96 vertices]
The dual configuration with edge length 2 has all coordinate and sign permutations of:
(0,2,2,2) [4×23 = 32 vertices]
(1,1,1,3) [4×24 = 64 vertices]
Images
{{24-cell_4-cube_Coxeter_plane_graphs|t1|100|t2}}| Stereographic projection | |
|---|---|
| Center of stereographic projection with 96 triangular faces blue | |
Symmetry constructions
There are three different symmetry constructions of this polytope. The lowest  construction can be doubled into
construction can be doubled into 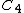 by adding a mirror that maps the bifurcating nodes onto each other.
by adding a mirror that maps the bifurcating nodes onto each other.  can be mapped up to
can be mapped up to 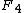 symmetry by adding two mirror that map all three end nodes together.
symmetry by adding two mirror that map all three end nodes together.
The vertex figure is a triangular prism, containing two cubes and three cuboctahedra. The three symmetries can be seen with 3 colored cuboctahedra in the lowest  construction, and two colors (1:2 ratio) in
construction, and two colors (1:2 ratio) in 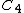 , and all identical cuboctahedra in
, and all identical cuboctahedra in 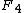 .
.
| Coxeter group | 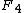 = [3,4,3] = [3,4,3] | 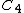 = [4,3,3] = [4,3,3] |  = [3,31,1] = [3,31,1] |
|---|---|---|---|
| Order | 1152 | 384 | 192 |
| Full symmetry group | [3,4,3] | [4,3,3] | <[3,31,1]> = [4,3,3] [3[31,1,1]] = [3,4,3] |
| Coxeter diagram | node|3|node_1|4|node|3|node}} | node|4|node_1|3|node|3|node_1}} | nodes_11|split2|node|3|node_1}} |
| Facets | 3: {{CDD>node|3|node_1|4|node}} 2: {{CDD|node_1|4|node|3|node}} | 2,2: {{CDD>node_1|3|node|3|node_1}} 2: {{CDD|node|4|node_1|2|node_1}} | 1,1,1: {{CDD>node_1|3|node|3|node_1}} 2: {{CDD|node_1|2|node_1|2|node_1}} |
| Vertex figure |
Alternate names
- Rectified 24-cell, Cantellated 16-cell (Norman Johnson)
- Rectified icositetrachoron (Acronym rico) (George Olshevsky, Jonathan Bowers)
- Cantellated hexadecachoron
- Disicositetrachoron
- Amboicositetrachoron (Neil Sloane & John Horton Conway)
Related uniform polytopes
{{Demitesseract family}}{{24-cell_family}}The rectified 24-cell can also be derived as a cantellated 16-cell:
{{Tesseract family}}References
- T. Gosset: On the Regular and Semi-Regular Figures in Space of n Dimensions, Messenger of Mathematics, Macmillan, 1900
- H.S.M. Coxeter:
- Coxeter, Regular Polytopes, (3rd edition, 1973), Dover edition, {{isbn|0-486-61480-8}}, p. 296, Table I (iii): Regular Polytopes, three regular polytopes in n-dimensions (n≥5)
- H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973, p. 296, Table I (iii): Regular Polytopes, three regular polytopes in n-dimensions (n≥5)
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, {{isbn|978-0-471-01003-6}}
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
- (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
- (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, {{isbn|978-1-56881-220-5}} (Chapter 26. pp. 409: Hemicubes: 1n1)
- Norman Johnson Uniform Polytopes, Manuscript (1991)
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. (1966)
- {{PolyCell | urlname = section2.html| title = 2. Convex uniform polychora based on the tesseract (8-cell) and hexadecachoron (16-cell) - Model 23}}
- {{PolyCell | urlname = section3.html| title = 3. Convex uniform polychora based on the icositetrachoron (24-cell) - Model 23}}
- {{PolyCell | urlname = section7.html| title = 7. Uniform polychora derived from glomeric tetrahedron B4 - Model 23 }}
- {{KlitzingPolytopes|polychora.htm|4D uniform polytopes (polychora)|o3x4o3o - rico}}
- Shabaam Sahdeeq rapper
- Shabaan
- Shabaan Abdul Raheem
- Shabab Al-Baydaa
- Shabab Al Hussein
- Shabab al-Moumineen
- Shabab Al Moumineen
- Shabab Al Mu'mineen
- Shabab al-Mumineen
- Shabab al-Muminin
- Shabab al-Muomineen
- Shabab Al-Ordon
- Shabab Al Ordon
- Shabab Al Ordon Al Qadisiya
- Shabab Al-Ordon Al-Qadisiya
- Shababeek
- Shabab emraa
- Shabab Emraa
- Shabab-ud-Din Shabban
- Shabad (disambiguation)
- Shabad (hymn)
- Shabadin
- Shaba (disambiguation)
- Shabad, Rangareddi district
- Shabad, Rangareddy district