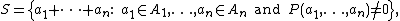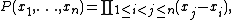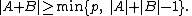- Cauchy–Davenport theorem
- Erdős–Heilbronn conjecture
- Combinatorial Nullstellensatz
- References
- External links
In additive number theory and combinatorics, a restricted sumset has the form
where  are finite nonempty subsets of a field F and
are finite nonempty subsets of a field F and  is a polynomial over F.
is a polynomial over F.
When  , S is the usual sumset
, S is the usual sumset  which is denoted by nA if
which is denoted by nA if  ; when
; when
S is written as  which is denoted by
which is denoted by  if
if  . Note that |S| > 0 if and only if there exist
. Note that |S| > 0 if and only if there exist  with
with  .
.
Cauchy–Davenport theorem
The Cauchy–Davenport theorem named after Augustin Louis Cauchy and Harold Davenport asserts that for any prime p and nonempty subsets A and B of the prime order cyclic group Z/pZ we have the inequality[1][2]
We may use this to deduce the Erdős–Ginzburg–Ziv theorem: given any sequence of 2n−1 elements in Z/n, there are n elements that sums to zero modulo n. (Here n does not need to be prime.)[3][4]
A direct consequence of the Cauchy-Davenport theorem is: Given any set S of p−1 or more nonzero elements, not necessarily distinct, of Z/pZ, every element of Z/pZ can be written as the sum of the elements of some subset (possibly empty) of S.[5]
Kneser's theorem generalises this to finite abelian groups.[6]Erdős–Heilbronn conjecture
The Erdős–Heilbronn conjecture posed by Paul Erdős and Hans Heilbronn in 1964 states that  if p is a prime and A is a nonempty subset of the field Z/pZ.[7] This was first confirmed by J. A. Dias da Silva and Y. O. Hamidoune in 1994[8]
if p is a prime and A is a nonempty subset of the field Z/pZ.[7] This was first confirmed by J. A. Dias da Silva and Y. O. Hamidoune in 1994[8]
who showed that
where A is a finite nonempty subset of a field F, and p(F) is a prime p if F is of characteristic p, and p(F) = ∞ if F is of characteristic 0. Various extensions of this result were given by Noga Alon, M. B. Nathanson and I. Ruzsa in 1996,[9] Q. H. Hou and Zhi-Wei Sun in 2002,[9]
and G. Karolyi in 2004.[10]
Combinatorial Nullstellensatz
A powerful tool in the study of lower bounds for cardinalities of various restricted sumsets is the following fundamental principle: the combinatorial Nullstellensatz.[11] Let 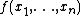 be a polynomial over a field F. Suppose that the coefficient of the monomial
be a polynomial over a field F. Suppose that the coefficient of the monomial  in
in 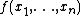 is nonzero and
is nonzero and  is the total degree of
is the total degree of 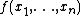 . If
. If  are finite subsets of F with
are finite subsets of F with  for
for  , then there are
, then there are  such that
such that  .
.
The method using the combinatorial Nullstellensatz is also called the polynomial method. This tool was rooted in a paper of N. Alon and M. Tarsi in 1989,[12]
and developed by Alon, Nathanson and Ruzsa in 1995-1996,[13]
and reformulated by Alon in 1999.[11]
References
2. ^Geroldinger & Ruzsa (2009) pp.141–142
3. ^Nathanson (1996) p.48
4. ^Geroldinger & Ruzsa (2009) p.53
5. ^Wolfram's MathWorld, Cauchy-Davenport Theorem, http://mathworld.wolfram.com/Cauchy-DavenportTheorem.html, accessed 20 June 2012.
6. ^Geroldinger & Ruzsa (2009) p.143
7. ^Nathanson (1996) p.77
8. ^{{cite journal | author = Dias da Silva, J. A.; Hamidoune, Y. O. | title = Cyclic spaces for Grassmann derivatives and additive theory | journal = Bulletin of the London Mathematical Society | volume = 26 | year = 1994 | pages = 140–146 | doi = 10.1112/blms/26.2.140 | issue = 2}}
9. ^{{cite journal | author = Hou, Qing-Hu; Sun, Zhi-Wei | title = Restricted sums in a field | journal = Acta Arithmetica | volume = 102 | year = 2002 | issue = 3 | pages = 239–249 | mr = 1884717 | doi = 10.4064/aa102-3-3| bibcode = 2002AcAri.102..239H }}
10. ^{{cite journal | author = Károlyi, Gyula | title = The Erdős–Heilbronn problem in abelian groups | journal = Israel Journal of Mathematics | volume = 139 | year = 2004 | pages = 349–359 | mr = 2041798 | doi = 10.1007/BF02787556}}
11. ^1 {{cite journal | author = Alon, Noga | url = http://www.math.tau.ac.il/~nogaa/PDFS/null2.pdf | title = Combinatorial Nullstellensatz | journal = Combinatorics, Probability and Computing | volume = 8 | issue = 1–2 | year = 1999 | pages = 7–29 | mr = 1684621 | doi = 10.1017/S0963548398003411}}
12. ^{{cite journal | author = Alon, Noga; Tarsi, Michael | title = A nowhere-zero point in linear mappings | journal = Combinatorica | volume = 9 | year = 1989 | pages = 393–395 | mr = 1054015 | doi = 10.1007/BF02125351 | issue = 4}}
13. ^1 {{cite journal | author = Alon, Noga; Nathanson, Melvyn B.; Ruzsa, Imre | url = http://www.math.tau.ac.il/~nogaa/PDFS/anrf3.pdf | title = The polynomial method and restricted sums of congruence classes | journal = Journal of Number Theory | volume = 56 | issue = 2 | year = 1996 | pages = 404–417 | mr = 1373563 | doi = 10.1006/jnth.1996.0029}}
- {{cite book | editor1-last=Geroldinger | editor1-first=Alfred | editor2-last=Ruzsa | editor2-first=Imre Z. | others=Elsholtz, C.; Freiman, G.; Hamidoune, Y. O.; Hegyvári, N.; Károlyi, G.; Nathanson, M.; Solymosi, J.; Stanchescu, Y. With a foreword by Javier Cilleruelo, Marc Noy and Oriol Serra (Coordinators of the DocCourse) | title=Combinatorial number theory and additive group theory | series=Advanced Courses in Mathematics CRM Barcelona | location=Basel | publisher=Birkhäuser | year=2009 | isbn=978-3-7643-8961-1 | zbl=1177.11005 }}
- {{cite book | first=Melvyn B. | last=Nathanson | title=Additive Number Theory: Inverse Problems and the Geometry of Sumsets | volume=165 | series=Graduate Texts in Mathematics | publisher=Springer-Verlag | year=1996 | isbn=0-387-94655-1 | zbl=0859.11003 }}
External links
- {{cite journal | author = Sun, Zhi-Wei | title = An additive theorem and restricted sumsets | year = 2006 | pages = 1263–1276 | volume = 15 | issue = 6 | journal = Math. Res. Lett. , no. | arxiv = math.CO/0610981 }}
- Zhi-Wei Sun: On some conjectures of Erdős-Heilbronn, Lev and Snevily (PDF), a survey talk.
- {{mathworld | urlname = Erdos-HeilbronnConjecture | title = Erdős-Heilbronn Conjecture}}
- Yantaromyrmex
- Yantaromyrmex constricta
- Yantaromyrmex constrictus
- Yantaromyrmex geinitzi
- Yantaromyrmex intermedius
- Yantaromyrmex mayrianum
- Yantaromyrmex samlandica
- Yantaromyrmex samlandicus
- Yantar Seversk
- Yan Tatsine
- Yantau mine
- Yanta (village)
- Yantecaw River
- Yan Temple
- Yantian International Container Terminals
- Yantian Line, Shenzhen Metro
- Yanticaw
- Yanticaw Park
- Yantidzhe
- Yantie lun
- Yantis High School
- Yantlet Creek
- Yantley
- Yantley, Alabama
- Yantokah