“Skew-Hamiltonian matrix”的意思、由来-开放百科全书

词条

Skew-Hamiltonian matrix

释义

Notes

In linear algebra, skew-Hamiltonian matrices are special matrices which correspond to skew-symmetric bilinear forms on a symplectic vector space.

Let V be a vector space, equipped with a symplectic form . Such a space must be even-dimensional. A linear map is called a skew-Hamiltonian operator with respect to if the form is skew-symmetric.

Choose a basis 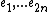in V, such that is written as . Then a linear operator is skew-Hamiltonian with respect to if and only if its matrix A satisfies , where J is the skew-symmetric matrix

and I_n is the identity matrix.^[1] Such matrices are called skew-Hamiltonian.

The square of a Hamiltonian matrix is skew-Hamiltonian. The converse is also true: every skew-Hamiltonian matrix can be obtained as the square of a Hamiltonian matrix.^[1]^[2]

Notes

1. ^¹William C. Waterhouse, The structure of alternating-Hamiltonian matrices, Linear Algebra and its Applications, Volume 396, 1 February 2005, Pages 385-390
2. ^ Heike Fassbender, D. Steven Mackey, Niloufer Mackey and Hongguo XuHamiltonian Square Roots of Skew-Hamiltonian Matrices,Linear Algebra and its Applications 287, pp. 125 - 159, 1999

2 : Matrices|Linear algebra

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。