“Slingshot argument”的意思、由来-开放百科全书

词条

Slingshot argument

释义

The argument
Responses to the argument
See also
References
External links

In philosophical logic, a slingshot argument is one of a group of arguments claiming to show that all true sentences stand for the same thing.

This type of argument was dubbed the "slingshot" by philosophers Jon Barwise and John Perry (1981) due to its disarming simplicity. It is usually said that versions of the slingshot argument have been given by Gottlob Frege, Alonzo Church, W. V. Quine, and Donald Davidson. However, it has been disputed by Lorenz Krüger (1995) that there is much unity in this tradition. Moreover, Krüger rejects Davidson's claim that the argument can refute the correspondence theory of truth. Stephen Neale (1995) claims, controversially, that the most compelling version was suggested by Kurt Gödel (1944).

These arguments are sometimes modified to support the alternative, and evidently stronger, conclusion that there is only one fact, or one true proposition, state of affairs, truth condition, truthmaker, and so on.

The argument

One version of the argument (Perry 1996) proceeds as follows.

Assumptions:

Substitution. If two terms designate the same thing, then substituting one for another in a sentence does not change the designation of that sentence.
Redistribution. Rearranging the parts of a sentence does not change the designation of that sentence, provided the truth conditions of the sentence do not change.
Every sentence is equivalent to a sentence of the form F(a). In other words, every sentence has the same designation as some sentence that attributes a property to something. (For example, "All men are mortal" is equivalent to "The number 1 has the property of being such that all men are mortal".)
For any two objects there is a relation that holds uniquely between them. (For example, if the objects in question are denoted by "a" and "b", the relation in question might be R(x, y), which is stipulated to hold just in case x = a and y = b.)

Let S and T be arbitrary true sentences, designating Des(S) and Des(T), respectively. (No assumptions are made about what kinds of things Des(S) and Des(T) are.) It is now shown by a series of designation-preserving transformations that Des(S) = Des(T). Here, "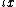" can be read as "the x such that".

1.
2.		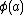assumption 3
3.		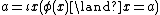redistribution
4.		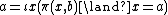substitution, assumption 4
5.		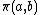redistribution
6.		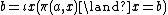redistribution
7.		substitution, assumption 3
8.		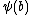redistribution
9.		assumption 3

Note that (1)-(9) is not a derivation of T from S. Rather, it is a series of (allegedly) designation-preserving transformation steps.

Responses to the argument

As Gödel (1944) observed, the slingshot argument does not go through if Bertrand Russell's famous account of definite descriptions is assumed. Russell claimed that the proper logical interpretation of a sentence of the form "The F is G" is:

Exactly one thing is F, and that thing is also G.

Or, in the language of first-order logic:

When the sentences above containing -expressions are expanded out to their proper form, the steps involving substitution are seen to be illegitimate. Consider, for example, the move from (3) to (4). On Russell's account, (3) and (4) are shorthand for:

3'.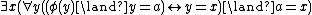
4'.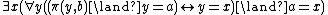

Clearly the substitution principle and assumption 4 do not license the move from (3') to (4'). Thus, one way to look at the slingshot is as simply another argument in favor of Russell's theory of definite descriptions.

If one is not willing to accept Russell's theory, then it seems wise to challenge either substitution or redistribution, which seem to be the other weakest points in the argument. Perry (1996), for example, rejects both of these principles, proposing to replace them with certain weaker, qualified versions that do not allow the slingshot argument to go through.

Gaetano Licata (2011) rejected the slingshot argument showing that the concept of identity (=) employed in the Davidson's and Gödel's demonstration is very problematic, because Gödel (following Russell) uses the G. W. Leibniz's principle of the identity of indiscernibles,{{page needed|date=March 2018}} which suffer from the criticism proposed by Ludwig Wittgenstein: to state that x=y when all properties of x are also properties of y is false because y and x are different signs, while to state that x=x when all properties of x are also properties of x is a nonsense.{{page needed|date=April 2018}} Licata's thesis is that the sign = (usually employed between numbers) needs a logical foundation before being employed between objects and properties.

References

Barwise, Jon, and Perry, John (1981), "Semantic innocence and uncompromising situations", Midwest Studies in the Philosophy of Language, VI.
Gödel, Kurt (1944), "Russell's mathematical logic", in Schilpp (ed.), The Philosophy of Bertrand Russell, Evanston and Chicago: Northwestern University Press, pp. 125–53.
Krüger, Lorenz (1995), "Has the correspondence theory of truth been refuted?", European Journal of Philosophy, vol. 3, 157-173, repr. in Krüger, Why Does History Matter to Philosophy and the Sciences?, ed. by Thomas Sturm, Wolfgang Carl, and Lorraine Daston. Berlin: De Gruyter, 2005, pp. 201-217.
Licata, Gaetano (2011), Truth and Facts: Rejection of the Slingshot Argument in Defence of the Correspondence Theory of Truth, Rome, Aracne.
Neale, Stephen (1995), "The philosophical significance of Gödel's Slingshot", Mind, vol. 104, no. 416, pp. 761–825.
Peirce, C.S. (1906), "Prolegomena to an Apology for Pragmaticism", The Monist, 16, 492–546 (1906). Reprinted, Collected Papers, CP 4.530–572. [https://web.archive.org/web/20070905164710/http://www.existentialgraphs.com/peirceoneg/prolegomena.htm Eprint].
Perry, John (1996), "Evading the slingshot", in Andy Clark et al. (eds.), Philosophy and Cognitive Science. [https://web.archive.org/web/20060902060442/http://www-csli.stanford.edu/~john/PHILPAPERS/evading.pdf PDF].

External links

Stephen Neale's Facing Facts reviewed by John Macfarlane
An Analysis of Davidson's Slingshot Argument

3 : Philosophy of language|Philosophical arguments|Philosophical logic

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。

The argument

Responses to the argument

See also

References

External links