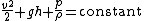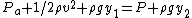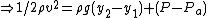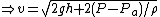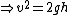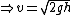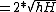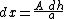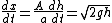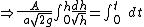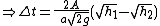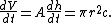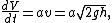- Derivation
- Experimental evidence
- Horizontal distance covered by the jet of liquid
- Total time to empty the container
- Clepsydra problem
- See also
- References
- Further reading
Torricelli's law, also known as Torricelli's theorem, is a theorem in fluid dynamics relating the speed of fluid flowing out of an orifice to the height of fluid above the opening. The law states that the speed of efflux, v, of a fluid through a sharp-edged hole at the bottom of a tank filled to a depth h is the same as the speed that a body (in this case a drop of water) would acquire in falling freely from a height h, i.e.  , where g is the acceleration due to gravity (9.81 N/kg near the surface of the earth). This last expression comes from equating the kinetic energy gained,
, where g is the acceleration due to gravity (9.81 N/kg near the surface of the earth). This last expression comes from equating the kinetic energy gained, 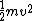 , with the potential energy lost, mgh , and solving for v. The law was discovered (though not in this form) by the Italian scientist Evangelista Torricelli, in 1643. It was later shown to be a particular case of Bernoulli's principle.
, with the potential energy lost, mgh , and solving for v. The law was discovered (though not in this form) by the Italian scientist Evangelista Torricelli, in 1643. It was later shown to be a particular case of Bernoulli's principle.
Derivation
Under the assumptions of an incompressible fluid with negligible viscosity, Bernoulli's principle states that:
where v is fluid speed, g is the gravitational acceleration (9.81 m/s2), h is the fluid's height above a reference point, p is pressure, and ρ is density. Define the opening to be at h=0. At the top of the tank, p is equal to the atmospheric pressure. v can be considered 0 because the fluid surface drops in height extremely slowly compared to the speed at which fluid exits the tank. At the opening, h=0 and p is again atmospheric pressure. Eliminating the constant and solving gives:
Then,
Special cases
I) Small orifice (Area of hole negligible as compared to area of the tank):
Experimental evidence
Torricelli's law can be demonstrated in the spouting can experiment, which is designed to show that in a liquid with an open surface, pressure increases with depth. It consists of a tube with three separate holes and an open surface. The three holes are blocked, then the tube is filled with water. When it is full, the holes are unblocked. The lower a jet is on the tube, the more powerful it is. The fluid's exit velocity is greater further down the tube.[1]
Ignoring viscosity and other losses, if the nozzles point vertically upward then each jet will reach the height of the surface of the liquid in the container.
Horizontal distance covered by the jet of liquid
If h is the depth of the spout and H+h is height of the liquid column, the horizontal distance covered by the jet of liquid to reach the same level as the base of the liquid column can be easily derived:
Velocity of the jet * time taken by the jet to fall H units.
Note that:
1. This distance is independent of acceleration due to gravity, density of the liquid, and the atmospheric pressure.
2. Since interchanging h and H results in the same quantity, two spouts at depths h and H will shoot out the same horizontal distance.
3. At mid way point in the column of liquid, i.e. if h = H, the horizontal distance covered by the jet is maximum.
Total time to empty the container
Consider a cylindrical container containing water to height h is being emptied through a tube freely. Let h be the height of water at any time. Let the velocity of efflux be 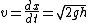
Due to conservation of mass (assuming incompressible flow), 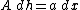 where A and a are the cross sections of container and tube respectively, dh is the height of liquid in container corresponding to dx in the tube which decreases in same time dt:
where A and a are the cross sections of container and tube respectively, dh is the height of liquid in container corresponding to dx in the tube which decreases in same time dt:
is the time required to empty the water from height h1 to h2 in the container, where h1 > h2. This formula can be used to calibrate a water clock.
Clepsydra problem
A clepsydra is a clock that measures time by the flow of water. It consists of a pot with a small hole at the bottom through which the water can escape. The amount of escaping water gives the measure of time. As given by the Torricelli's law, the rate of efflux through the hole depends on the height of the water; and as the water level diminishes, the discharge is not uniform. A simple solution is to keep the height of the water constant. This can be attained by letting a constant stream of water flow into the vessel, the overflow of which is allowed to escape from the top, from another hole. Thus having a constant height, the discharging water from the bottom can be collected in another cylindrical vessel with uniform graduation to measure time. This is an inflow clepsydra.
Alternatively, by carefully selecting the shape of the vessel, the water level in the vessel can be made to decrease at constant rate. By measuring the level of water remaining in the vessel, the time can be measured. This is an example of outflow clepsydra. Since the water outflow rate is higher when the water level is higher (due to more pressure), the fluid's volume should be more than a simple cylinder when the water level is high. That is, the radius should be larger when the water level is higher. Let the radius  increase with the height of the water level
increase with the height of the water level  above the exit hole of area
above the exit hole of area  That is,
That is,  . We want to find the radius that has a constant rate of decrease, i.e.
. We want to find the radius that has a constant rate of decrease, i.e. 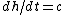 .
.
At a given water level  , the water surface area is
, the water surface area is 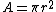 . The instantaneous rate of change in water volume is
. The instantaneous rate of change in water volume is
From Torricelli's law, the rate of outflow is
From these two equations,
Thus, the radius of the container should change in proportion to the quartic root of its height, 
See also
{{commons category|Torricelli's Law}}- Darcy's law
- Dynamic pressure
- Fluid statics
- Hagen–Poiseuille equation
- Helmholtz's theorems
- Kirchhoff equations
- Knudsen equation
- Manning equation
- Mild-slope equation
- Morison equation
- Navier–Stokes equations
- Oseen flow
- Pascal's law
- Poiseuille's law
- Potential flow
- Pressure
- Static pressure
- Pressure head
- Relativistic Euler equations
- Reynolds decomposition
- Stokes flow
- Stokes stream function
- Stream function
- Streamlines, streaklines and pathlines
References
Further reading
- {{cite book | last = T. E. Faber | year = 1995 | title = Fluid Dynamics for Physicists | publisher = Cambridge University Press | isbn = 978-0-521-42969-6}}
- Stanley Middleman, An Introduction to Fluid Dynamics: Principles of Analysis and Design (John Wiley & Sons, 1997) {{ISBN|978-0-471-18209-2}}
- Dennis G. Zill, A First Course in Differential Equations (2005)