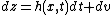“Zakai equation”的意思、由来-开放百科全书

词条

Zakai equation

释义

Overview
See also
References

In filtering theory the Zakai equation is a linear stochastic partial differential equation for the un-normalized density of a hidden state. In contrast, the Kushner equation gives a non-linear stochastic partial differential equation for the normalized density of the hidden state. In principle either approach allows one to estimate a quantity function (the state of a Dynamical system) from noisy measurements, even when the system is non-linear (thus generalizing the earlier results of Wiener and Kalman for linear systems and solving a central problem in estimation theory). The application of this approach to a specific engineering situation may be problematic however, as these equations are quite complex. The Zakai equation is a bilinear stochastic partial differential equation. It was named after Moshe Zakai.

Overview

Assume the state of the system evolves according to

and a noisy measurement of the system state is available:

where 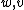are independent Wiener processes. Then the unnormalized conditional probability density of the state at time t is given by the Zakai equation:

where the operator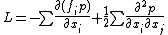

As previously mentioned p is an unnormalized density, i.e. it does not necessarily integrate to 1. After solving for p integration and normalization can be done if desired (an extra step not required in the Kushner approach).

Note that if the last two terms on the right hand side are omitted (by choosing h identically zero), the result is a nonstochastic PDE : the familiar Kolmogorov Forward Equation, which describes the evolution of the state when no measurement information is available.

References

{{cite journal |last1=Zakai |first1=M. |year=1969 |title=On the optimal filtering of diffusion processes |journal=Zeitschrift für Wahrscheinlichkeitstheorie und Verwandte Gebiete |volume=11 |issue=3 |pages=230 |mr=242552 |zbl=0164.19201 |doi=10.1007/BF00536382}}
{{cite book |last=Sritharan |first=S. S. |year=1994 |chapter=Nonlinear filtering of stochastic Navier–Stokes equations |url=http://www.nps.edu/Academics/Schools/GSEAS/SRI/BookCH9.pdf |editor1-last=Funaki |editor1-first=T. |editor2-last=Woyczynski |editor2-first=W. A. |title=Nonlinear Stochastic PDEs: Burgers Turbulence and Hydrodynamic Limit |pages=247–260 |publisher=Springer-Verlag |isbn=0-387-94624-1}}
{{cite book |last=Hobbs |first=S. L. |last2=Sritharan |first2=S. S. |year=1996 |chapter=Nonlinear filtering theory for stochastic reaction–diffusion equations |url=http://www.nps.edu/Academics/Schools/GSEAS/SRI/BookCH10.pdf |editor1-last=Gretsky |editor1-first=N. |editor2-last=Goldstein |editor2-first=J. |editor3-last=Uhl |editor3-first=J. J. |title=Probability and Modern Analysis |pages=219–234 |publisher=Marcel Dekker |isbn= }}

2 : Signal estimation|Stochastic differential equations

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。

Overview

See also

References