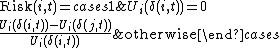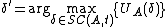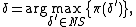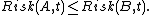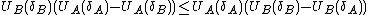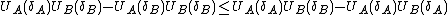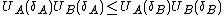“Zeuthen strategy”的意思、由来-开放百科全书

词条

Zeuthen strategy

释义

Risk
Sufficient concession
Minimal sufficient concession
References

{{Multiple issues|{{one source|date=November 2009}}{{context|date=October 2009}}
}}

The Zeuthen strategy is a negotiation strategy used by some artificial agents. Its purpose is to measure the willingness to risk conflict. An agent will be more willing to risk conflict if the difference in utility between its current proposal and the conflict deal is low.

When used by both agents in the Monotonic Concession Protocol, the Zeuthen strategy leads the agents to agree upon the deal in the negotiation set, the set of all conflict free deals which are individually rational and Pareto optimal plus the conflict deal, which maximizes the Nash product.

The strategy was introduced in 1930 by the Danish economist Frederik Zeuthen.^[1]

Risk

Risk(A,t) is a measurement of agent A's willingness to risk conflict. The risk function formalizes the notion that an agent's willingness to risk conflict is the ratio of the utility that agent would lose by accepting the other agent's proposal to the utility that agent would lose by causing a conflict. Agent A is said to be using a rational negotiation strategy if at any step t + 1 that agent A sticks to his last proposal, Risk(A,t) > Risk(B,t).

Sufficient concession

If agent A makes a sufficient concession in the next step, then, assuming that agent B is using a rational negotiation strategy, if agent B does not concede in the next step, he must do so in the step after that. The set of all sufficient concessions of agent A at step t is denoted SC(A, t).

Minimal sufficient concession

is the minimal sufficient concession of agent A in step t.

Agent A begins the negotiation by proposing

and will make the minimal sufficient concession in step t+1 if and only if Risk(A,t) ≤ Risk(B,t).

Theorem

If both agents are using Zeuthen strategies, then they will agree on

that is, the deal which maximizes the Nash product. (Harsanyi 56)

Proof

Let δ_A = δ(A,t).

Let δ_B = δ(B,t).

According to the Zeuthen strategy, agent A will concede at step if and only if

That is, if and only if

Thus, Agent A will concede if and only if 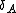does not yield the larger product of utilities.

Therefore, the Zeuthen strategy guarantees a final agreement that maximizes the Nash Product.

References

1. ^{{cite book|author=Fatima, Shaheen|author2=Kraus, Sarit|author3=Wooldridge, Michael|title=Principles of automated negotiation|publisher=Cambridge University Press|year=2014|pages=14–15|url=https://books.google.com/books?id=_j1YCgAAQBAJ&pg=PA14}}

{{cite book

|title= Rules of encounter: designing conventions for automated negotiation among computers Artificial intelligence – The MIT Press series in artificial intelligence
|last1= Rosenschein | first1 = Jeffrey S.
|last2= Zlotkin | first2 = Gilad
|year= 1994
|publisher= MIT Press
|location=
|isbn= 0-262-18159-2
|page=
|pages= 229
|url= https://books.google.com/books?id=4ZhPMk3ftpoC&dq=Zeuthen+Strategy&client=firefox-a&source=gbs_navlinks_s}}

2 : Artificial intelligence|Risk

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。