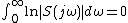- References
- Further reading
- External links
- See also
Bode's sensitivity integral, discovered by Hendrik Wade Bode, is a formula that quantifies some of the limitations in feedback control of linear parameter invariant systems. Let L be the loop transfer function and S be the sensitivity function. Then the following holds:
where 
are the poles of L in the right half plane (unstable poles).
If L has at least two more poles than zeros, and has no poles in the right half plane (is stable), the equation simplifies to:
This equality shows that if sensitivity to disturbance is suppressed at some frequency range, it is necessarily increased at some other range. This has been called the "waterbed effect."[1]
References
1. ^Megretski: The Waterbed Effect. MIT OCW, 2004
Further reading
- Karl Johan Åström and Richard M. Murray. Feedback Systems: An Introduction for Scientists and Engineers. Chapter 11 - Frequency Domain Design. Princeton University Press, 2008. http://www.cds.caltech.edu/~murray/amwiki/Frequency_Domain_Design
- {{Cite journal|last=Stein|first=G.|date=2003|title=Respect the unstable|url=https://ieeexplore.ieee.org/document/1213600/|journal=IEEE Control Systems Magazine|volume=23|issue=4|pages=12–25|doi=10.1109/MCS.2003.1213600|issn=1066-033X}}
- {{Cite journal|last=Costa-Castelló|first=Ramon|last2=Dormido|first2=Sebastián|date=2015|title=An interactive tool to introduce the waterbed effect|url=https://linkinghub.elsevier.com/retrieve/pii/S2405896315025045|journal=IFAC-PapersOnLine|volume=48|issue=29|pages=259–264|doi=10.1016/j.ifacol.2015.11.246|issn=2405-8963}}
External links
- [https://sites.google.com/site/ramoncostacastello/waterbedITOOL WaterbedITOOL] - Interactive software tool to analyze, learn/teach the Waterbed effect in linear control systems.
- [https://www.youtube.com/watch?v=9Lhu31X94V4 Gunter Stein’s Bode Lecture] on fundamental limitations on the achievable sensitivity function expressed by Bode's integral.
See also
- Bode plot
- Sensitivity (control systems)
1 : Control theory
- B & A Trail
- Batram Suri
- Batranesti
- Bat ray
- Batraz
- Bat (Resident Evil)
- Batrochoglanis
- Batroc's Brigade
- Batrope
- Bat-rope
- Batros
- Batroun District
- Batroun, Lebanon
- Batrovci
- Batroxobin
- Batsakis v. Demostis
- Batsakis v. Demotis
- Batsakis v. Demotsis
- Batsbi
- Batsbi language
- Batsch
- Batschia
- BATS Chi-X Europe
- Batschka
- Bats College