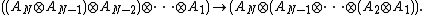“Coherence condition”的意思、由来-开放百科全书

词条

Coherence condition

释义

An illustrative example: a monoidal category
Further examples
Identity Associativity of composition
References

{{Multiple issues|{{Expert-subject|mathematics|date=February 2009}}{{nofootnotes|date=September 2017}}
}}

In mathematics, and particularly category theory a coherence condition is a collection of conditions requiring that various compositions of elementary morphisms are equal. Typically the elementary morphisms are part of the data of the category. A coherence theorem states that, in order to be assured that all these equalities hold, it suffices to check a small number of identities.

An illustrative example: a monoidal category

Part of the data of a monoidal category is a chosen morphism

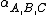, called the associator:

for each triple of objects in the category. Using compositions of these 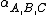, one can construct a morphism

Actually, there are many ways to construct such a morphism as a composition of various 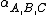. One coherence condition that is typically imposed is that these compositions are all equal.

Typically one proves a coherence condition using a coherence theorem, which states that one only needs to check a few equalities of compositions in order to show that the rest also hold. In the above example, one only needs to check that, for all quadruples of objects , the following diagram commutes.

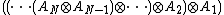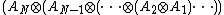Any pair of morphisms from to constructed as compositions of various 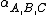are equal.

Further examples

Two simple examples that illustrate the definition are as follows. Both are directly from the definition of a category.

Identity

Let {{nowrap|f : A → B}} be a morphism of a category containing two objects A and B. Associated with these objects are the identity morphisms {{nowrap|1_A : A → A}} and {{nowrap|1_B : B → B}}. By composing these with f, we construct two morphisms:

{{nowrap|f o 1_A : A → B}}, and

{{nowrap|1_B o f : A → B}}.

Both are morphisms between the same objects as f. We have, accordingly, the following coherence statement:

{{nowrap|1= f o 1_A = f = 1_B o f}}.

Associativity of composition

Let {{nowrap|f : A → B}}, {{nowrap|g : B → C}} and {{nowrap|h : C → D}} be morphisms of a category containing objects A, B, C and D. By repeated composition, we can construct a morphism from A to D in two ways:

{{nowrap|(h o g) o f : A → D}}, and

{{nowrap|h o (g o f) : A → D}}.

We have now the following coherence statement:

{{nowrap|1= (h o g) o f = h o (g o f)}}.

In these two particular examples, the coherence statements are theorems for the case of an abstract category, since they follow directly from the axioms; in fact, they are axioms. For the case of a concrete mathematical structure, they can be viewed as conditions, namely as requirements for the mathematical structure under consideration to be a concrete category, requirements that such a structure may meet or fail to meet.

References

Mac Lane, Saunders (1971). Categories for the working mathematician. Graduate texts in mathematics Springer-Verlag. Especially Chapter VII Part 2.

1 : Category theory

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。