“Condensation lemma”的意思、由来-开放百科全书

词条

Condensation lemma

释义

References

In set theory, a branch of mathematics, the condensation lemma is a result about sets in the

constructible universe.

It states that if X is a transitive set and is an elementary submodel of some level of the constructible hierarchy L_α, that is, , then in fact there is some ordinal 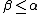such that .

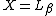More can be said: If X is not transitive, then its transitive collapse is equal to some 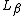, and the hypothesis of elementarity can be weakened to elementarity only for formulas which are in the Lévy hierarchy. Also, the assumption that X be transitive automatically holds when .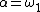

The lemma was formulated and proved by Kurt Gödel in his proof that the axiom of constructibility implies GCH.

References

{{cite book

}} (theorem II.5.2 and lemma II.5.10)

2 : Set theory|Lemmas

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。